Two long straight wires are set parallel to each other Each carries a current i in the same direction and the separation between them is 2r. The intensity of the magnetic field midway between them is
μ0i/r
4μ0i/r
zero
μ0i/4r
The correct Answer is:C
To find the intensity of the magnetic field midway between two long straight parallel wires carrying the same current in the same direction, we can follow these steps:
1. Identify the Setup:
- We have two long straight wires, Wire 1 and Wire 2, carrying a current I in the same direction.
- The distance between the two wires is 2r, which means the distance from each wire to the midpoint is r.
2. Determine the Magnetic Field due to Each Wire:
- The magnetic field B created by a long straight wire at a distance r from it is given by the formula:
B=μ0I2πr
- Here, μ0 is the permeability of free space.
3. Calculate the Magnetic Field at the Midpoint:
- For Wire 1, the magnetic field at the midpoint (due to Wire 1) will be directed into the page (inward).
- For Wire 2, the magnetic field at the midpoint (due to Wire 2) will be directed out of the page (outward).
4. Direction of Magnetic Fields:
- Using the right-hand rule, we can determine the direction of the magnetic fields:
- For Wire 1 (current flowing in the same direction), the magnetic field at the midpoint is inward.
- For Wire 2 (also with current flowing in the same direction), the magnetic field at the midpoint is outward.
5. Magnitude of the Magnetic Fields:
- The magnitudes of the magnetic fields due to both wires at the midpoint are the same:
B1=B2=μ0I2πr
6. Net Magnetic Field Calculation:
- Since the magnetic fields are equal in magnitude but opposite in direction, they will cancel each other out:
Bnet=B1−B2=μ0I2πr−μ0I2πr=0
Conclusion:
The intensity of the magnetic field midway between the two wires is:
Bnet=0
- We have two long straight wires, Wire 1 and Wire 2, carrying a current I in the same direction.
- The distance between the two wires is 2r, which means the distance from each wire to the midpoint is r.
- The magnetic field B created by a long straight wire at a distance r from it is given by the formula:
B=μ0I2πr
- Here, μ0 is the permeability of free space.
- For Wire 1, the magnetic field at the midpoint (due to Wire 1) will be directed into the page (inward).
- For Wire 2, the magnetic field at the midpoint (due to Wire 2) will be directed out of the page (outward).
- Using the right-hand rule, we can determine the direction of the magnetic fields:
- For Wire 1 (current flowing in the same direction), the magnetic field at the midpoint is inward.
- For Wire 2 (also with current flowing in the same direction), the magnetic field at the midpoint is outward.
- The magnitudes of the magnetic fields due to both wires at the midpoint are the same:
B1=B2=μ0I2πr
- Since the magnetic fields are equal in magnitude but opposite in direction, they will cancel each other out:
Bnet=B1−B2=μ0I2πr−μ0I2πr=0
The intensity of the magnetic field midway between the two wires is:
Bnet=0
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Assignment (Section B) Objective Type Questions (One option is correct)
35 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Assignment Section C (Objective Type Questions (More than one option are correct)
14 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Try Yourself
27 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - SECTION D
16 Videos
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
Similar Questions
Two long straight wires are set parallel to each other. Each carriers a current i in the same direction and the separation between them is 2r. The intensity of the magnetic field midway between them is
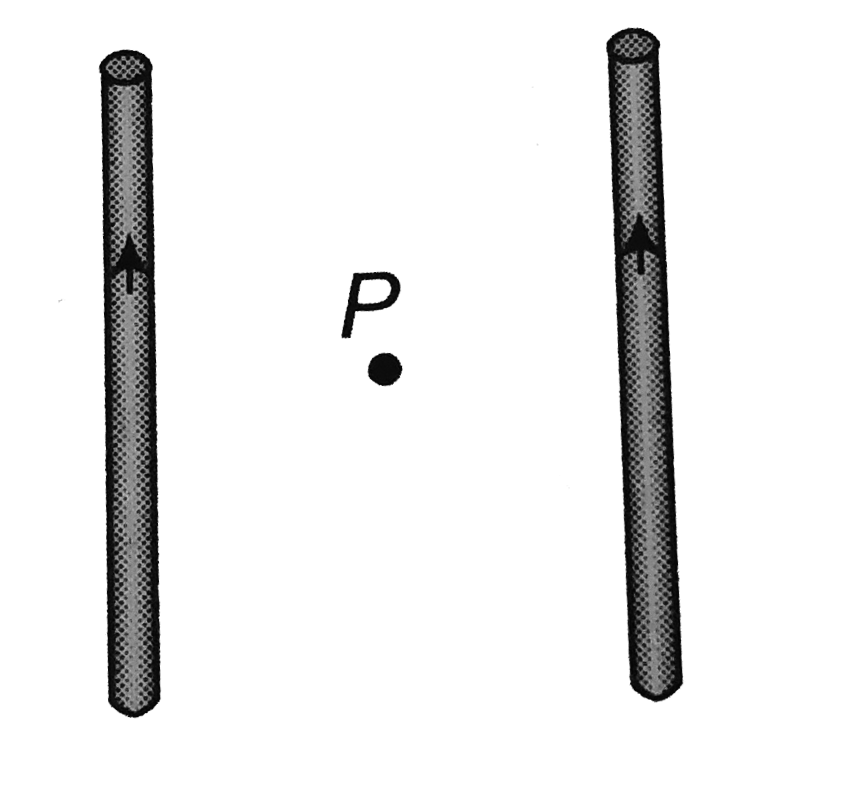
View SolutionThe two long, straight wires carrying electric currents in opposite directions. The separation between the wires is 5.0 cm. Find the magnetic field at a point P midway between the wires.
View Solution
Knowledge Check
The two linear parallel conductors carrying currents in the opposite direction…………………each other.
AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is the correct explanation of assertion.CIf assertion is true but reason is falseDIf both assertion and reason are false.
Two thin, long, parallel wires, separated by a distance 'd' carry a current of 'i' A in the same direction. They will
View SolutionThree coplanar parallel wires, each carrying a current of 10 A along the same direction, are placed with a separation 5.0 cm between the consecutive ones. Find the magnitude of the magnetic force per unit length acting on the wires.
View SolutionTwo long straight wires, each carrying a current I in opposite directions are separated by a distance R. The magnetic induction at a point mid way between the wire is
View SolutionTwo long wires are placed parallel to each other with a distance 1 m between them. The wires carry equal current 1 A in the same direction. The magnetic field intensity at a point P which is equidistant from the wires is 1.6×10−7T. Then, the distance of this point from any one of the wires is _________ m.
[Permeability of vacuum, μ0=4π×10−7T−m/A]
View SolutionTwo long straight parallel conductors 10 cm apart, carry currents of 5A each in the same direction. Then the magnetic induction at a point midway between them is
View Solution(a) Two straight long parallel conductors carry currents I1 and I2 in the same direction. Deduce the expression for the firce per unit length between them.
Depict the pattern of magnetic field lines around them.
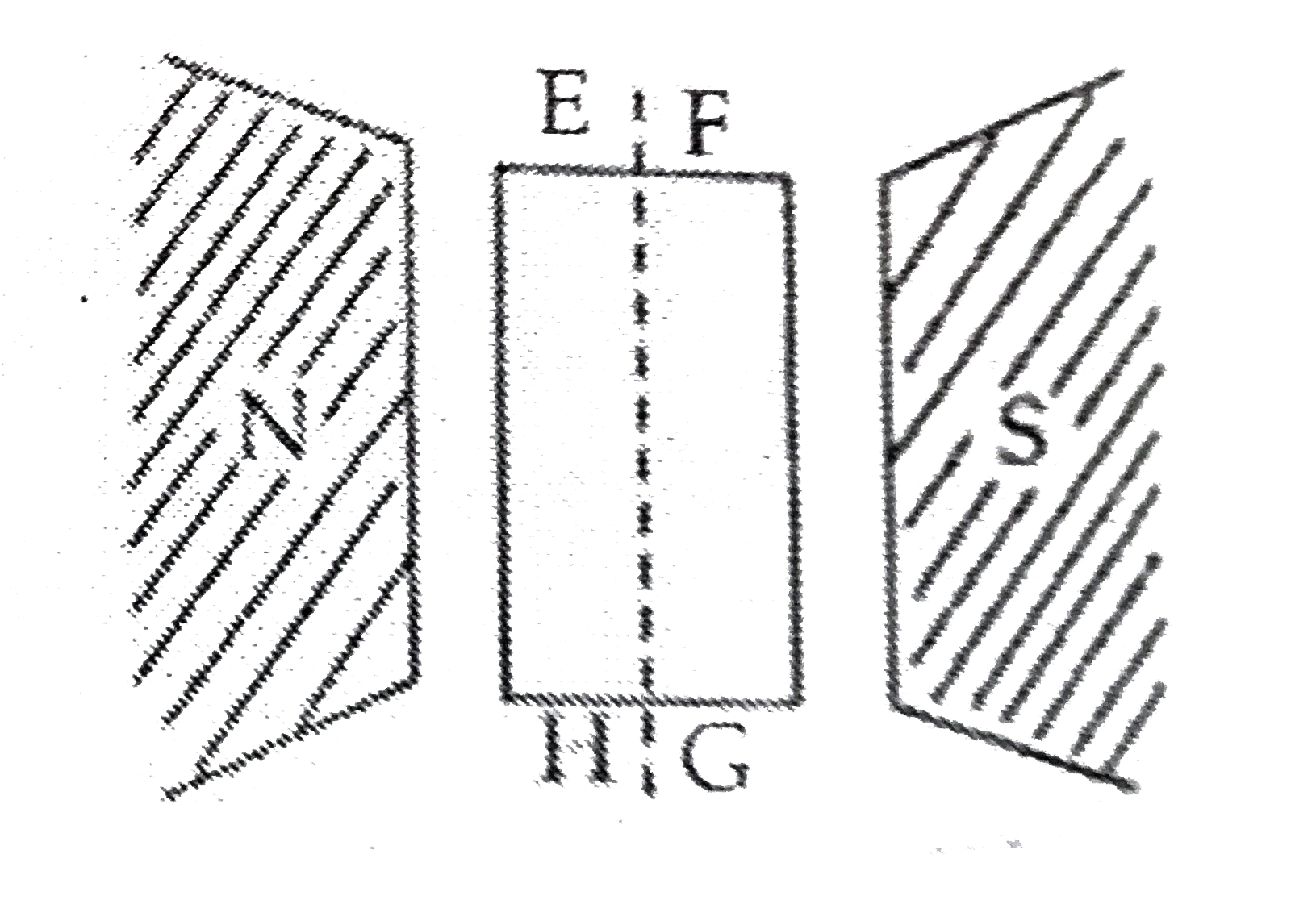
(b) A rectangular current carrying loop EFGH is kept in a uniform magnetic field as shown in the figure.
(i) What is the direction of the magnetic moment of the current loop ?
(ii) What is the torque acting on the loop (A) maximum, (B) zero ?
View SolutionTwo proton beams going in the same direction repel each
other whereas two wires carrying currents in the same
direction attract each other. Explain.
View Solution
