Suppose a small angled prism of 6∘ deviates a ray through 3∘, then the refractive index is
1.62
1.33
1.5
1.72
The correct Answer is:C
To find the refractive index (μ) of a small angled prism that deviates a ray through a certain angle, we can use the formula related to prism deviation. Here’s a step-by-step solution:
Step 1: Identify the given values
- Angle of the prism (A) = 6 degrees
- Angle of deviation (δ) = 3 degrees
Step 2: Use the formula for deviation in a prism
The relationship between the angle of the prism (A), the angle of deviation (δ), and the refractive index (μ) is given by the formula:
δ=A(μ−1)
Step 3: Substitute the known values into the formula
Substituting the values of A and δ into the formula:
3=6(μ−1)
Step 4: Simplify the equation
Now, we will simplify the equation:
3=6μ−6
Step 5: Rearrange the equation to solve for μ
Adding 6 to both sides:
3+6=6μ
9=6μ
Step 6: Divide both sides by 6 to isolate μ
μ=96
Step 7: Simplify the fraction
μ=1.5
Conclusion
The refractive index of the prism is 1.5.
---
- Angle of the prism (A) = 6 degrees
- Angle of deviation (δ) = 3 degrees
The relationship between the angle of the prism (A), the angle of deviation (δ), and the refractive index (μ) is given by the formula:
δ=A(μ−1)
Substituting the values of A and δ into the formula:
3=6(μ−1)
Now, we will simplify the equation:
3=6μ−6
Adding 6 to both sides:
3+6=6μ
9=6μ
μ=96
μ=1.5
The refractive index of the prism is 1.5.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Assignment (Section - B) Objective Type Questions (One option is correct)
15 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Assignment (Section - C) Objective Type Questions (More than one option are correct)
9 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSIGNMENT (SECTION - D)
16 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
4 Videos
- SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )EXERCISE - Assignment SECTION - D (Assertion & reason type Question)
10 Videos
Similar Questions
For a small angled prism, angle of minimum deviation (δ) varies with the refractive index of the prism as shown in the graph
View SolutionA light ray, going through a prism with the angle of prism 60∘, is found to deviate by 30∘. What limit on the refractive index can be put from these data ?
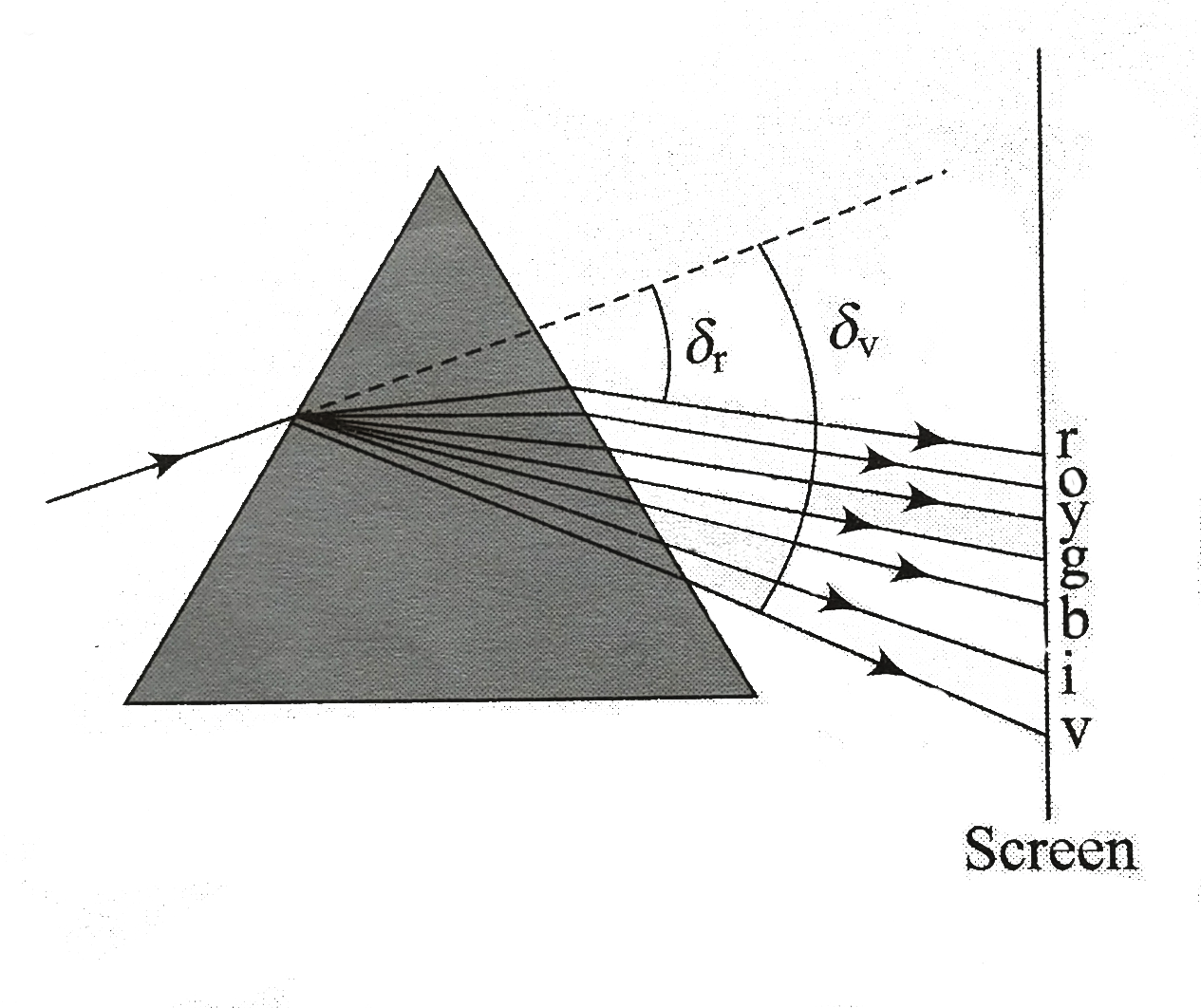
View SolutionA thin prism of angle A=6∘ produces a deviation d=3∘. Find the refractive index of the material of prism.
View SolutionIf the angle of minimum deviation is of 60∘ for an equilateral prism , then the refractive index of the material of the prism is
View SolutionA light ray going through a prism with the angle of prism 60∘, is founded to deviate at least by 30∘. what Is the range of the refractive index of the prism?
View SolutionThe angle of minimum deviation measured with a prism is 30∘ and the angle of prism is 60∘. The refractive index of prism material is
View SolutionA prism is made of glass of unknown refractive index. A parallel beam of light is incident on a face of the prism. By rotating the prism, the minimum angle of deviation is measured to be 40∘. What is the refractive index of the prism ? If the prism is placed in water (μ=1.33), predict the new angle of minimum deviation of the parallel beam. The refracting angle of prism is 60∘.
View SolutionThe angle of incidance for an equilateral prism is 60∘. What should be the refractive index of prism so that the ray is parallel to the base inside the prism ?
View SolutionA Ray of light is incident at an angle 60° on one face of a prism which has protecting angle of 30°. The emerging ray deviates through 30° from incident light. The refractive index of material of prism is
View SolutionFor a ray of light refracted through a prism of angle 60∘, the angle of incidence is equal to the angle of emergence, each equal to 45∘. Find the refractive index of the material of the prism.
View Solution
