In which transition of a hydrogen atom, photons of lowest frequency are emitted ?
n=4 to n=3
n=4 to n=2
n=2 to n=1
n=3 to n=1
The correct Answer is:A
To determine the transition in a hydrogen atom that emits photons of the lowest frequency, we can follow these steps:
Step 1: Understand the relationship between energy and frequency
The energy of a photon is given by the equation:
E=hν
where E is the energy, h is Planck's constant, and ν (nu) is the frequency of the photon. From this equation, we can see that energy is directly proportional to frequency. Therefore, to find the transition that emits the lowest frequency, we need to find the transition that corresponds to the lowest energy difference between two energy levels.
Step 2: Identify the energy levels in a hydrogen atom
In a hydrogen atom, the energy levels are quantized and can be expressed using the formula:
En=−13.6eVn2
where n is the principal quantum number (n = 1, 2, 3, ...). As n increases, the energy levels get closer together, meaning the difference in energy between successive levels decreases.
Step 3: Determine the transitions and their energy differences
To find the transition with the lowest energy difference, we can consider the transitions between higher energy levels. The energy difference ΔE between two levels ni and nf is given by:
ΔE=Enf−Eni=−13.6n2f+13.6n2i
Step 4: Calculate the energy differences for possible transitions
Let's consider the transitions between higher energy levels:
1. Transition from n=4 to n=3
2. Transition from n=3 to n=2
3. Transition from n=2 to n=1
Calculating the energy differences:
- For n=4 to n=3:
ΔE4→3=E3−E4=(−13.632)−(−13.642)
=−13.69+13.616
- For n=3 to n=2:
ΔE3→2=E2−E3=(−13.622)−(−13.632)
=−13.64+13.69
- For n=2 to n=1:
ΔE2→1=E1−E2=(−13.612)−(−13.622)
=−13.6+13.64
Step 5: Identify the transition with the minimum energy difference
From the calculations, we can see that the transition from n=4 to n=3 has the smallest energy difference because the energy levels are closer together at higher values of n. Therefore, this transition will emit photons of the lowest frequency.
Conclusion
The transition in a hydrogen atom that emits photons of the lowest frequency is:
Transition from n=4 to n=3.
---
The energy of a photon is given by the equation:
E=hν
where E is the energy, h is Planck's constant, and ν (nu) is the frequency of the photon. From this equation, we can see that energy is directly proportional to frequency. Therefore, to find the transition that emits the lowest frequency, we need to find the transition that corresponds to the lowest energy difference between two energy levels.
In a hydrogen atom, the energy levels are quantized and can be expressed using the formula:
En=−13.6eVn2
where n is the principal quantum number (n = 1, 2, 3, ...). As n increases, the energy levels get closer together, meaning the difference in energy between successive levels decreases.
To find the transition with the lowest energy difference, we can consider the transitions between higher energy levels. The energy difference ΔE between two levels ni and nf is given by:
ΔE=Enf−Eni=−13.6n2f+13.6n2i
Let's consider the transitions between higher energy levels:
1. Transition from n=4 to n=3
2. Transition from n=3 to n=2
3. Transition from n=2 to n=1
- For n=4 to n=3:
ΔE4→3=E3−E4=(−13.632)−(−13.642)
=−13.69+13.616
ΔE3→2=E2−E3=(−13.622)−(−13.632)
=−13.64+13.69
ΔE2→1=E1−E2=(−13.612)−(−13.622)
=−13.6+13.64
From the calculations, we can see that the transition from n=4 to n=3 has the smallest energy difference because the energy levels are closer together at higher values of n. Therefore, this transition will emit photons of the lowest frequency.
The transition in a hydrogen atom that emits photons of the lowest frequency is:
Transition from n=4 to n=3.
Topper's Solved these Questions
- ATOMSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ATOMSEXERCISE - ASSIGNMENT SECTION B Objective (One option is correct )
12 Videos
- ATOMSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ATOMSEXERCISE - ASSIGNMENT SECTION C Objective (More than one option is correct )
6 Videos
- ATOMSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ATOMSEXERCISE - Try Yourself
24 Videos
- ALTERNATING CURRENT BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ALTERNATING CURRENT EXERCISE - Assignment (Section-J) (Aakash Chailengers Questions)
2 Videos
- COMMUNICATION SYSTEMS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - COMMUNICATION SYSTEMS EXERCISE - ASSIGNMENT SECTION D (Assertion-Reason)
10 Videos
Similar Questions
Which of the following transitions in a hydrogen atom emits photon of the highest frequency ?
View SolutionA hydrogen atom is in the third excited state. It make a transition to a different state and a photon is either obsorbed or emitted. Determine the quantum number n of the final state and the energy energy of the photon If it is
a emitted with the shortest possible wavelength.
b emitted with the longest possible wavelength and
c absorbed with the longest possible wavelength.
View Solution
Knowledge Check
Which of the transitions in hydrogen atom emits a photon of lowest frequecny (n= quantum number)?
An=2 to n=1Bn=4 to n=2Cn=4 to n=1Dn=4 to n=3
In Bohr Atomic model regarding transition of electron for hydrogen like atom following observation were noted.
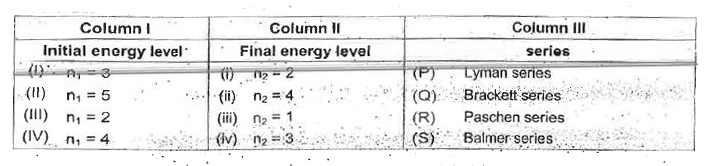
Transition of electron to corresponding to lowest energy released is
View SolutionWhich of the following electron transitions in a hydrogen atom will require the largest amount of energy?
View SolutionWhich electronic transition in a hydrogen atom releases the greatest amount of energy?
View SolutionCalculate the frequency of light amitted in an electron transition from the sixth to the second orbit of a hydrogen atom .In what region of the specturm does this frequency accur?
View SolutionWhich of the following electronic transition in hydrogen atom will emit largest amount of energy?
View SolutionA hydrogen-like atom emits radiation of frequency 2.7×1015 Hz when if makes a transition from n = 2 to n = 1. The frequency emitted in a transition from n = 3 to n = 1 will be
View SolutionWhen a photon is emitted from an atom , the atom recils The kinetic energy of recoils and the energy of the photon come from the difference in energy between the state involved in the transition suppose a hydrogen atom change its state from n=3→n=2 calculate the fractional change in the wavelength of light emitted , due to the recoil
View Solution
