A particle projected at some angle with velocity 50 m/s crosses a 20 m high wall after 4 s from the time of projection. The angle of porjection of the particle is
30∘
45∘
60∘
53∘
The correct Answer is:A
To solve the problem step by step, we will follow the projectile motion equations and the given conditions.
Step 1: Identify the components of the initial velocity
The initial velocity u of the particle is given as 50 m/s. This velocity can be broken down into horizontal and vertical components:
- Horizontal component: ux=ucosθ=50cosθ
- Vertical component: uy=usinθ=50sinθ
Step 2: Use the vertical motion equation
The vertical motion of the particle can be described using the equation:
S=ut+12at2
where:
- S is the vertical displacement (height of the wall = 20 m),
- u is the initial vertical velocity,
- t is the time of flight (4 s),
- a is the acceleration due to gravity (approximately -10 m/s², acting downwards).
Substituting the known values into the equation:
20=(50sinθ)(4)+12(−10)(42)
Step 3: Simplify the equation
Calculating the second term:
12(−10)(16)=−80
Now substituting this back into the equation:
20=200sinθ−80
Step 4: Rearrange the equation
Rearranging gives:
200sinθ=20+80
200sinθ=100
Step 5: Solve for sinθ
Now, divide both sides by 200:
sinθ=100200=12
Step 6: Find the angle θ
The angle θ for which sinθ=12 is:
θ=30∘
Conclusion
The angle of projection of the particle is 30∘.
The initial velocity u of the particle is given as 50 m/s. This velocity can be broken down into horizontal and vertical components:
- Horizontal component: ux=ucosθ=50cosθ
- Vertical component: uy=usinθ=50sinθ
The vertical motion of the particle can be described using the equation:
S=ut+12at2
where:
- S is the vertical displacement (height of the wall = 20 m),
- u is the initial vertical velocity,
- t is the time of flight (4 s),
- a is the acceleration due to gravity (approximately -10 m/s², acting downwards).
20=(50sinθ)(4)+12(−10)(42)
Calculating the second term:
12(−10)(16)=−80
Now substituting this back into the equation:
20=200sinθ−80
Rearranging gives:
200sinθ=20+80
200sinθ=100
Now, divide both sides by 200:
sinθ=100200=12
The angle θ for which sinθ=12 is:
θ=30∘
The angle of projection of the particle is 30∘.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -C Objective (More than one option is correct)
7 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -D (Linked Comprehension)
12 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -A Objective (one option is correct)
50 Videos
- MOCK_TEST_17BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOCK_TEST_17EXERCISE - Example
15 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - D)
15 Videos
Similar Questions
A particle is projected from ground with velocity 40√2m/s at 45∘. At time t=2s
View SolutionA particle projected from ground moves at angle 45∘ with horizontal one second after projection and speed is minimum two seconds after the projection The angle of projection of particle is [ Neglect the effect of air resistance )
View SolutionA body is projected with a velocity of 30m/s to have to horizontal range of 45m. Find the angle of projection .
View SolutionA particle is projected from ground with velocity 3ˆi+4ˆj m/s. Find range of the projectile :-
View SolutionA particle is projected at an angle 60∘ with horizontal with a speed v=20m/s. Taking g=10m/s2. Find the time after which the speed of the particle remains half of its initial speed.
View SolutionA particle A is projected with an initial velocity of 60m/s at an angle 30∘ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of 50m/s from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection α of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. (g=10m/s2)
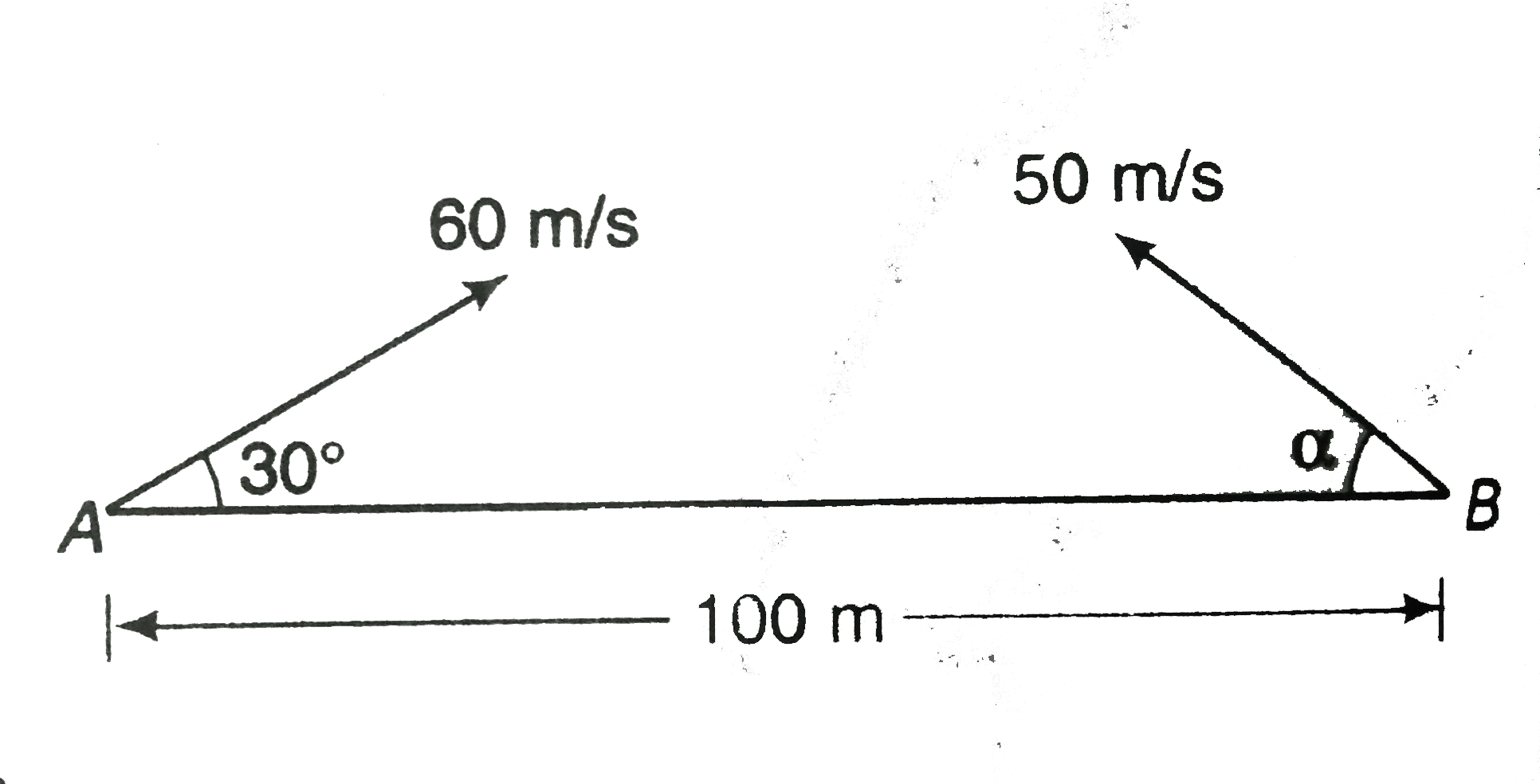
View SolutionA ball is projected horizontally. After 3s from projection its velocity becomes 1.25 times of the velocity of projection. Its velocity of projection is
View SolutionA particle is projected from ground at some angle with horizontal (Assuming point of projection to the origin and the horizontal and vertical directions to be the x and y axis) the particle passes through the points (3, 4) m and (4, 3) m during its motion then the range of the particle would be : (g=10m/s2)
View SolutionA particle is projected vertically upwards from ground with velocity 10 m/s. Find the time taken by it to reach at the highest point ?
View SolutionA particle is projected with velocity v at an angle θ aith horizontal. The average angle velocity of the particle from the point of projection to impact equals
View Solution