six particles are situated at the corners of a regular hexagon. These particles start moving with equal speed of 10 m/s in such that velocity of any one particle is directed towards the next particle. Find the rate (in m/s) at which length of a side of the hexagon is decreasing.
The correct Answer is:5
To solve the problem of determining the rate at which the length of a side of the hexagon is decreasing, we can follow these steps:
Step 1: Understand the Configuration
We have six particles located at the corners of a regular hexagon. Each particle moves towards the next particle with a speed of 10 m/s. Let's label the particles as A, B, C, D, E, and F in a clockwise manner.
Hint: Visualize the hexagon and the direction of motion of each particle towards the next.
Step 2: Analyze the Motion
Each particle moves directly towards the next one. For example, particle A moves towards particle B, particle B moves towards particle C, and so on. The angle between the direction of motion of any particle and the line connecting it to the next particle is 60 degrees (since the internal angles of a regular hexagon are 120 degrees).
Hint: Remember that the angle between the velocity vector of a particle and the line connecting it to the next particle is crucial for resolving the velocities.
Step 3: Resolve the Velocity Components
The velocity of each particle can be resolved into two components: one along the line connecting it to the next particle and one perpendicular to that line.
For particle A moving towards B:
- The component of the velocity of A towards B is vAB=vcos(60∘)=10⋅12=5m/s.
Hint: Use trigonometric functions to resolve the velocities into components along the direction of interest.
Step 4: Determine the Rate of Decrease of the Side Length
Since particle A is moving towards particle B with a velocity of 5 m/s, this means that the distance between particles A and B (which is the length of the side of the hexagon) is decreasing at this rate.
Thus, the rate at which the length of a side of the hexagon is decreasing is 5m/s.
Hint: The rate of decrease in distance between two moving particles can be directly derived from the component of their velocities along the line connecting them.
Final Answer
The rate at which the length of a side of the hexagon is decreasing is 5m/s.
---
We have six particles located at the corners of a regular hexagon. Each particle moves towards the next particle with a speed of 10 m/s. Let's label the particles as A, B, C, D, E, and F in a clockwise manner.
Each particle moves directly towards the next one. For example, particle A moves towards particle B, particle B moves towards particle C, and so on. The angle between the direction of motion of any particle and the line connecting it to the next particle is 60 degrees (since the internal angles of a regular hexagon are 120 degrees).
The velocity of each particle can be resolved into two components: one along the line connecting it to the next particle and one perpendicular to that line.
- The component of the velocity of A towards B is vAB=vcos(60∘)=10⋅12=5m/s.
Since particle A is moving towards particle B with a velocity of 5 m/s, this means that the distance between particles A and B (which is the length of the side of the hexagon) is decreasing at this rate.
The rate at which the length of a side of the hexagon is decreasing is 5m/s.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -H (Multiple True-False)
1 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -I (Subjective )
2 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -F (Matrix-Match)
2 Videos
- MOCK_TEST_17BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOCK_TEST_17EXERCISE - Example
15 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - D)
15 Videos
Similar Questions
Six particles situated at the corners of a regular hexagon of side a move at a constant speed v. Each particle maintains a direction towards the particle at the next corner. Calculate the time the particles will take to meet each other.
View SolutionA particle moves with a uniform velocity of 50 m/s for 20 min. What is the velocity of particle at t = 2 min ?
View Solution
Knowledge Check
A particle moves in the direction of east for 2s with velocity of 15m/s .Then it moves towards north for 8s with a velocity of 5m/s . The average velocity of the particle is (in m/s)
A1 m/sB5 m/sC7 m/sD10 m/s
A particle moves in a straight line with constant speed of 4m/s for 2s, then with 6m/s for 3s. Find the average speed of the particle in the given time interval.
View SolutionA particle is moving along a circle of radius 20cm, with a linear velocity of 2m//s. Calculate the angular velocity of the particle.
View SolutionA particle is moving along a circle of radius 20cm, with a linear velocity of 2m//s. Calculate the angular velocity of the particle.
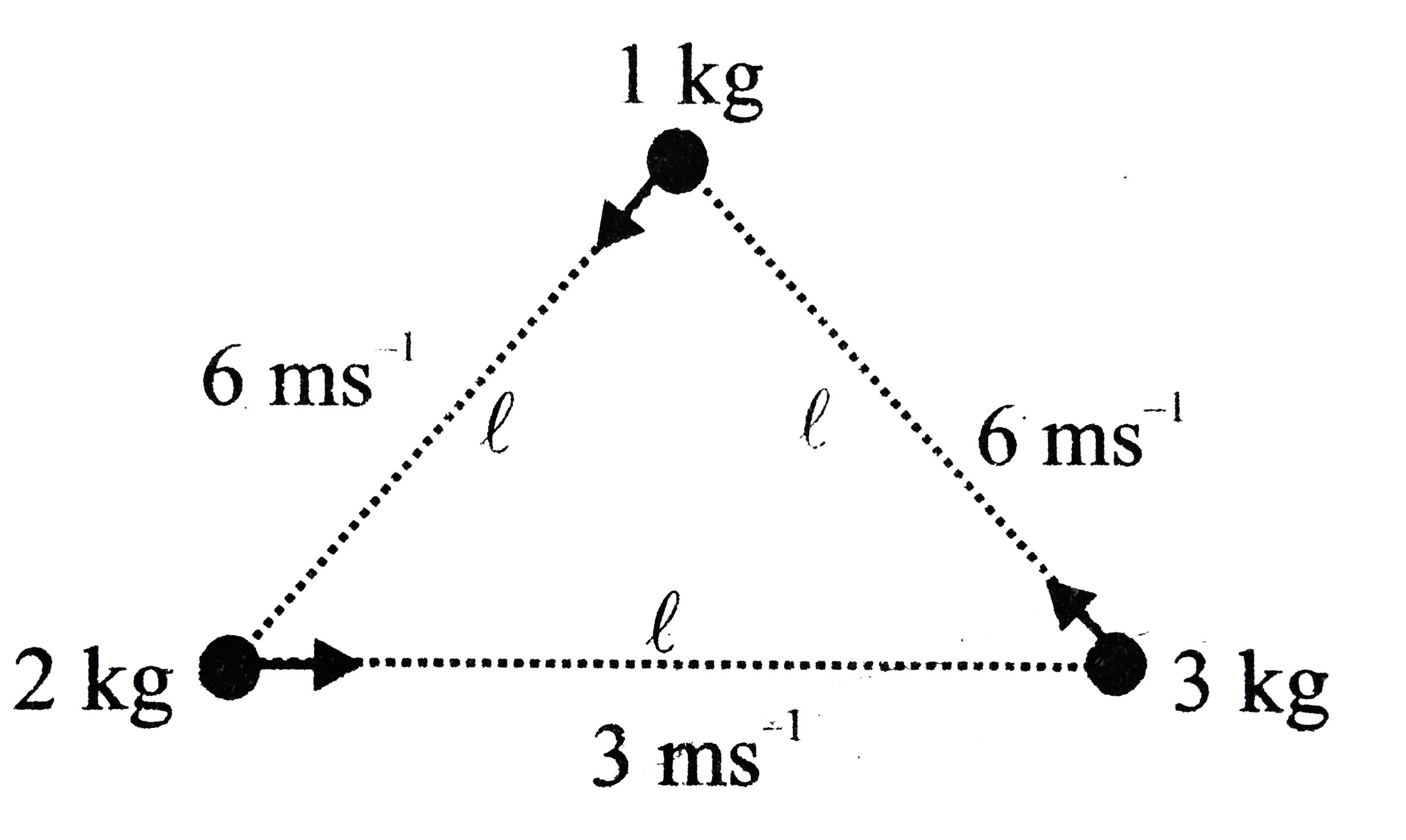
View SolutionThree particles of masses 1kg,2kg and 3kg are situated at the corners of an equilateral triangle move at speed 6ms−1,3ms−1 and 2ms−1 respectively. Each particle maintains a direction towards the particles at the next corners symmetrically. Find velocity of CM of the system at this instant
View SolutionA particle is moing with a velocity of 10m/s towards east. After 10s its velocity changes to 10m/s towards north. Its average acceleration is:-
View SolutionIn a 3 dimensional space, two particles are moving with uniform speeds of 6m/s and 8m/s along two arbitrary curves. The speed of one particle, as observed by other can be :
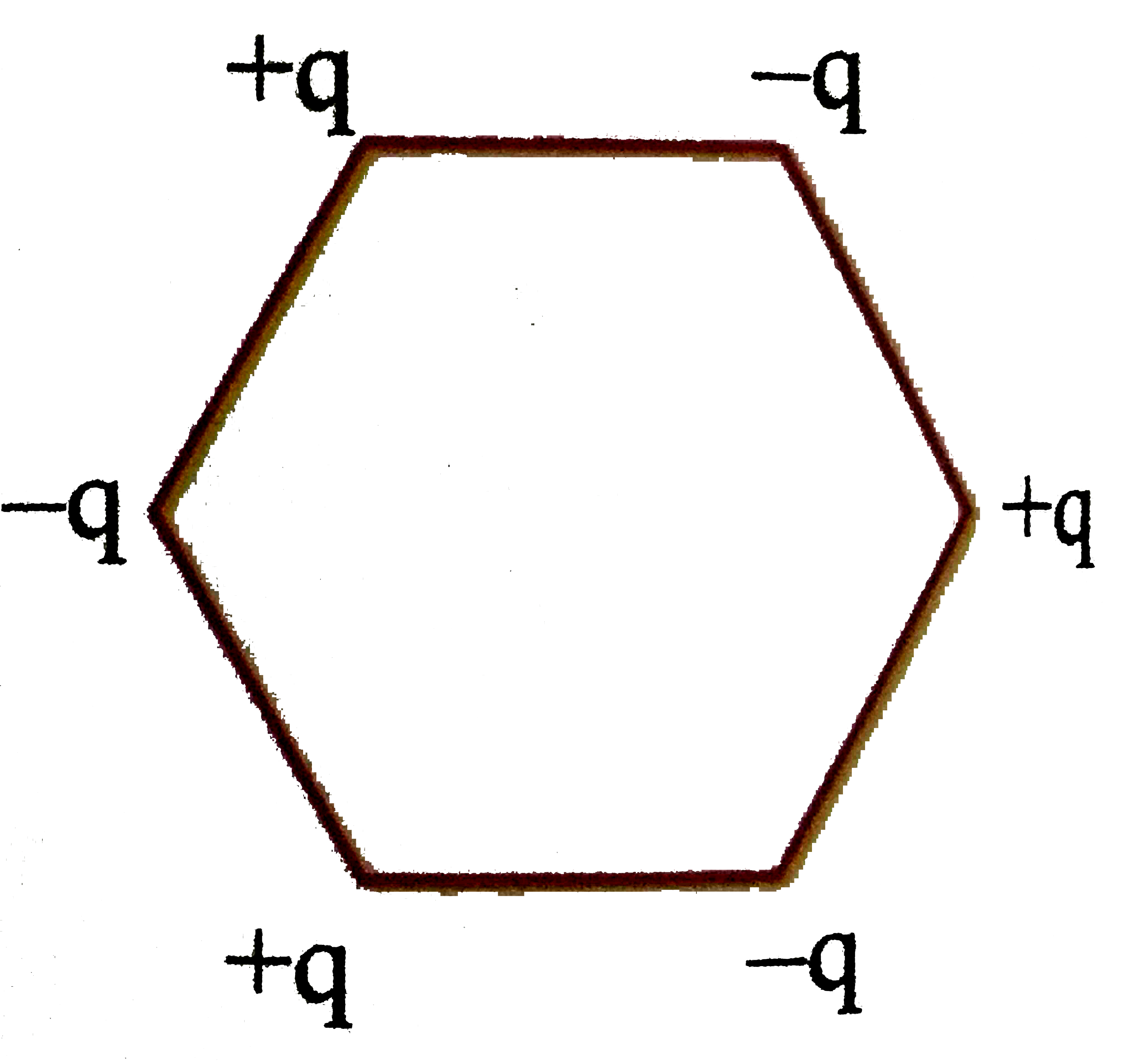
View SolutionSix charge of magnitude +q and −q are fixed at the corners of a regular hexagon of edge length a as shown in the figure .The electrostatic interaction energy of the charged particle is :
View Solution
