What will be the acceleration of the bar magnet, if the loop had a cut in between (or if it was not a closed loop)?
To solve the question regarding the acceleration of a bar magnet when placed near a loop with a cut, we can follow these steps:
1. Understanding the Setup:
- We have a bar magnet and a loop of wire. In the case where the loop is complete, the movement of the magnet induces a current in the loop.
2. Induced Current in a Closed Loop:
- When the bar magnet moves towards the closed loop, it creates a changing magnetic field. According to Faraday's law of electromagnetic induction, this changing magnetic field induces a current in the loop.
- The direction of the induced current can be determined using Lenz's law, which states that the induced current will flow in a direction that opposes the change in magnetic flux.
3. Force on the Magnet:
- The induced current in the loop creates a magnetic field that exerts a force on the bar magnet in the opposite direction of its motion. This opposing force reduces the acceleration of the magnet.
4. Acceleration in the Closed Loop Case:
- Since there is an opposing force due to the induced current, the acceleration of the bar magnet will be less than the acceleration due to gravity (g).
5. Considering the Cut in the Loop:
- Now, if the loop has a cut (making it an open loop), there will be no complete circuit for the current to flow.
- Without a closed loop, there is no induced current when the bar magnet moves.
6. Conclusion for the Open Loop:
- Since there is no induced current, there is no opposing force acting on the bar magnet. Therefore, the only force acting on the magnet is gravity.
7. Final Acceleration of the Bar Magnet:
- In this case, the acceleration of the bar magnet will be equal to the acceleration due to gravity (g).
Final Answer:
The acceleration of the bar magnet, if the loop had a cut in between (or if it was not a closed loop), will be equal to g (acceleration due to gravity).
---
- We have a bar magnet and a loop of wire. In the case where the loop is complete, the movement of the magnet induces a current in the loop.
- When the bar magnet moves towards the closed loop, it creates a changing magnetic field. According to Faraday's law of electromagnetic induction, this changing magnetic field induces a current in the loop.
- The direction of the induced current can be determined using Lenz's law, which states that the induced current will flow in a direction that opposes the change in magnetic flux.
- The induced current in the loop creates a magnetic field that exerts a force on the bar magnet in the opposite direction of its motion. This opposing force reduces the acceleration of the magnet.
- Since there is an opposing force due to the induced current, the acceleration of the bar magnet will be less than the acceleration due to gravity (g).
- Now, if the loop has a cut (making it an open loop), there will be no complete circuit for the current to flow.
- Without a closed loop, there is no induced current when the bar magnet moves.
- Since there is no induced current, there is no opposing force acting on the bar magnet. Therefore, the only force acting on the magnet is gravity.
- In this case, the acceleration of the bar magnet will be equal to the acceleration due to gravity (g).
The acceleration of the bar magnet, if the loop had a cut in between (or if it was not a closed loop), will be equal to g (acceleration due to gravity).
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assignment (SECTION - A)
55 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assignment (SECTION - B)
22 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT(SECTION -D) Assertion-Reason type Question)
15 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - comprehension
3 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions
25 Videos
Similar Questions
Knowledge Check
Statement-1 The net magnetic flux through a spherical surface enclosing north pole of a bar magnet as zero
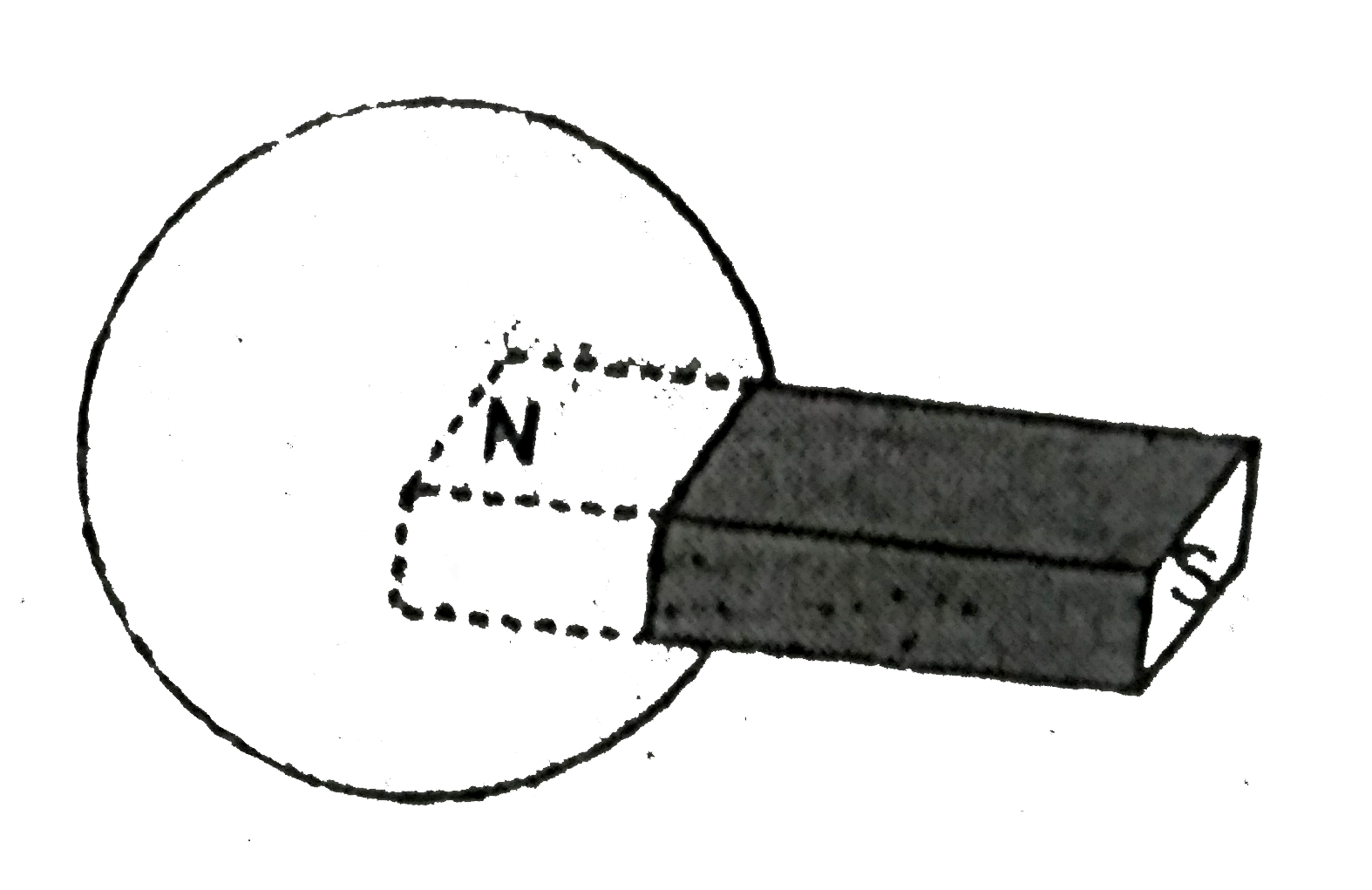
Statement-2 Magnetic field lines always form closed loopsAIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is the correct explanation of assertion.CIf assertion is true but reason is falseDIf both assertion and reason are false.Statement-1 The net magnetic flux through a spherical surface enclosing north pole of a bar magnet as zero

Statement-2 Magnetic field lines always form closed loopsAIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is the correct explanation of assertion.CIf assertion is true but reason is falseDIf both assertion and reason are false.
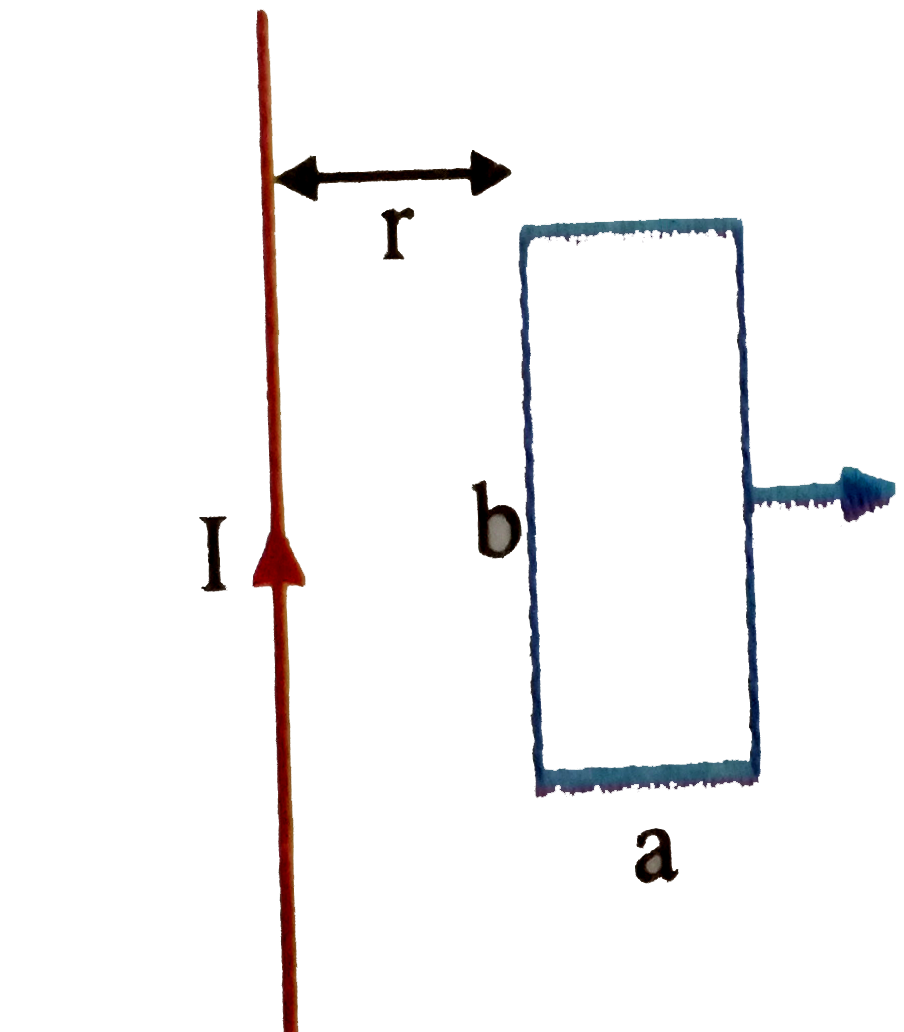
A rectangular loop of wire with dimensions shown in figure is coplanar with a long wire carrying current 'I'. The distance between the wire and the left side of the loop is r. The loop is pulled to the right as indicated. What are the directions of the induced current in the loop and the magnetic forces on the left and right sides of the loop when the loop is pulled ?
View SolutionA current loop in a magnetic field
View SolutionMagnetic lines form continuous closed loop. Why ?
View SolutionA bar magnet is dropped through a horizontal aluminium ring along the axis of the ring. What will be direction of induced current in the loop for the observer shown? What will be the direction of magnetic force experienced by the bar magnet?

View SolutionStatement-1 The net magnetic flux through a spherical surface enclosing north pole of a bar magnet as zero

Statement-2 Magnetic field lines always form closed loops
View SolutionOn meter length of wires carriers a constant current. The wire is bent to from a circular loop. The magnetic field at the centre of this loop is B. The same is now bent to form a circular loop of smaller radius to have four turns in the loop. The magnetic field at the centre of this loop B. The same is now bent to form a circular loop of smaller radius of have four turns in the loop. The magnetic field at the centre of this new loop is
View Solution