A sheet of relative permeability 100 is placed inside the solenoid, so as to fill the space. What will be the energy stored inside the solenoid?
To find the energy stored inside the solenoid when a sheet of relative permeability 100 is placed inside, we can follow these steps:
Step 1: Understand the formula for magnetic energy density
The magnetic energy density (u) inside a solenoid is given by the formula:
u=B22μ
where B is the magnetic field inside the solenoid, and μ is the magnetic permeability of the material inside the solenoid.
Step 2: Relate relative permeability to absolute permeability
The relative permeability (μr) is defined as:
μr=μμ0
where μ0 is the permeability of free space (vacuum). Given that μr=100, we can express μ in terms of μ0:
μ=μr⋅μ0=100⋅μ0
Step 3: Substitute μ into the energy density formula
Now, substituting μ back into the energy density formula:
u=B22μ=B22⋅(100⋅μ0)
This simplifies to:
u=B2200μ0
Step 4: Express the magnetic energy density in terms of μ0
We can further simplify the expression:
u=1200⋅B2μ0
Step 5: Final expression for energy density
Thus, the final expression for the magnetic energy density inside the solenoid with the sheet of relative permeability 100 is:
u=B2200μ0
Step 6: Calculate total energy stored (if needed)
If we need to find the total energy stored in the solenoid, we would multiply the energy density by the volume (V) of the solenoid:
U=u⋅V=B2200μ0⋅V
The magnetic energy density (u) inside a solenoid is given by the formula:
u=B22μ
where B is the magnetic field inside the solenoid, and μ is the magnetic permeability of the material inside the solenoid.
The relative permeability (μr) is defined as:
μr=μμ0
where μ0 is the permeability of free space (vacuum). Given that μr=100, we can express μ in terms of μ0:
μ=μr⋅μ0=100⋅μ0
Now, substituting μ back into the energy density formula:
u=B22μ=B22⋅(100⋅μ0)
This simplifies to:
u=B2200μ0
We can further simplify the expression:
u=1200⋅B2μ0
Thus, the final expression for the magnetic energy density inside the solenoid with the sheet of relative permeability 100 is:
u=B2200μ0
If we need to find the total energy stored in the solenoid, we would multiply the energy density by the volume (V) of the solenoid:
U=u⋅V=B2200μ0⋅V
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assignment (SECTION - A)55videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assignment (SECTION - B)22videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT(SECTION -D) Assertion-Reason type Question)15videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - comprehension3videos
ELECTRIC CHARGES AND FIELDS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions25videos
ELECTROMAGNETIC WAVES
Similar Questions
Knowledge Check
- Question 1 - Select One
An 8 cm long wire carrying a current of 10 A is placed inside a solenoid perpendicular to its axis. If the magnetic field inside the solenoid is 0.3 T, then magnetic force on the wire is
A0.14NB0.24NC0.34ND0.44N
An iron rod of volume 10−4m3 and relative permeability 1000 is placed inside a long solenoid wound with 5turns/cm. If a current of 0.5A is passed through the solenoid, then the magnetic moment of the rod is
View SolutionStatement I: An emf is induced ina long solenoid by a bar magnet that moves while totally inside the solenoid along axis of the solenoid.
Statement II: as the magnet moves inside the solenoid the flux through turns of the solenoid changes.View SolutionA 3⋅0cm wire carrying a current of 10A is placed inside a solenoid perpendicular to its axis. The magnetic field inside the solenoid is given to be 0⋅27T. What is the magnetic force on the wire?
View SolutionA solenoid S1 is placed inside another solenoid S2 as shown in the fig. The radii of the inner and the outer solenoids are r1 and r2 respectively and the numbers of turns per unit length are n1 and n2 respectively. Consider a length I of each solenoid. Calculate the mutual inductance between them.
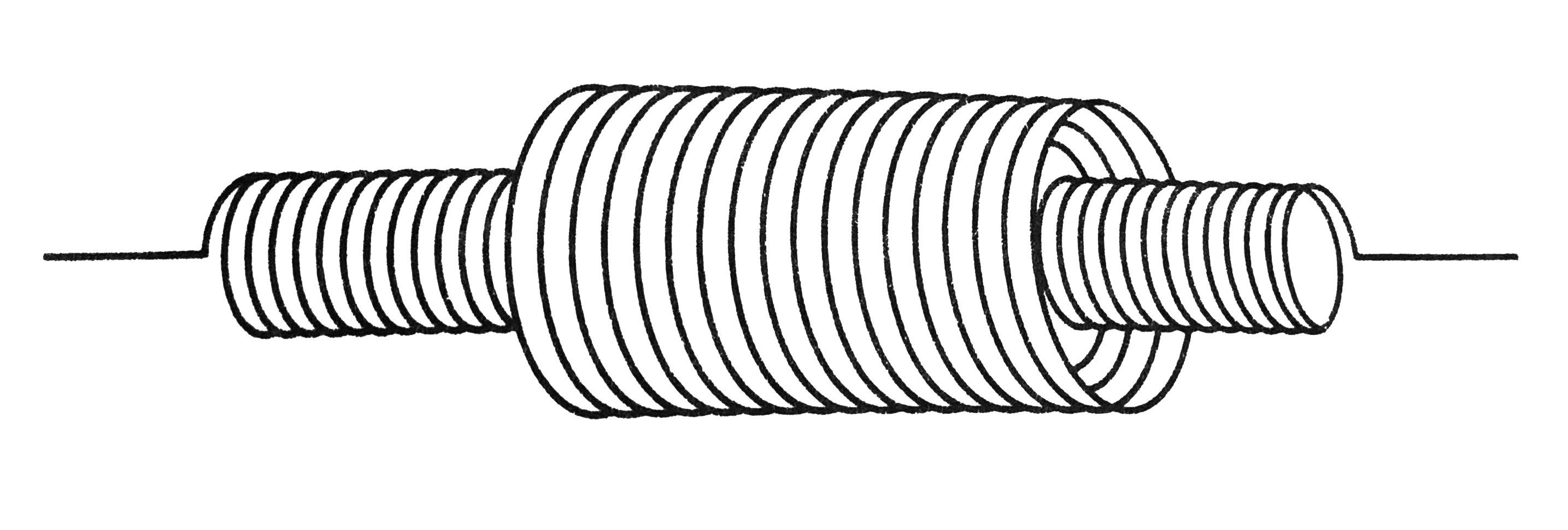 View Solution
View SolutionThe B-H curve for a ferromagnet is shown in the figure. The ferromagnet is placed inside a long solenoid with 1000 turns/cm. The current that should be passed in the solenoid to demagnetise the ferromagnet completely is :
View SolutionA solenoid has a core of a material with relative permeability 400. The windings of the solenoid are insulated from the core and carry a current of 2A. If the number of turns is 1000 per metre, calculate (i) H (ii) B (iii) Intensity of magnetisation I, and the magnetising current.
View Solution(a)Using Ampere's circuital law, obtain the expression for the magnetic field due to a long solenoid at a point inside the solenoid on its axis.
(b )How is the magnetic field inside a given solenoid made strong?View Solution

