A uniform magnetic field exists in the space B=B1ˆi+B2ˆj−B3ˆk. Find the magnetic flux through an area S, if the area S is in yz - plane.
To find the magnetic flux through an area S in the yz-plane when a uniform magnetic field is given by B=B1^i+B2^j−B3^k, we can follow these steps:
1. Identify the Area Vector:
Since the area S is in the yz-plane, the area vector A will be perpendicular to the yz-plane. Therefore, the area vector can be expressed as:
A=S^i
where S is the magnitude of the area and ^i indicates the direction along the x-axis.
2. Write the Magnetic Field Vector:
The magnetic field is given as:
B=B1^i+B2^j−B3^k
3. Calculate the Magnetic Flux:
The magnetic flux Φ through the area S is given by the dot product of the magnetic field B and the area vector A:
Φ=B⋅A
Substituting the expressions for B and A:
Φ=(B1^i+B2^j−B3^k)⋅(S^i)
4. Evaluate the Dot Product:
Using the properties of the dot product:
Φ=B1S(^i⋅^i)+B2S(^j⋅^i)−B3S(^k⋅^i)
Since ^i⋅^i=1, ^j⋅^i=0, and ^k⋅^i=0, we get:
Φ=B1S⋅1+B2S⋅0−B3S⋅0
Thus, simplifying gives:
Φ=B1S
5. Final Result:
The magnetic flux through the area S in the yz-plane is:
Φ=B1S
Since the area S is in the yz-plane, the area vector A will be perpendicular to the yz-plane. Therefore, the area vector can be expressed as:
A=S^i
where S is the magnitude of the area and ^i indicates the direction along the x-axis.
The magnetic field is given as:
B=B1^i+B2^j−B3^k
The magnetic flux Φ through the area S is given by the dot product of the magnetic field B and the area vector A:
Φ=B⋅A
Substituting the expressions for B and A:
Φ=(B1^i+B2^j−B3^k)⋅(S^i)
Using the properties of the dot product:
Φ=B1S(^i⋅^i)+B2S(^j⋅^i)−B3S(^k⋅^i)
Since ^i⋅^i=1, ^j⋅^i=0, and ^k⋅^i=0, we get:
Φ=B1S⋅1+B2S⋅0−B3S⋅0
Thus, simplifying gives:
Φ=B1S
The magnetic flux through the area S in the yz-plane is:
Φ=B1S
Similar Questions
A uniform magnetic field exists in the space →B=B1ˆi+B2ˆj+B3ˆk. Find the magnetic flux through an area →S if the area →S is in (i) x-y plane (ii) y-z plane (iii) z-x plane
View SolutionIn a region of space, the electric field is given by →E=8ˆi+4ˆj+3ˆk. The electric flux through a surface of area 100 units in the xy plane is
View SolutionIf an electric field is given by 10ˆi+3ˆj+4ˆk calculate the electric flux through a surface of area 10 units lying in yz plane
View SolutionA uniform electric field aˆi+bˆj intersects a surface of area A. What is the flux through this area if the surface lies (a) in the yz plane, (b) in the xz plane, (c) in the xy plane?
View SolutionA wire of length a'a carries a current I along the y-axis.A magnetic field exists given by
B=B0(3ˆi+2ˆj+ˆk)T
Calculate magnetic force in vector from and its magnitude
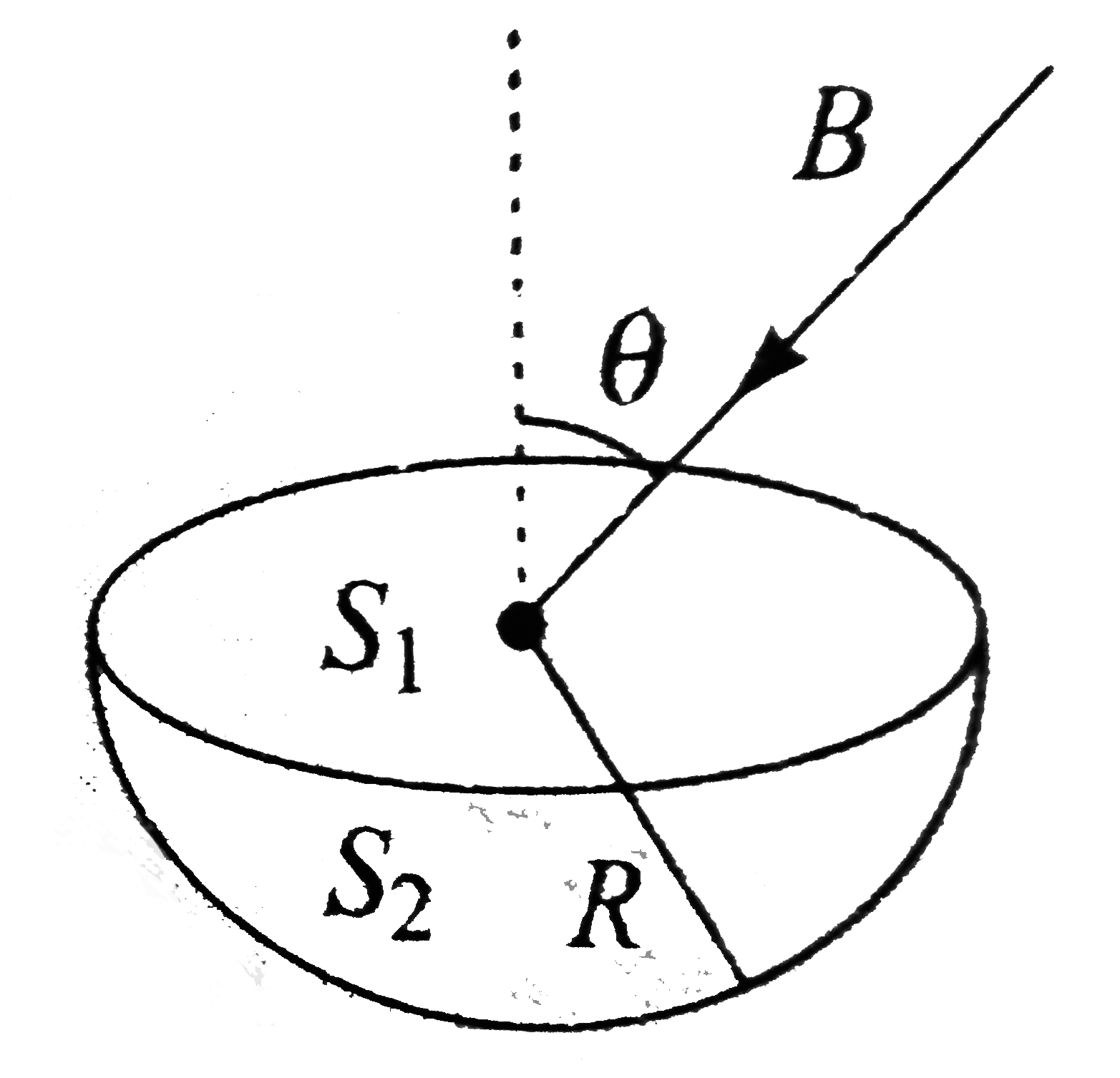
View SolutionConsider the hemispherical closed surface as shown in Fig. 3.19. if the hemisphere is a uniform magnetic field that makes an angle θ with the vertical, calculate the magnetic flux
(a) through the flat surface S1.
(b) through the hemisphere surface S2.
View SolutionA non uniform magnetic field B=B0tˆi in a region exists. A circular conducting loop of radius r and resistance R is placed with its plane in yz-plane. Determine the current through the loop and sense of the current.
View SolutionA circular loop of radius R carrying current I is kept in XZ plane. A uniform and constant magnetic field →B=(B0ˆi+2B0ˆj+3B0ˆk) exists in the region (B0− a positive constant). Then the magnitude of the torque acting on the loop will be
View SolutionA system consists of two parallel plane carrying currents producing a uniform magnetic field of induction B between the planes. Outside this space there is no magnetic field. The magnetic force acting per unit area of each plane is found to be B2/Nμ0. Find N.
View SolutionA square of side L meters lies in the x-y plane in a region, where the magnetic field is given by B=B0(2ˆi+3ˆj+4ˆk)T, where B0 is constant. The magnitude of flux passing through the square is
View Solution
