A uniformly wound solenoid coil of self inductance and resistance is broken up into two identical coils. These identical coils are then connected in parallel across a battery of negligible resistance. The time constant and steady state current will be
The correct Answer is:C
To solve the problem step by step, we will find the time constant and the steady state current for the given solenoid coil.
Step 1: Find the new inductance of each coil
The original solenoid has a self-inductance L=1.8×10−4H. When it is broken into two identical coils, the inductance of each coil L′ can be calculated as follows:
L′=L2=1.8×10−42=0.9×10−4H
Step 2: Find the equivalent inductance of the two coils in parallel
When two inductors are connected in parallel, the equivalent inductance Leq is given by:
1Leq=1L′+1L′=2L′
Thus,
Leq=L′2=0.9×10−42=0.45×10−4H=4.5×10−5H
Step 3: Find the new resistance of each coil
The original resistance R=6Ω. When the coil is divided into two identical coils, the resistance of each coil R′ is:
R′=R2=62=3Ω
Step 4: Find the equivalent resistance of the two coils in parallel
The equivalent resistance Req for two resistors in parallel is given by:
1Req=1R′+1R′=2R′
Thus,
Req=R′2=32=1.5Ω
Step 5: Calculate the time constant
The time constant τ for an RL circuit is given by:
τ=LeqReq
Substituting the values we found:
τ=4.5×10−51.5=3.0×10−5s=0.3×10−4s
Step 6: Calculate the steady state current
The steady state current I can be calculated using Ohm's law:
I=VReq
Given V=12V:
I=121.5=8A
Final Answers
- Time constant τ=0.3×10−4s
- Steady state current I=8A
---
The original solenoid has a self-inductance L=1.8×10−4H. When it is broken into two identical coils, the inductance of each coil L′ can be calculated as follows:
When two inductors are connected in parallel, the equivalent inductance Leq is given by:
The original resistance R=6Ω. When the coil is divided into two identical coils, the resistance of each coil R′ is:
The equivalent resistance Req for two resistors in parallel is given by:
The time constant τ for an RL circuit is given by:
The steady state current I can be calculated using Ohm's law:
- Time constant τ=0.3×10−4s
- Steady state current I=8A
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assignment (SECTION - B)
22 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assignment (SECTION - C)
10 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Try Yourself
42 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - comprehension
3 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions
25 Videos
Similar Questions
A uniform wound solenoidal coil of self inductance 1.8×10−4 henry and resistance 6 ohm is broken up into two identical coils. These identical coils are then connected in parallel across a 15-volt battery of negligible resistance. The time constant for the current in the circuit is.......seconds and the steady state current through the battery is ..............amperes.
View SolutionSelf - inductance 0.8×10−4H of a uniformly wound solenoid, having resistance 3 Ω is broken up into two indentical coils. Those coils are connected in series across a 6 V battery of negligible resistance. Find time constant and steady state current.
View SolutionA uniformly wound solenoid coil of slf inductnace 4 mH and resistance 12Ω is broken int two parts. These two coil are connected in parallel across is 12V battery. The steady current through battery is
View SolutionA uniformly wound long solenoid of inductance L and resistance R is cut into two parts in the ratio η:1, which are then connected in parallel. The combination is then connected to a cell of emf E. The time constant of the circuit is
View SolutionA coil of inductance 8.4mH and resistance 6 Ω is connected to a 12V battery. The current in the coil is 1.0A at approximately the time.
View SolutionA coil of inductance 8.4 mH and resistance 6(Ω) is connected to a 12 V battery. The current in the coil is 1.0 A at approximately the time
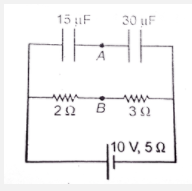
View SolutionThe figure shows two capacitors connected in parallel with two resistance and a battery of emf 10V , internal resistance 5Ω. At steady state.
View SolutionA coil of self inductance 2.5 H and resistance 20Ω is connected to a battery of emf 120 V having internal resistance of 5Ω. Find :
The time constant of the circuit
View SolutionA coil of inductance 300mh and resistance 2Ω is connected to a source of voltage 2V. The current reaches half of its steady state value in
View SolutionTwo resistors of 4 Ω and 6 Ω are connected in parallel. The combination is connected across a 6 V battery of negligible resistance. (i) Draw a circuit diagram. (ii) Calculate :the current through the battery
View Solution
