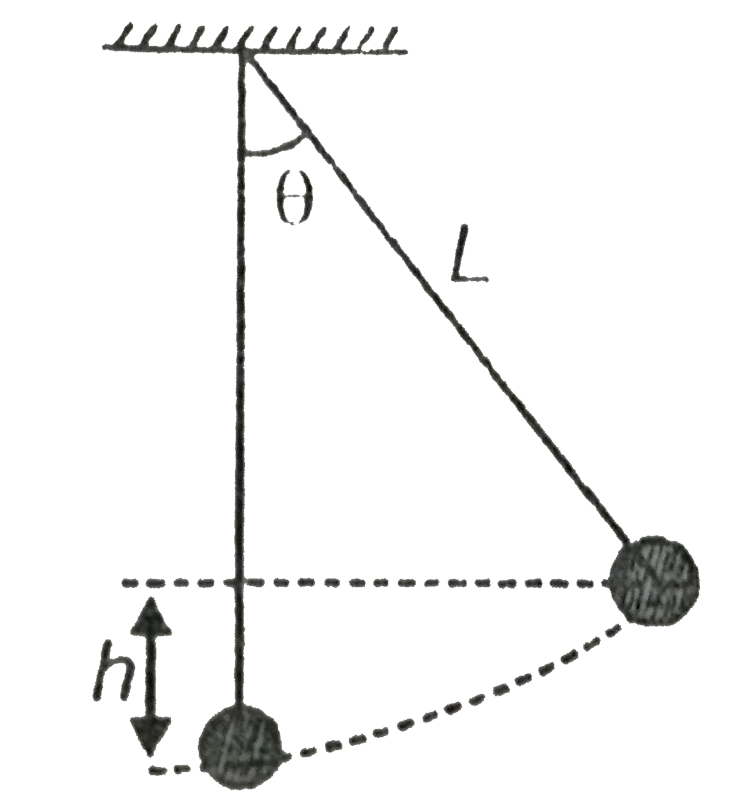
A simple pendulum with bob of mass m and conducting wire of length L swings under gravity through an angle 2θ. The earth's magnetic field component in the direction perpendicular to swing is B. The maximum potential difference induced across the pendulum is
a.2BLsin(θ2)√gL
b.BLsin(θ2)√(gl)
c..BLsin(θ2).(gL)3/2
d.BLsin(θ2).(gL)2
The correct Answer is:B
Step by step video & image solution for A simple pendulum with bob of mass m and conducting wire of length L swings under gravity through an angle 2theta. The earth's magnetic field component in the direction perpendicular to swing is B. The maximum potential difference induced across the pendulum is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assignment (SECTION - B)
22 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assignment (SECTION - C)
10 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Try Yourself
42 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - comprehension
3 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions
25 Videos
Similar Questions
A simple pendulum with bob of mass m and conducting wire of length L swings under gravity through an angle 2θ. The earth's magnetic field component in the direction perpendicular to swing is B. The maximum potential difference induced across the pendulum is

View SolutionA simple pendulum with a bob of mass m and a conducting wire of length L, swings under gravity with an angular amplitude 2θ. If the horizontal component of the earth's magnetic field perpendicular to the plane of motion of the pendulum is B, then the maximum emf induced across the pendulum is
View SolutionA conducting rod AB of length l=1m is moving at a velocity vA=4m/s making an angle 30∘ with its length. A uniform magnetic field B=2T exists in a direction perpendicular to the plane of motion. Then
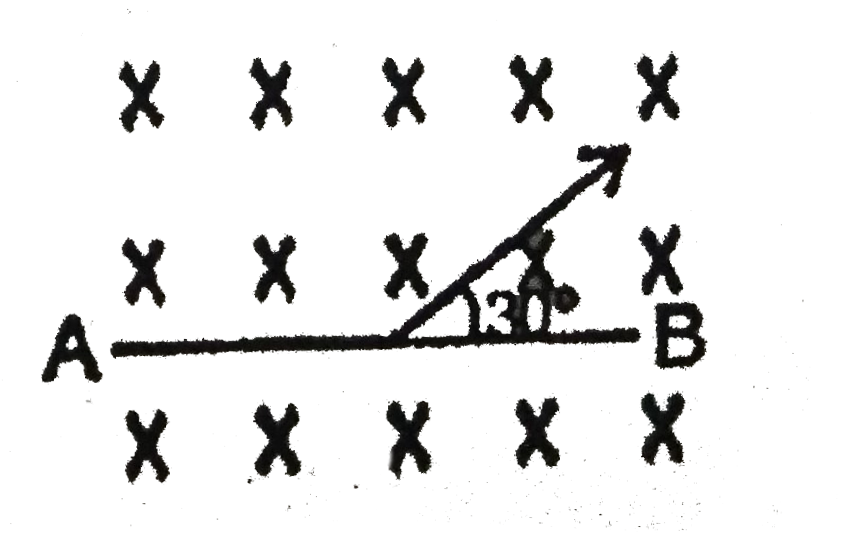
View SolutionA conducting rod PQ of length l=2m is moving at a speed of 2ms−1 making an angle of 30∘ with its length. A uniform magnetic field B=2T exists in a direction perpendicular to the plane of motin. Then

View SolutionA conducting rod PQ of length l=2m is moving at a speed of 2ms−1 making an angle of 30∘ with its length. A uniform magnetic field B=2T exists in a direction perpendicular to the plane of motin. Then

View SolutionA simple pendulum of length 1 m with a bob of mass m swings with an angular amplitude 30∘. Then (g=9.8m/s2
View SolutionA simple pendulum has a bob of mass m and swings with an angular amplitude ϕ. The tension in thread is T. At a certain time the string makes an angle θ with the vertical (θ≤ϕ)
View SolutionA conducting rod AB of lemgth 2 l moves through a uniform magnetic field B. The rod makes an angle θ with the vertical while it falls with a uniform linear velocity v. The potential difference between the two ends of the rod is Bv2l .

View SolutionA uniform wire of resistance per unit length λ is bent into semicircle of radius a. The wire rotates with angular velocity ω in a vertical plane about a orizontal is passing through C. A uniform magnetic field B exists in space in a direction perpendicular to paper inwards.
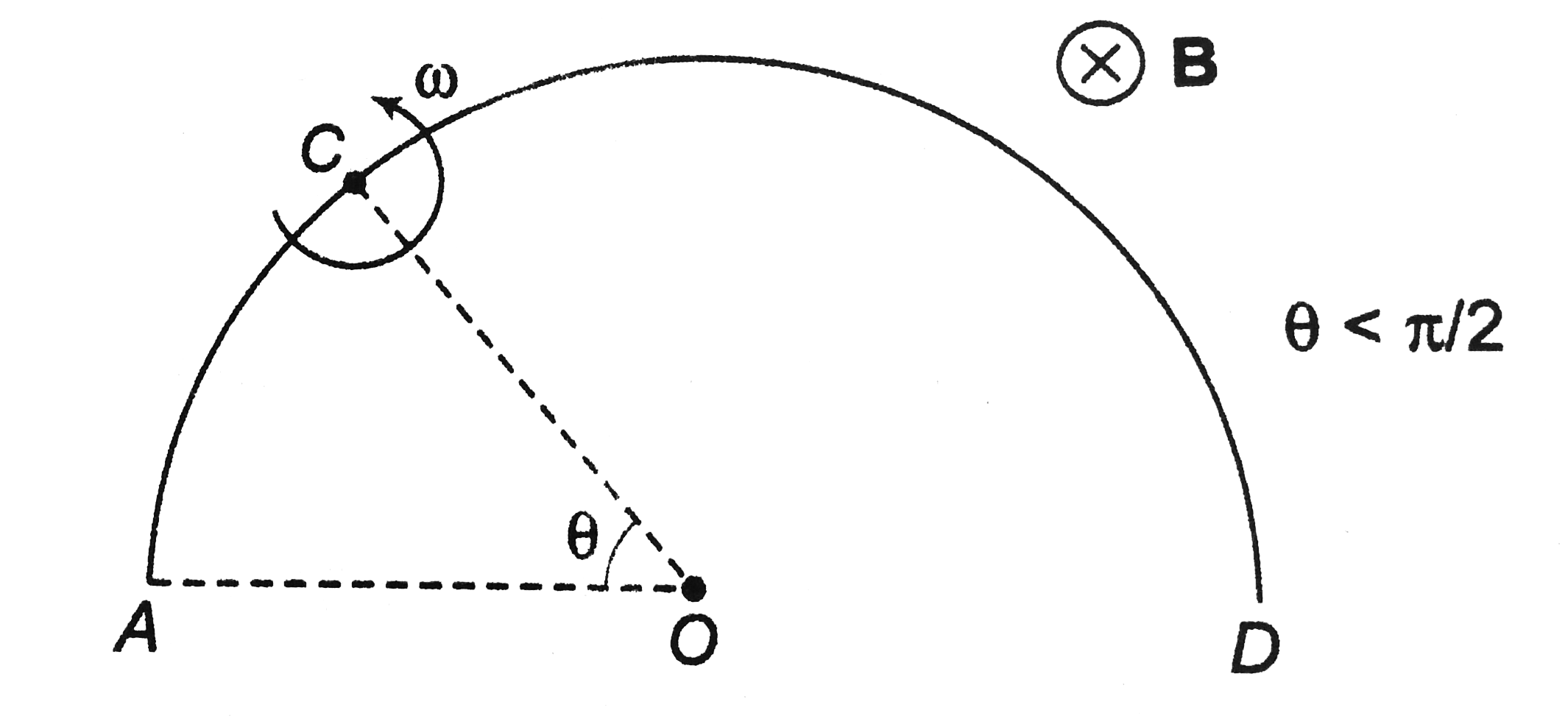
a. Calculate potential difference between points A and D. which point is at higher potential?
b. If points A and D are connected by a conducting wire of zero resistance, find the potential difference between A and C.
View SolutionA straight rod of length L is rotating about an axis passing through O and perpendicular to the plane. In the space a uniform magnetic field B exits normal to the plane of rotation. Potential difference between a & b is equal to

View Solution
