Two point P, Q are taken at random on a straight line OA of length a. The chance that PQ>b, where b<a is
|a−b|a
|b−a|a
(a−b)2a2
(b−a)2b2
The correct Answer is:B
To solve the problem step by step, we will analyze the situation of selecting two random points P and Q on a straight line segment OA of length a and find the probability that the distance PQ is greater than b, where b<a.
1. Understanding the Problem:
- We have a line segment OA of length a.
- We need to find the probability that the distance between two randomly chosen points P and Q on this line segment is greater than b.
2. Defining the Points:
- Let P be at position x and Q be at position y on the line segment OA.
- The coordinates of P and Q can be represented as P(x) and Q(y), where 0≤x,y≤a.
3. Distance Between Points:
- The distance PQ can be expressed as |x−y|.
- We want to find the condition where |x−y|>b.
4. Setting Up the Inequality:
- The condition |x−y|>b means that either x−y>b or y−x>b.
- This can be rewritten as two inequalities:
- x>y+b
- y>x+b
5. Visualizing the Problem:
- We can visualize the problem in a coordinate plane where the x-axis represents the position of point P and the y-axis represents the position of point Q.
- The area of interest is the square defined by 0≤x≤a and 0≤y≤a, which has an area of a2.
6. Finding the Area Where |x−y|>b:
- The lines y=x+b and y=x−b divide the square into regions.
- The area where |x−y|≤b is a band of width 2b around the line y=x.
- The area of this band can be calculated as the area of the square minus the area of the two triangles formed outside this band.
7. Calculating the Area of the Band:
- The area of the band is given by:
Area of band=a2−Area of two triangles
- Each triangle has a base and height of a−b, so the area of one triangle is 12(a−b)(a−b)=(a−b)22.
- Therefore, the total area of the two triangles is (a−b)2.
8. Final Area Calculation:
- The area where |x−y|>b is:
Area=a2−(a−b)2=a2−(a2−2ab+b2)=2ab−b2
9. Calculating the Probability:
- The probability P that |x−y|>b is given by the ratio of the area where |x−y|>b to the total area of the square:
P=2ab−b2a2
Final Result:
The probability that the distance PQ is greater than b is:
P=(a−b)2a2
- We have a line segment OA of length a.
- We need to find the probability that the distance between two randomly chosen points P and Q on this line segment is greater than b.
- Let P be at position x and Q be at position y on the line segment OA.
- The coordinates of P and Q can be represented as P(x) and Q(y), where 0≤x,y≤a.
- The distance PQ can be expressed as |x−y|.
- We want to find the condition where |x−y|>b.
- The condition |x−y|>b means that either x−y>b or y−x>b.
- This can be rewritten as two inequalities:
- x>y+b
- y>x+b
- We can visualize the problem in a coordinate plane where the x-axis represents the position of point P and the y-axis represents the position of point Q.
- The area of interest is the square defined by 0≤x≤a and 0≤y≤a, which has an area of a2.
- The lines y=x+b and y=x−b divide the square into regions.
- The area where |x−y|≤b is a band of width 2b around the line y=x.
- The area of this band can be calculated as the area of the square minus the area of the two triangles formed outside this band.
- The area of the band is given by:
Area of band=a2−Area of two triangles
- Each triangle has a base and height of a−b, so the area of one triangle is 12(a−b)(a−b)=(a−b)22.
- Therefore, the total area of the two triangles is (a−b)2.
- The area where |x−y|>b is:
Area=a2−(a−b)2=a2−(a2−2ab+b2)=2ab−b2
- The probability P that |x−y|>b is given by the ratio of the area where |x−y|>b to the total area of the square:
P=2ab−b2a2
The probability that the distance PQ is greater than b is:
P=(a−b)2a2
Topper's Solved these Questions
- PROBABILITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PROBABILITYEXERCISE - ASSIGNMENT SECTION-I (subjective type questions)
8 Videos
- PRINCIPLE OF MATHEMATICAL BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PRINCIPLE OF MATHEMATICAL EXERCISE - Section-D:(Assertion-Reason Type Questions)
11 Videos
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - J) Aakash Challengers Questions
8 Videos
Similar Questions
Two points are taken at random on the given straight line segment of length a. The probability for the distance between them to exceed a given length c, where 0<c<a, is
View SolutionIf two points are taken at random on the circumference of circle, the chance that their distance apart is greater than the radius of the circle is
View Solution
Knowledge Check
The points P,Q,R and S lie in that order on a straight line . The midpoint of ¯¯¯¯¯¯QS is R and the midpoint of ¯¯¯¯¯PS is Q . The length of ¯¯¯¯¯¯QR is x feet and the length of ¯¯¯¯¯¯PQ is 4x−16 feet . What is the length , in feet , of ¯¯¯¯¯PS?
A32B20C16D8
Three points P,QandR are selected at random from the cirumference of a circle. Find the probability p that the point lie on a semi-circle
View SolutionTwo circles intersect each other at points A and B. A straight line PAQ cuts the circles at P and Q. If the tangents at P and Q intersect at point T, show that the points P, B, Q and T are concylic.
View SolutionA straight lien is drawn from a fixed point O metting a fixed straight line P. A point Q is taken on the line OP such that OP.OQ is constant. Show that the locus of Q is a circle.
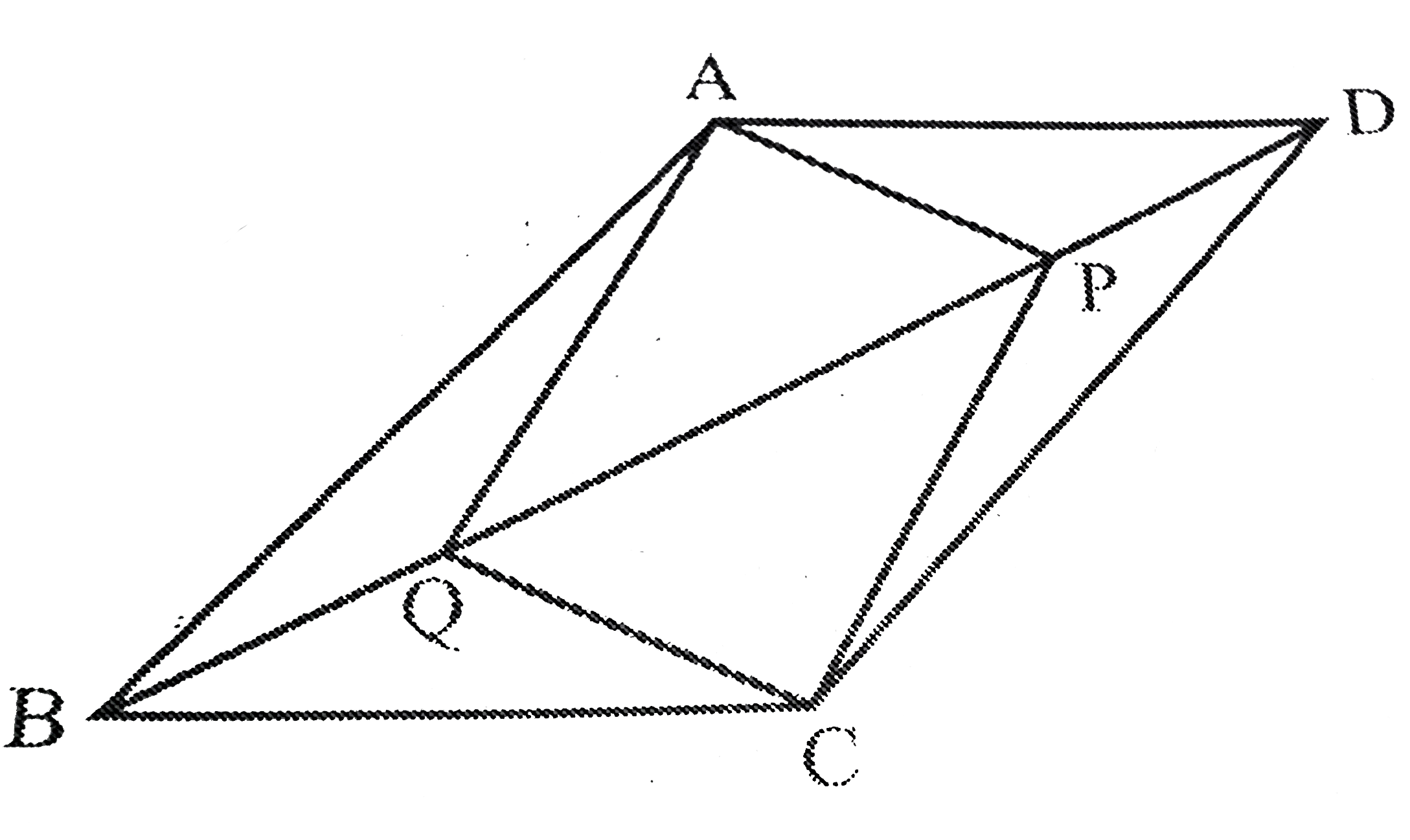
View SolutionIn parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP=BQ. Show that:
(i) ΔAPD≅ΔCQB
(ii) AP=CQ
(iii) ΔAQB≅ΔCPD
(iv) AQ=CP
(v) APCQ is a parallelogram.
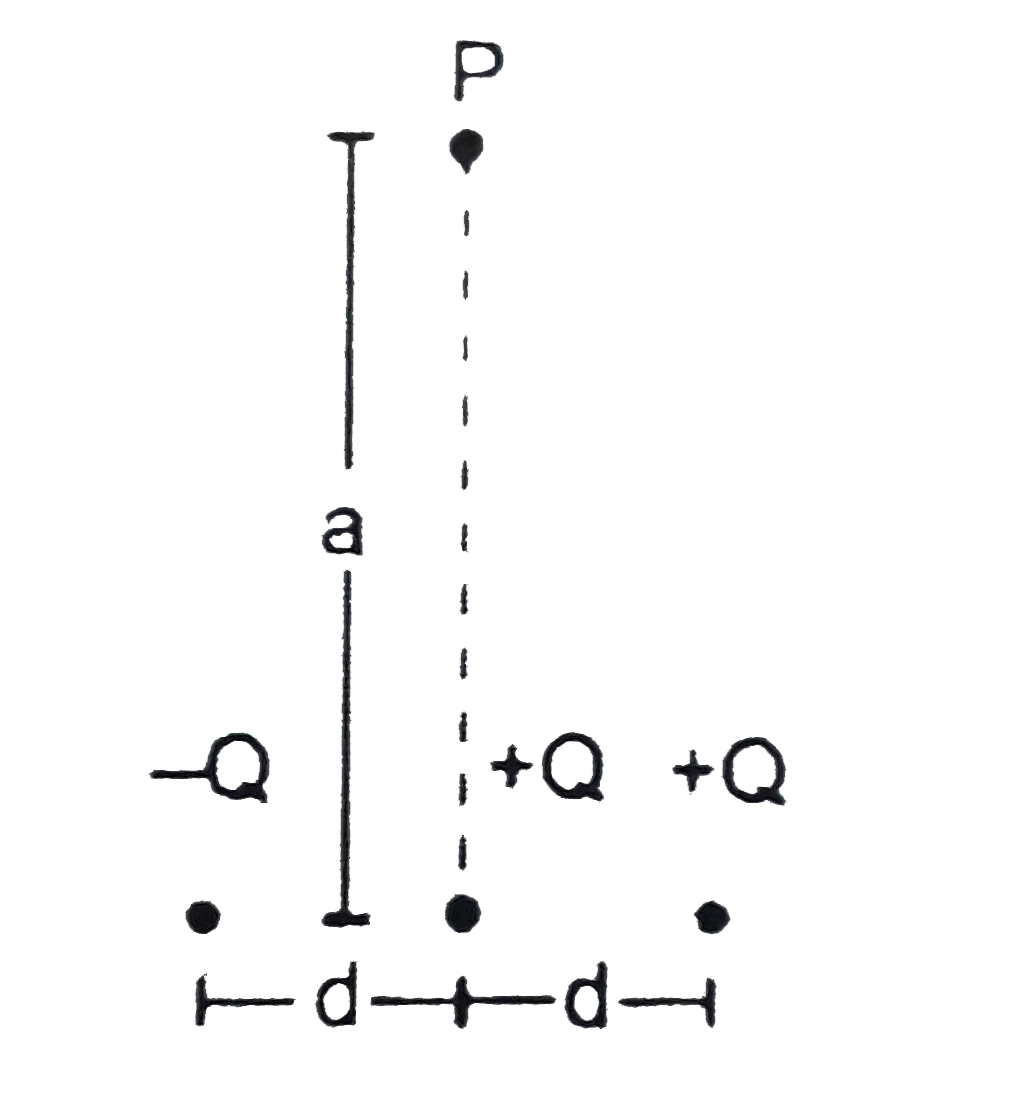
View SolutionThree point charges -Q, Q and Q are placed on a straight line with distance d between charges as shown. Find the magnitude of the electric field at the point P in the configuration shown which is at a distance a from middle charge Q in the system provided that a>>d. Take 2Qd=p.
View SolutionThree charge q,Q and −4q are placed in a straight line , line of length Lat points distant 0,L/2 and L respectively from one end. In order to make the net force on q zero, the charge Q must be equal to
View SolutionA straight line is drawn from a fixed point O meeting a fixed straight line in P. A point Q is taken on the line OP such that OP.OQ is constant. Show that the locus of Q is a circle.
View Solution
