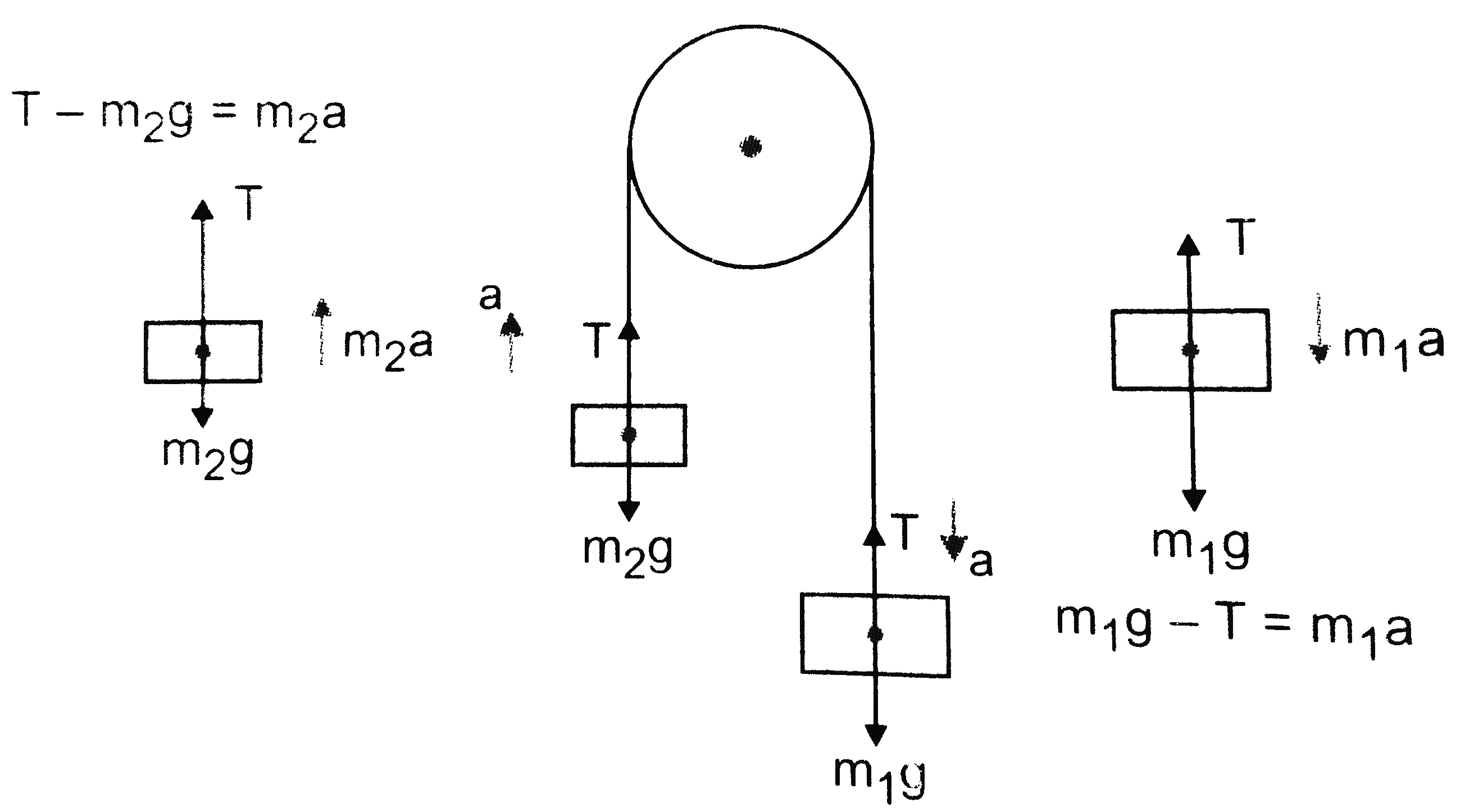
Two masses 2 kg and 4 kg are connected at two ends of light inextensible string passing over a frictionless pulley . If the masses are released , then find the accelaration of the masses and the tension in the string .
To solve the problem, we will follow these steps:
Step 1: Identify the forces acting on each mass
For the 2 kg mass (m1):
- Weight (W1) = m1 * g = 2 kg * 10 m/s² = 20 N (acting downward)
- Tension (T) in the string (acting upward)
For the 4 kg mass (m2):
- Weight (W2) = m2 * g = 4 kg * 10 m/s² = 40 N (acting downward)
- Tension (T) in the string (acting upward)
Step 2: Write the equations of motion for each mass
For the 4 kg mass (m2):
- The net force acting on it is (Weight - Tension):
W2−T=m2⋅a
40−T=4a (Equation 1)
For the 2 kg mass (m1):
- The net force acting on it is (Tension - Weight):
T−W1=m1⋅a
T−20=2a (Equation 2)
Step 3: Solve the equations simultaneously
We will add Equation 1 and Equation 2 to eliminate T:
(40−T)+(T−20)=4a+2a
This simplifies to:
40−20=6a
20=6a
a=206=103m/s2
Step 4: Find the tension in the string
Now, we can substitute the value of acceleration (a) back into either Equation 1 or Equation 2 to find T. We will use Equation 2:
T−20=2⋅103
T−20=203
T=20+203
To combine the terms, convert 20 to a fraction:
T=603+203
T=803N
Final Answers
- Acceleration (a) = 103m/s2 or approximately 3.33m/s2
- Tension (T) = 803N or approximately 26.67N
For the 2 kg mass (m1):
- Weight (W1) = m1 * g = 2 kg * 10 m/s² = 20 N (acting downward)
- Tension (T) in the string (acting upward)
- Weight (W2) = m2 * g = 4 kg * 10 m/s² = 40 N (acting downward)
- Tension (T) in the string (acting upward)
For the 4 kg mass (m2):
- The net force acting on it is (Weight - Tension):
W2−T=m2⋅a
40−T=4a (Equation 1)
- The net force acting on it is (Tension - Weight):
T−W1=m1⋅a
T−20=2a (Equation 2)
We will add Equation 1 and Equation 2 to eliminate T:
(40−T)+(T−20)=4a+2a
This simplifies to:
40−20=6a
20=6a
a=206=103m/s2
Now, we can substitute the value of acceleration (a) back into either Equation 1 or Equation 2 to find T. We will use Equation 2:
T−20=2⋅103
T−20=203
T=20+203
To combine the terms, convert 20 to a fraction:
T=603+203
T=803N
- Acceleration (a) = 103m/s2 or approximately 3.33m/s2
- Tension (T) = 803N or approximately 26.67N
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Illustration
7 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - TRY YOURSELF
69 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
Similar Questions
Two masses 2 kg and 4 kg are connected at the two ends of light inextensible string passing over a frictionless pulley. If the masses are released, then find the acceleration of the masses and the tension in the string.
View SolutionTwo masses of 3 kg and 4 kg are connected at the two ends of a light inextensible string that passes over a frictionless pulley. Find the acceleration of the masses and the tension in the string, when the masses are released.
View Solution
Knowledge Check
Two masses 8 kg and 12 kg are connected at the two ends of a light in extensible string that passes over a friction less pulley Find the acceleration of the masses and tension in the string , when the masses are released
 . Ag4Bg5Cg8Dg6
. Ag4Bg5Cg8Dg6Two blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.
 A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
Two masses 8 kg and 12 kg are connected at the two ends of a light in extensible string that passes over a friction less pulley. Find the acceleration of the masses and tension in the string , when the masses are released
 .
.
View SolutionTwo bodies of masses 1 kg and 2 kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string
View SolutionTwo masses M1=5kg, M2=10kg are connected at the ends of an inextensible string passing over a frictionless pulley as shown. When masses are released, then acceleration of masses will be
(a) g (b) g2 (c) g3 (d) g4
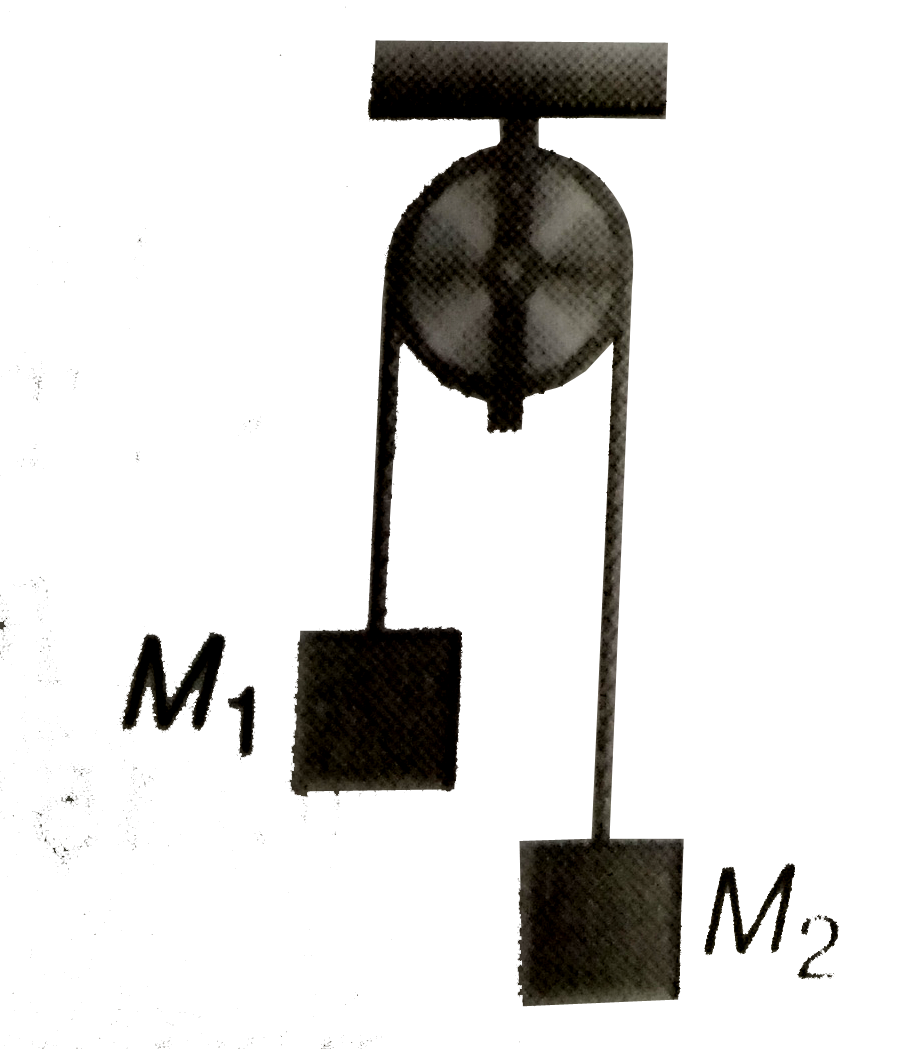
View SolutionTwo masses 5 kg and 3 kg are suspended from the ends of an unstretchable light string passing over a frictionless pulley. When the masses are released, the thrust on the pulley is (g = 10ms−2)
View SolutionTwo unequal masses of 1 kg and 2 kg are attached at the two ends of a light inextensible string passing over a smooth pulley as shown . If the system is released from rest, find the work done by string on both the blocks in 1 s.
(Take g=10m/s2).
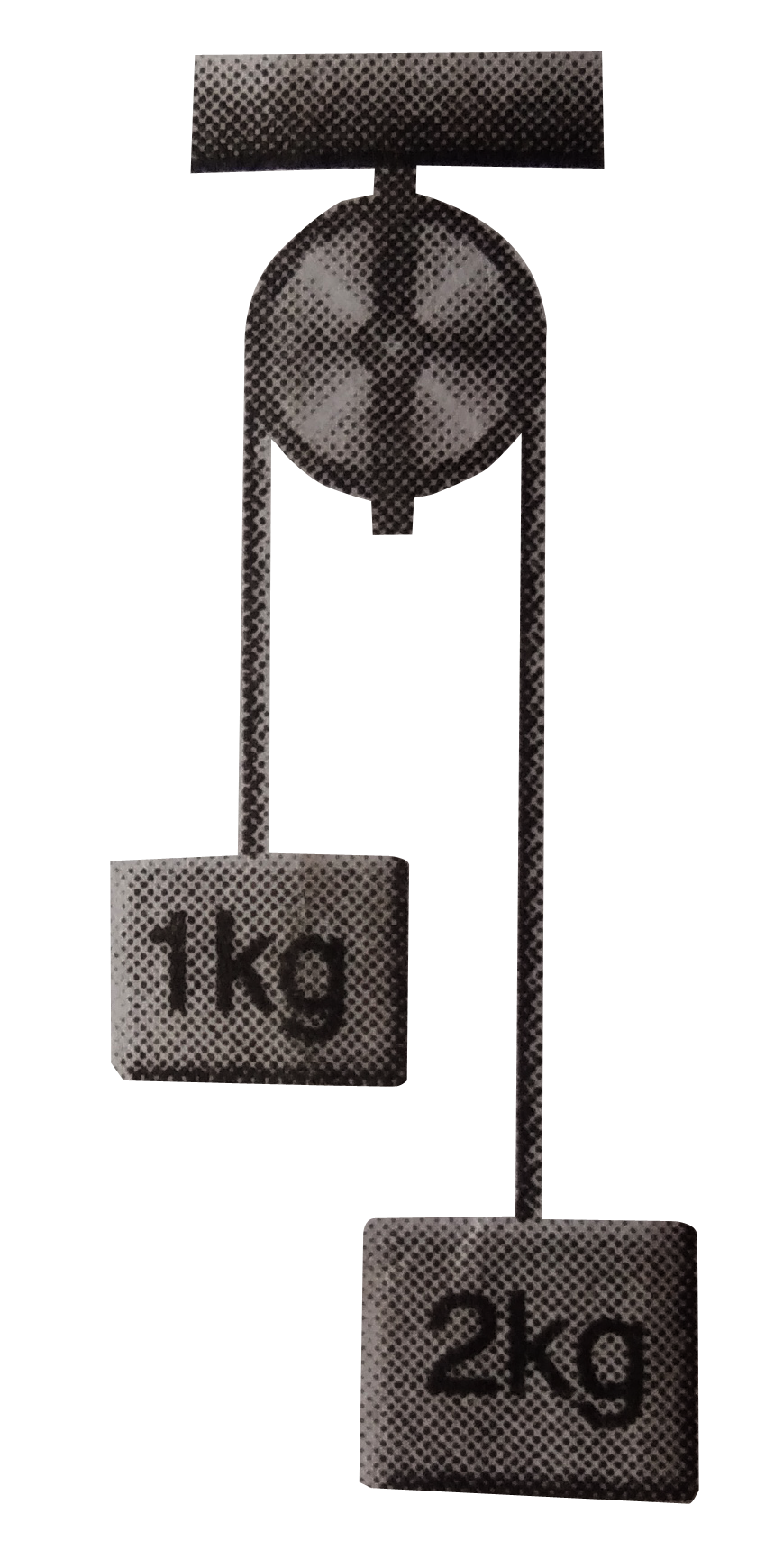
View SolutionTwo blocks of masses 6kg and 4kg are attached to the two ends of a massless string passing over a smooth fixed pulley. if the system is released, the acceleration of the centre of mass of the system will be
View Solution
