Two masses 5 kg and 3 kg are suspended from the ends of an unstretchable light string passing over a frictionless pulley. When the masses are released, the thrust on the pulley is (g = 10ms−2)
80 N
37.5 N
150 N
75 N
The correct Answer is:D
To solve the problem of finding the thrust on the pulley when two masses (5 kg and 3 kg) are suspended from a light string over a frictionless pulley, we can follow these steps:
Step 1: Identify the Forces Acting on Each Mass
- For the 3 kg mass, the weight acting downwards is W1=3g.
- For the 5 kg mass, the weight acting downwards is W2=5g.
- The tension in the string is denoted as T.
Step 2: Write the Equations of Motion
- For the 3 kg mass (which will accelerate upwards), the equation of motion can be written as:
T−3g=3a(Equation 1)
- For the 5 kg mass (which will accelerate downwards), the equation of motion can be written as:
5g−T=5a(Equation 2)
Step 3: Substitute the Value of g
Given g=10m/s2:
- Substitute g into the equations:
- Equation 1 becomes:
T−30=3a
- Equation 2 becomes:
50−T=5a
Step 4: Solve the Equations Simultaneously
- Rearranging Equation 1 gives:
T=3a+30(Equation 3)
- Rearranging Equation 2 gives:
T=50−5a(Equation 4)
Step 5: Set Equations 3 and 4 Equal to Each Other
Since both equations equal T:
3a+30=50−5a
Step 6: Solve for Acceleration (a)
- Rearranging gives:
3a+5a=50−30
8a=20
a=208=2.5m/s2
Step 7: Calculate the Tension (T)
- Substitute a back into Equation 3:
T=3(2.5)+30=7.5+30=37.5N
Step 8: Calculate the Thrust on the Pulley
- The thrust on the pulley (which is the total force acting on it due to both tensions) is given by:
Thrust=2T=2×37.5=75N
Final Answer
The thrust on the pulley is 75N.
---
- For the 3 kg mass, the weight acting downwards is W1=3g.
- For the 5 kg mass, the weight acting downwards is W2=5g.
- The tension in the string is denoted as T.
- For the 3 kg mass (which will accelerate upwards), the equation of motion can be written as:
T−3g=3a(Equation 1)
- For the 5 kg mass (which will accelerate downwards), the equation of motion can be written as:
5g−T=5a(Equation 2)
Given g=10m/s2:
- Substitute g into the equations:
- Equation 1 becomes:
T−30=3a
- Equation 2 becomes:
50−T=5a
- Rearranging Equation 1 gives:
T=3a+30(Equation 3)
- Rearranging Equation 2 gives:
T=50−5a(Equation 4)
Since both equations equal T:
3a+30=50−5a
- Rearranging gives:
3a+5a=50−30
8a=20
a=208=2.5m/s2
- Substitute a back into Equation 3:
T=3(2.5)+30=7.5+30=37.5N
- The thrust on the pulley (which is the total force acting on it due to both tensions) is given by:
Thrust=2T=2×37.5=75N
The thrust on the pulley is 75N.
Similar Questions
Two masses 2 kg and 4 kg are connected at the two ends of light inextensible string passing over a frictionless pulley. If the masses are released, then find the acceleration of the masses and the tension in the string.
View SolutionTwo masses 2 kg and 4 kg are connected at two ends of light inextensible string passing over a frictionless pulley . If the masses are released , then find the accelaration of the masses and the tension in the string .
View Solution
Knowledge Check
Two blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.
 A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2Two masses 8 kg and 12 kg are connected at the two ends of a light in extensible string that passes over a friction less pulley Find the acceleration of the masses and tension in the string , when the masses are released
 . Ag4Bg5Cg8Dg6
. Ag4Bg5Cg8Dg6
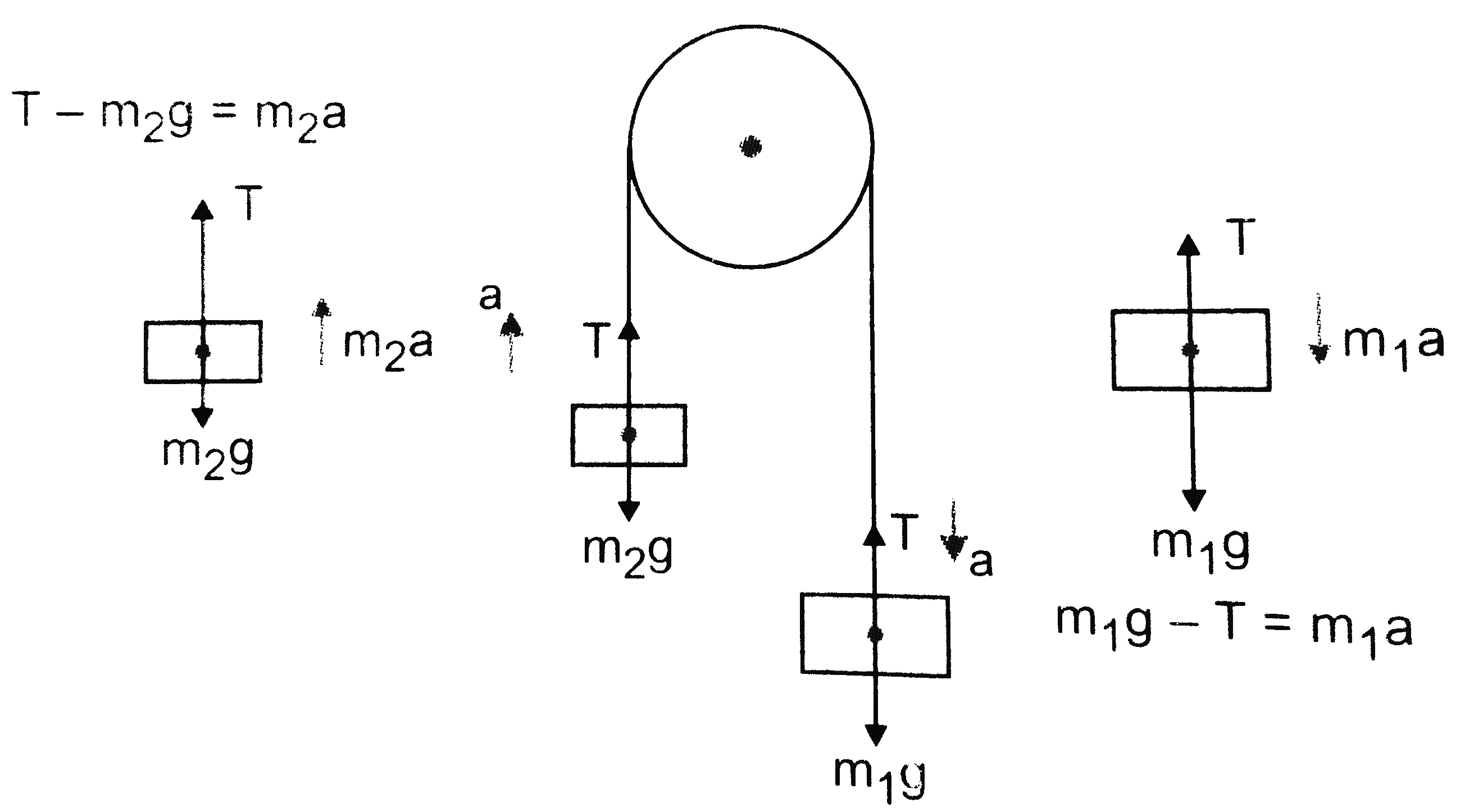
Two masses M1=5kg, M2=10kg are connected at the ends of an inextensible string passing over a frictionless pulley as shown. When masses are released, then acceleration of masses will be
(a) g (b) g2 (c) g3 (d) g4
View SolutionTwo bodies of masses 4 kg and 6 kg are tied to the ends of a massless string. The string passes over a frictionless pulley. The acceleration of the system is :
View SolutionTwo blocks of masses m1=4kg and m2=2kg are connected to the ends of a string which passes over a massless, frictionless pulley. The total downwards thrust on the pulley is nearly

View SolutionTwo masses of 3 kg and 4 kg are connected at the two ends of a light inextensible string that passes over a frictionless pulley. Find the acceleration of the masses and the tension in the string, when the masses are released.
View SolutionTwo masses 8 kg and 12 kg are connected at the two ends of a light in extensible string that passes over a friction less pulley. Find the acceleration of the masses and tension in the string , when the masses are released
 .
.
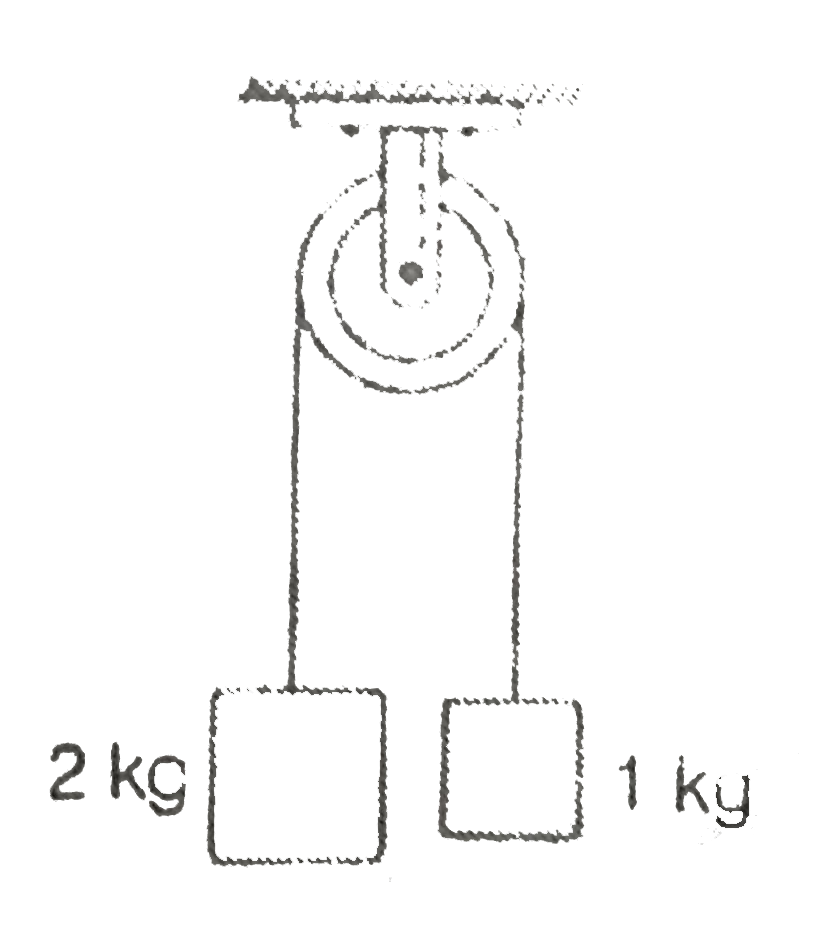
View SolutionTwo blocks of masses 2 kg and 1 kg respectively are tied to the ends of a string which passes over a light frictionless pulley . The masses are held at rest at the same horizontal level and then released . The distance traversed by centre of mass in 2 s is ( g = 10 m/s2)
View Solution
