In the figure shown coefficient of friction between 8 kg block and surface is zero and coefficient of friction ( both static and kinetic ) between the blocks is 0.4. A force of 20 N is applied on 8 kg block as shown . The acceleration of 4 kg mass is [ g = 10m/s2]
A. 4m/s2
B. 0.5m/s2
C. 53m/s2
D. 35m/s2
4m/s2
0.5m/s2
53m/s2
35m/s2
The correct Answer is:C
To solve the problem step-by-step, we will analyze the forces acting on the blocks and apply Newton's laws of motion.
Step 1: Identify the forces acting on the 4 kg block
The only force acting on the 4 kg block (let's call it Block B) is the frictional force exerted by the 8 kg block (Block A). The frictional force can be calculated using the formula:
Ffriction=μ⋅m⋅g
where:
- μ=0.4 (coefficient of friction between the blocks),
- m=4kg (mass of Block B),
- g=10m/s2 (acceleration due to gravity).
Step 2: Calculate the frictional force
Substituting the values into the formula:
Ffriction=0.4⋅4kg⋅10m/s2
Ffriction=0.4⋅40
Ffriction=16N
Step 3: Determine the maximum acceleration of the 4 kg block
Using Newton's second law F=m⋅a, we can find the acceleration of Block B:
Ffriction=m⋅a
16N=4kg⋅a
To find a:
a=16N4kg
a=4m/s2
Step 4: Analyze the system when both blocks move together
Next, we consider the scenario where both blocks move together. The total mass of the system is:
mtotal=8kg+4kg=12kg
The total force applied on the system is 20 N. According to Newton's second law:
Fnet=mtotal⋅a
Thus:
20N=12kg⋅a
To find a:
a=20N12kg
a=53m/s2
Conclusion
The acceleration of the 4 kg mass when both blocks move together is:
a=53m/s2
Final Answer
The correct option is C: 53m/s2.
---
The only force acting on the 4 kg block (let's call it Block B) is the frictional force exerted by the 8 kg block (Block A). The frictional force can be calculated using the formula:
Ffriction=μ⋅m⋅g
where:
- μ=0.4 (coefficient of friction between the blocks),
- m=4kg (mass of Block B),
- g=10m/s2 (acceleration due to gravity).
Substituting the values into the formula:
Ffriction=0.4⋅4kg⋅10m/s2
Ffriction=0.4⋅40
Ffriction=16N
Using Newton's second law F=m⋅a, we can find the acceleration of Block B:
Ffriction=m⋅a
16N=4kg⋅a
To find a:
a=16N4kg
a=4m/s2
Next, we consider the scenario where both blocks move together. The total mass of the system is:
mtotal=8kg+4kg=12kg
The total force applied on the system is 20 N. According to Newton's second law:
Fnet=mtotal⋅a
Thus:
20N=12kg⋅a
To find a:
a=20N12kg
a=53m/s2
The acceleration of the 4 kg mass when both blocks move together is:
a=53m/s2
The correct option is C: 53m/s2.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - ASSIGNMENT ( SECTION -C) objective type questions (More than one options are correct)
21 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - ASSIGNMENT ( SECTION -D) Linked Comprehension Type Question
1 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - ASSIGNMENT ( SECTION -A)
50 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
Similar Questions
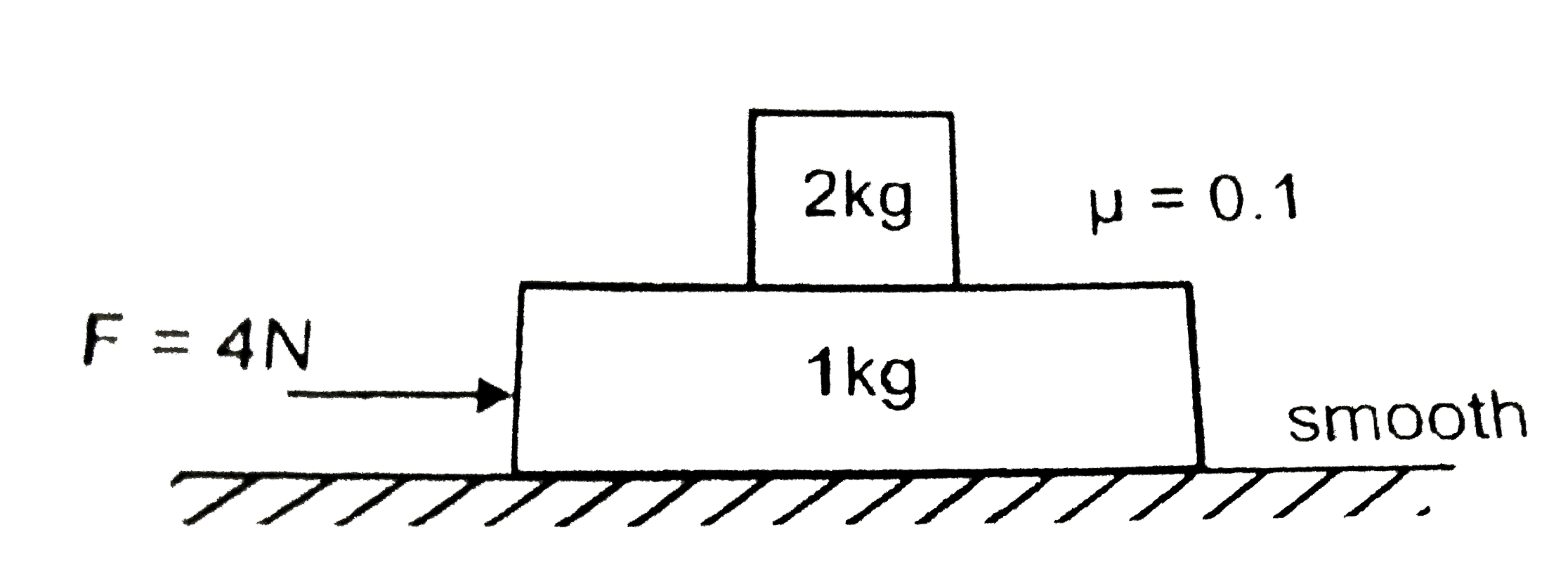
A block of mass 2 kg is placed on the floor. The coefficient of static friction it 0.4. A force F of 2.5 N is applied on the block, as shown. calculate the force of friction between the block and the floor. (g=9.8m/s2).
View Solution2gk block is kept on 1kg block as shown . The friction between 1kg block and fixed surface is absent and the coefficient of friction between 2kg block is μ=0.1. A constant horizontal force F=4N is applied on 1kg block. If the work done by the friction on 1kg block in 2s is −XJ, then find X. Take g=10m/s2.
View Solution
Knowledge Check
A block of mass 2 kg is placed on the floor. The coefficient of static friction is 0.4. A force F of 2.5 N is applied on the block as shown in Fig. 15.4.4. The force of friction between the block and the floor is (g=9.8m/s2)
 A1.5 NB0.5 NC2.5 NDNone of the above
A1.5 NB0.5 NC2.5 NDNone of the aboveA block of mass 2 kg is placed on the floor. The coefficient of static friction is 0.4. A force F of 3 N is applied on the block as shown in figure. The force of friction between the block and the floor is (Take g=10ms−2)
 A3 NB8 NC4 ND6 N
A3 NB8 NC4 ND6 N
In the diagram shown coefficient of friction between 2 kg block and 4 kg block is μ=0.1 and between 4 kg and ground is μ=0.2 then answer the following quwstions .(tis time)
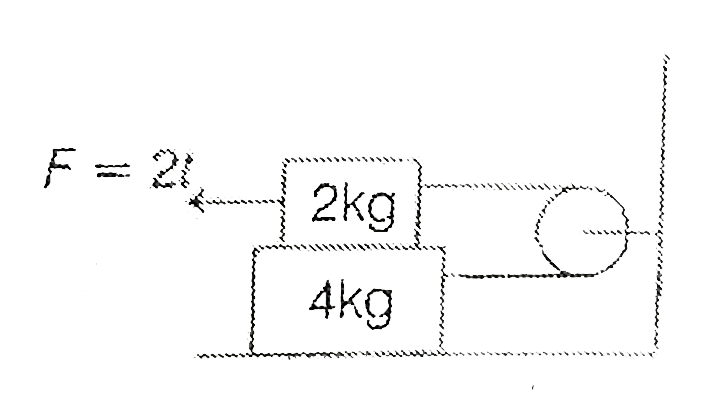
Force of friction on 2 kg block at t=12 s will be
View SolutionA block of mass 2 kg is placed on the floor . The coefficient of static friction is 0.4 . If a force of 2.8 N is applied on the block parallel to floor , the force of friction between the block and floor is : (Taking g = 10m/s2)
View SolutionIf coeffiecient of friction between the block of mass 2kg and table is 0.4 then out acceleration of the system and tension in the string. (g=10m/s2)
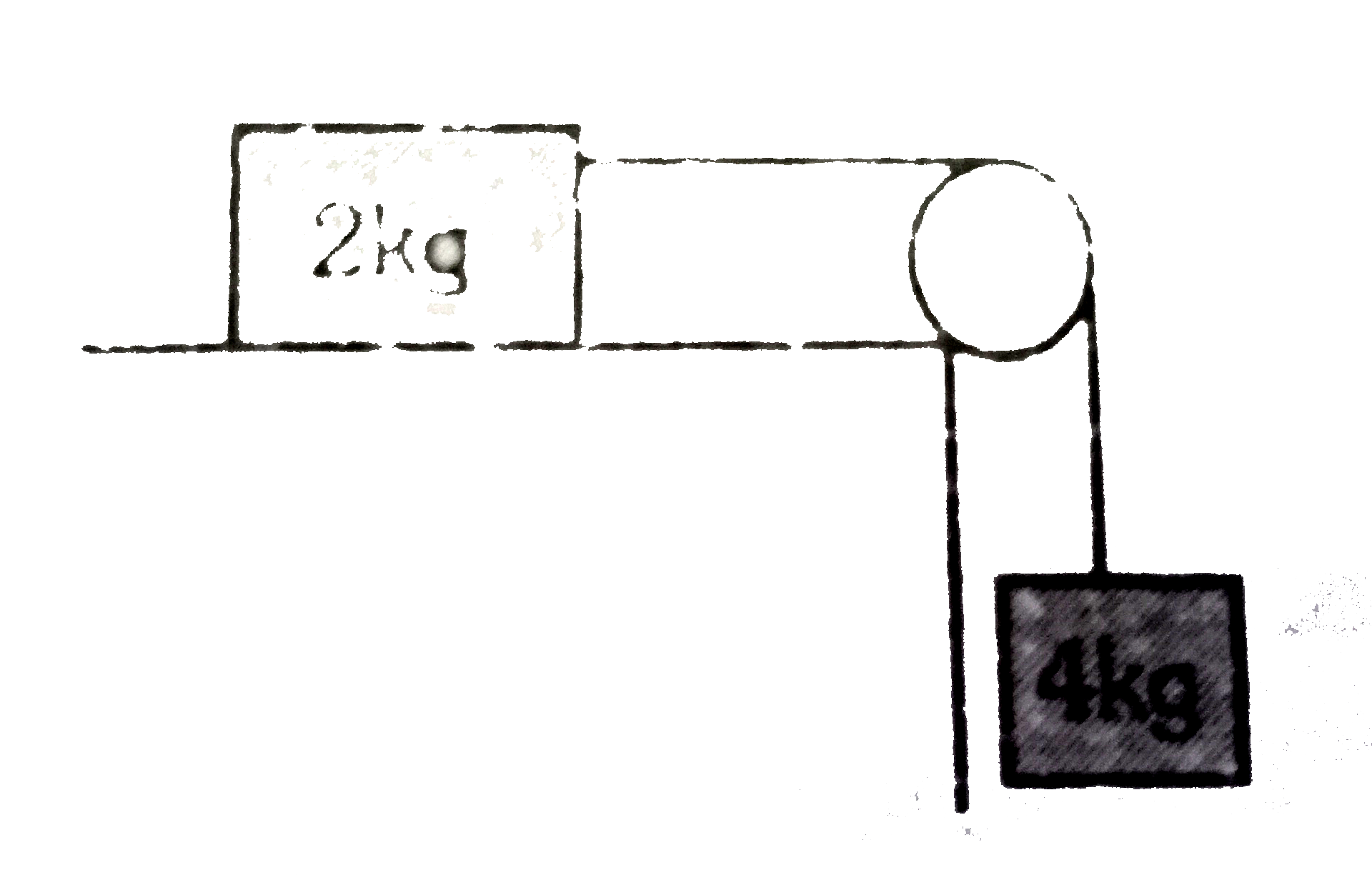
View SolutionA 2kg block is kept over a ground with coefficient of friction μ=0.8 as shown in figure. A time varying force F=2t(F in newton and t in second) is applied on the block. Plot a graph between acceleration of block versus time (g=10m/s2)

View SolutionCoefficient of friction between 5kg and 10 kg block is 0.5 and that between floor and 10 kg block is 0.8 if friction between floor and 10 kg block is 20 N what is the value of force (in N) being applied on 5 kg
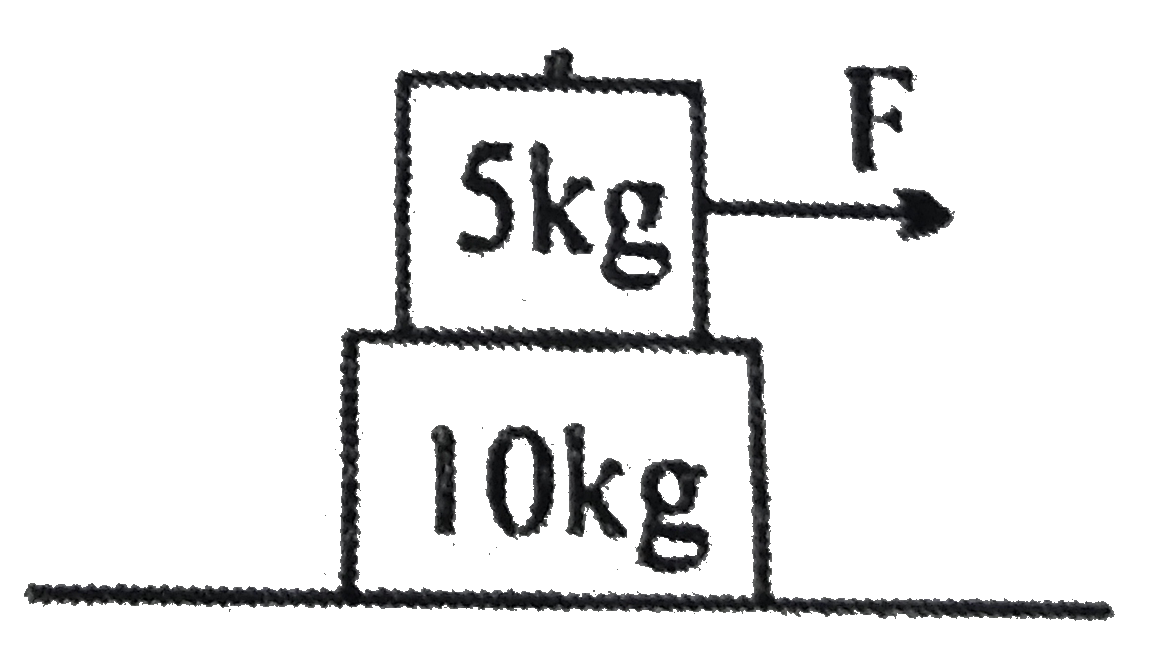
View SolutionA slab of 40kg lying on a frictionales floor and a block of 10 kg rests on the slab as shown in the figure. The coefficients of static and kinetic friction between the slab and the block are 0.6 and 0.4 respectively. If a force of 100N is applied on the block, then the acceleration the slab will be (g=10m/s2)
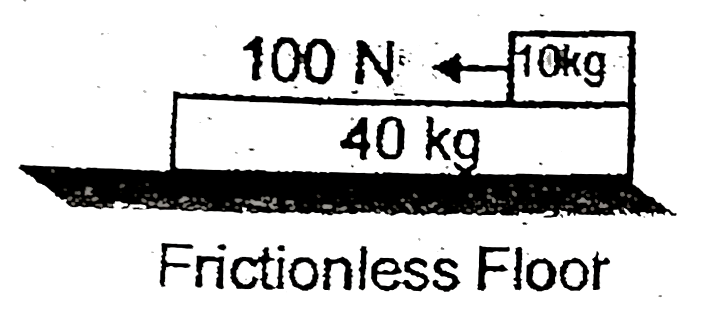
View Solution
