A 2kg block is kept over a ground with coefficient of friction μ=0.8 as shown in figure. A time varying force F=2t(F in newton and t in second) is applied on the block. Plot a graph between acceleration of block versus time (g=10m/s2)

fmax=μN=μmg
=0.8×2×10=16N
Block will not move till driving force F=2t becomes 16N. This force becomes 16N in 8s
For t≤8s
a=0
For t≥8s
a=F−fmaxm=2t−162⇒a=t−8
i.e. a−t graph is a straight line of slop +1 and intercept−8
Corresponding a−t graph is shown bellow 
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Objective Question
32 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Comprehension
9 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assertion And Reason
28 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
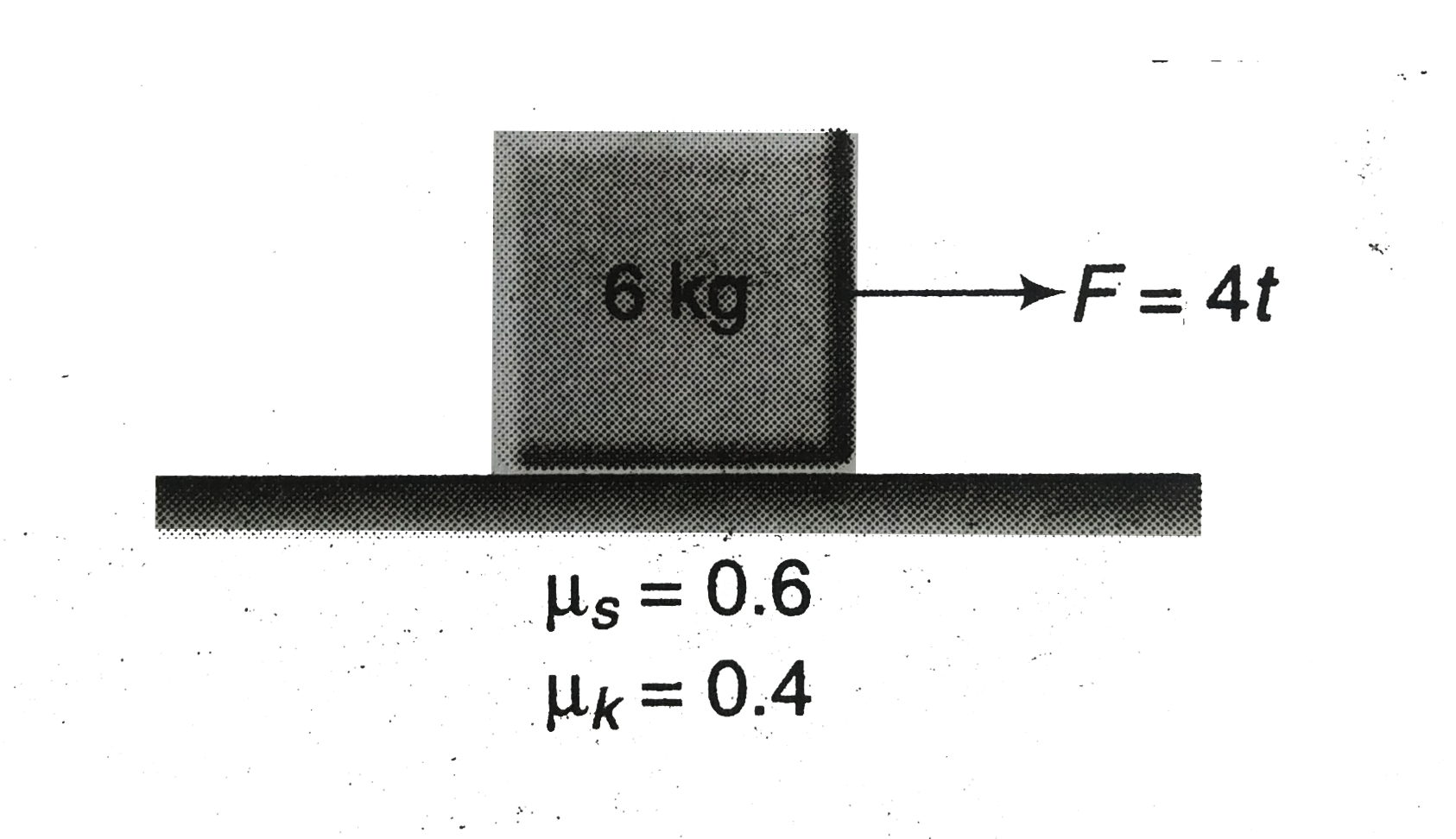
A 6kg block is kept over a rough surface with coefficient of friction μs=0.6 and μk=0.4 as shown in figure.A time varying force F=4t(F in newton and t in second) is applied on the block as shown. Plot a graph between acceleration of block and time (g=10m/s2)
View SolutionIn the given diagram, the block of mass 2 kg and 3 kg are placed one over the other . The contact surfaces are rough with coefficient of friction μ1=0.2,μ92)=0.06) . A force F=12t N ( where t is in second ) is applied on upper block in the direction ( Given that g = 10m/s2)
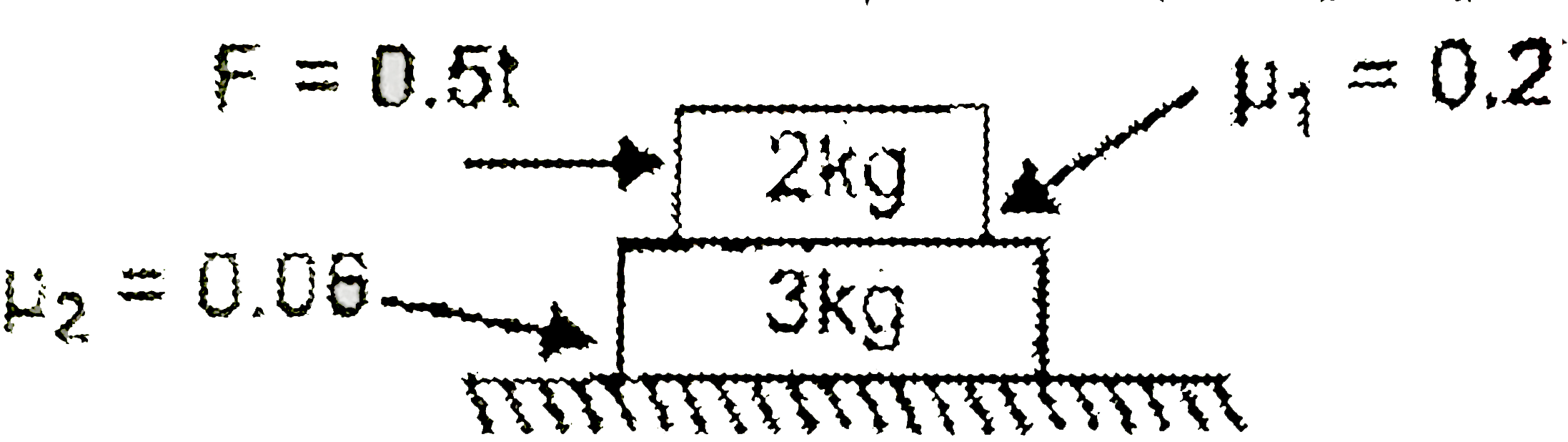
The acceleration time graph for 4 kg block is : Friction force acting between the two blocks at t=8 s
View SolutionIn the given diagram, the block of mass 2 kg and 3 kg are placed one over the other . The contact surfaces are rough with coefficient of friction μ1=0.2,μ92)=0.06) . A force F=12t N ( where t is in second ) is applied on upper block in the direction ( Given that g = 10m/s2)
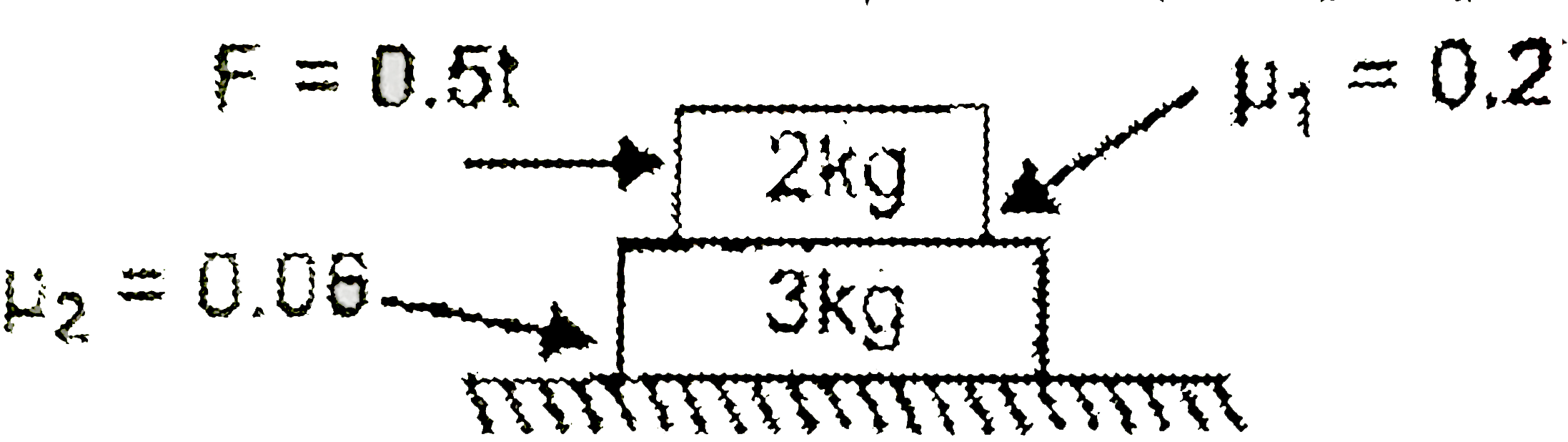
The acceleration time graph for 4 kg block is : The relative slipping between the blocks occurs at t =
View SolutionIn the given diagram, the block of mass 2 kg and 3 kg are placed one over the other . The contact surfaces are rough with coefficient of friction μ1=0.2,μ92)=0.06) . A force F=12t N ( where t is in second ) is applied on upper block in the direction ( Given that g = 10m/s2)
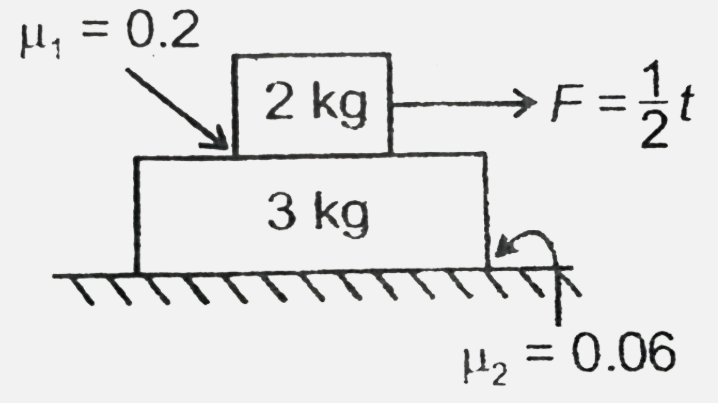
The acceleration time graph for 3 kg block is
View Solution
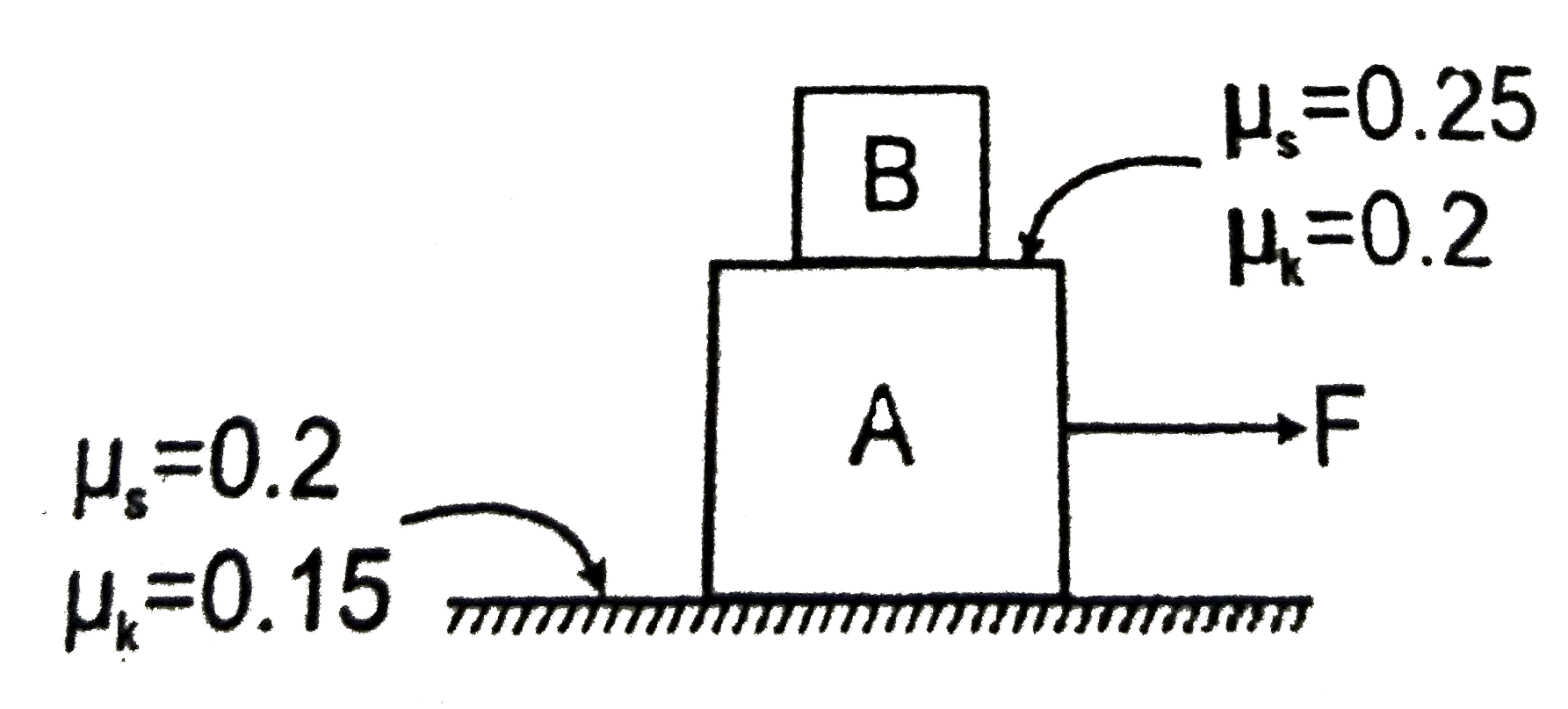
Block B of mass 2 kg rests on block A of mass 10 kg. All surfaces are rough with the value of coefficient of friction as shown in the figure. Find the minimum force F that should ber appplied on blocks A to cause relative motion between A and B.(g=10ms2)
View Solution
Block B of mass 2 kg rests on block A of mass 10 kg. All surfaces are rough with the value of coefficient of friction as shown in the figure. Find the minimum force F that should ber appplied on blocks A to cause relative motion between A and B.(g=10ms2)
View SolutionBlock B of mass 2kg rests on block A of mass 10kg. All surfaces are rought with the value of coefficient of friction as shown in the figure. Find the minimum force F that should be applied on block A to cause relative motion between A and B. (g=10m/s2)
View SolutionA block of mass m is placed on another block of mass M lying on a smooth horizontal surface as shown in the figure. The co-efficient of friction between the blocks is μ. Find the maximum horizontal force F (in Newton) that can be applied to the block M so that the blocks together with same acceleration? (M=3kg,m=2kg,μ=0.1,g=10m/s2)

View SolutionA block of mass 2 kg is at rest on a floor . The coefficient of static friction between block and the floor is 0.54. A horizonatl force of 2.8 N is applied to the block . What should be the frictional force between the block and the floor ? ( take , g=10m/s2)
View SolutionThe coefficient of static friction between the two blocks shown in figure is μ and the table is smooth. What maximum horizontal forced F can be applied to he block of mass M so that the block move together?

View Solution
