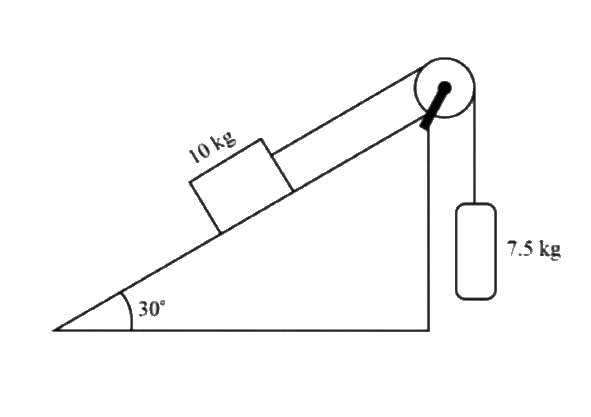
The linear mass density , i.e mass per unit length of the rope as shown in figure , varies 0 to λ from one end to another . The acceleration of the combined system of figure will be
F(M+λL2)
F(M+λL)
F(M+2λL)
F(M2+λL)
The correct Answer is:A
Step by step video & image solution for The linear mass density , i.e mass per unit length of the rope as shown in figure , varies 0 to lambda from one end to another . The acceleration of the combined system of figure will be by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Example
24 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE
69 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - ASSIGNMENT ( SECTION -I ) Subjective Type Questions
9 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
Similar Questions
Knowledge Check
In the system shown in the figure, the acceleration of 1 kg mass is
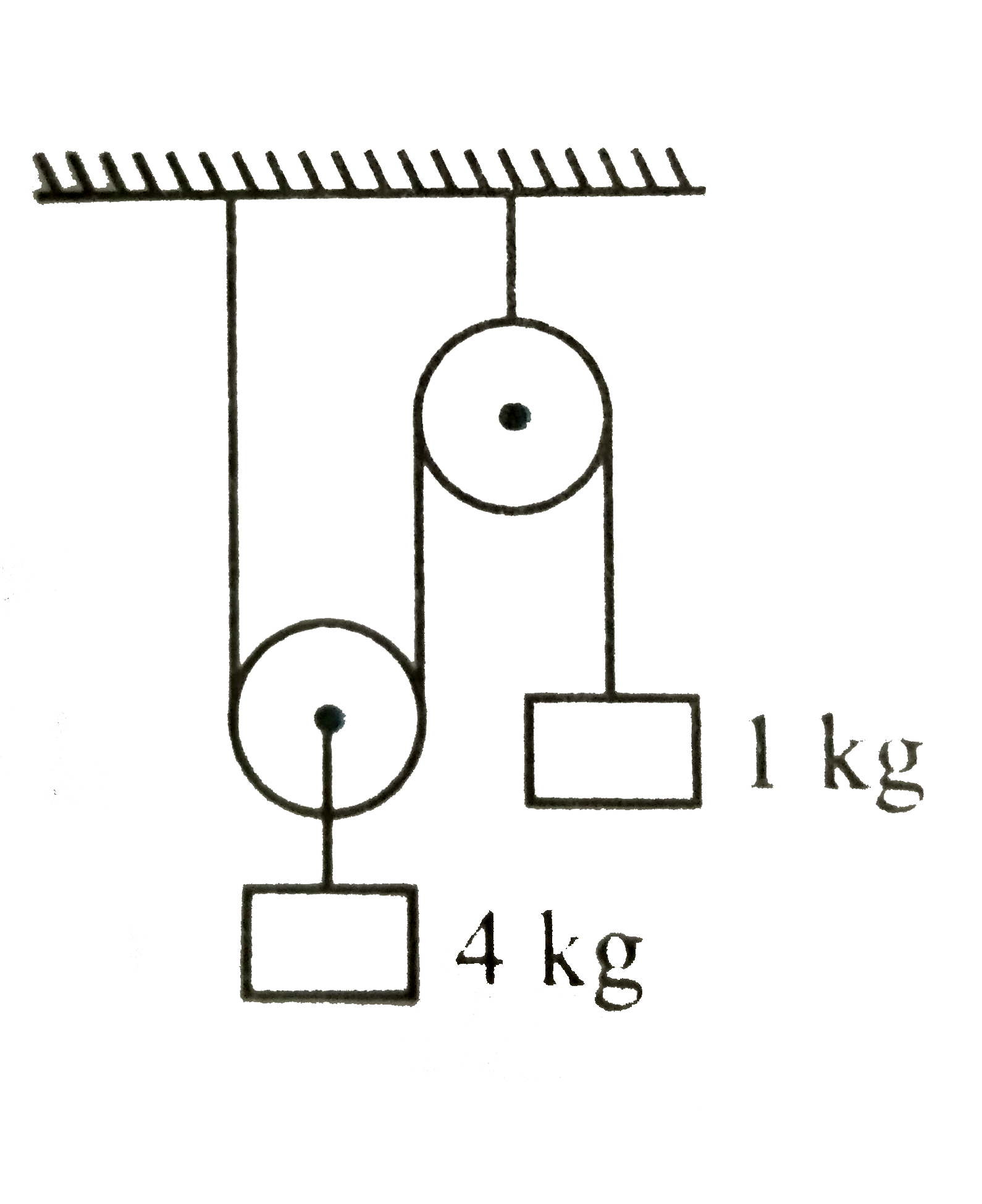 Ag4downwardsBg2downwardsCg2upwardsDg4upwards
Ag4downwardsBg2downwardsCg2upwardsDg4upwards
If linear charge density of a wire as shown in the figure is λ

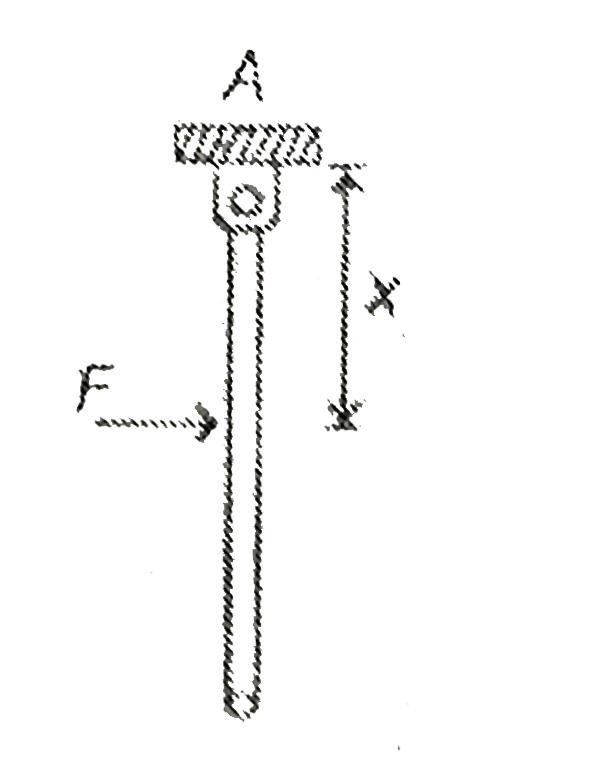
View SolutionA rod of mass m and length l is hinged at one of its ends A as shown in figure. A force F is applied at a distance x from A . The acceleration of centre of mass varies with x as −
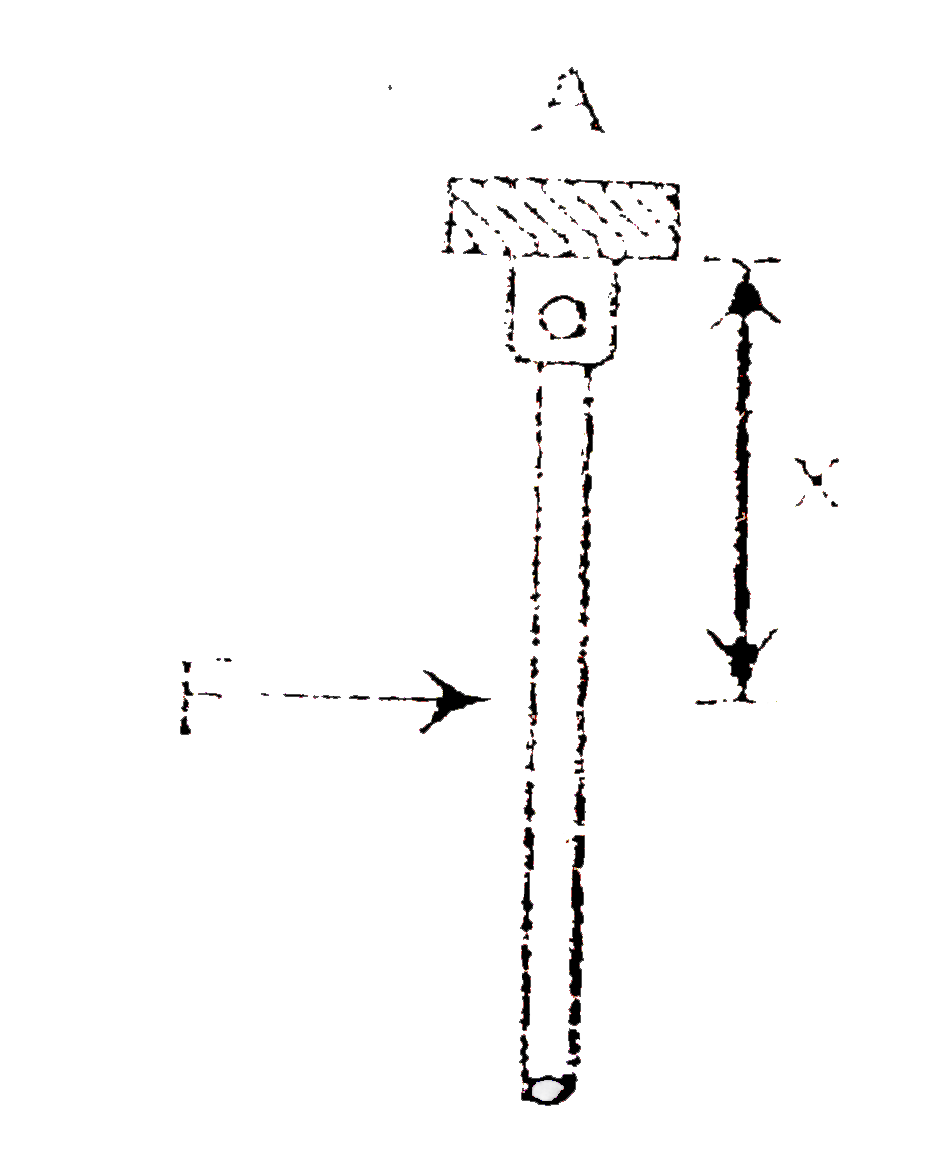
View SolutionA rod of mass m and length l is hinged at one of its ends A as shown in figure. A force F is applied at a distance x from A . The acceleration of centre of mass varies with x as −
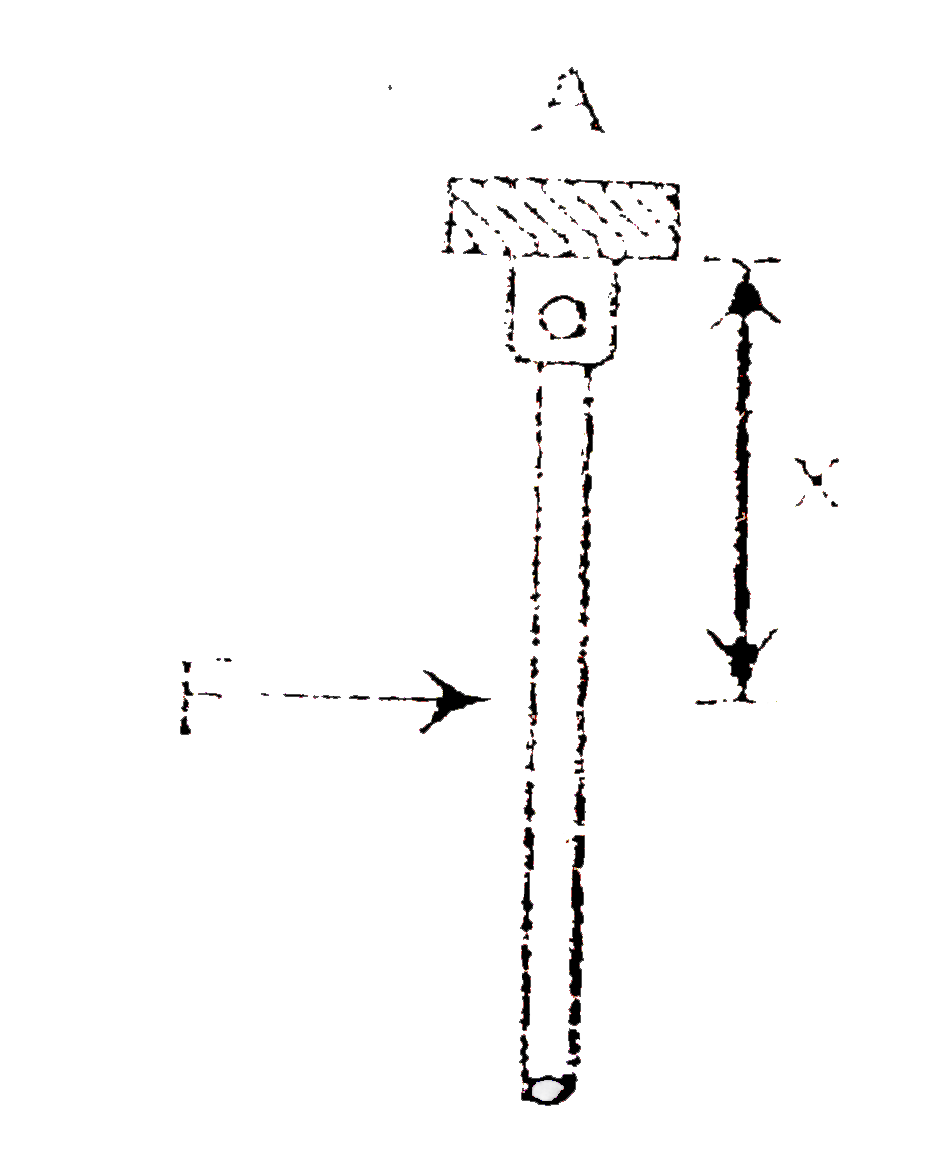
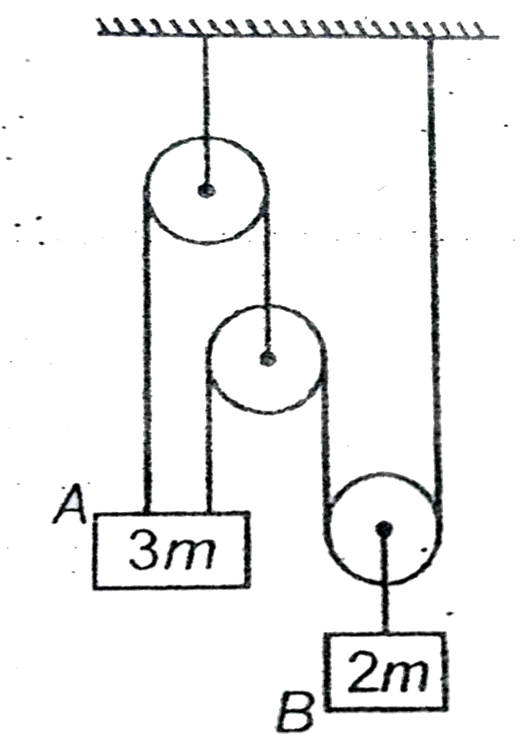
View SolutionConsider the system shown in figure
Mass of block A= 3m ,
Mass of block B= 2m .
Find the acceleration of A and B.
View SolutionThe co-ordinates of the centre of mass of the system as shown in figure are
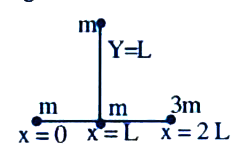
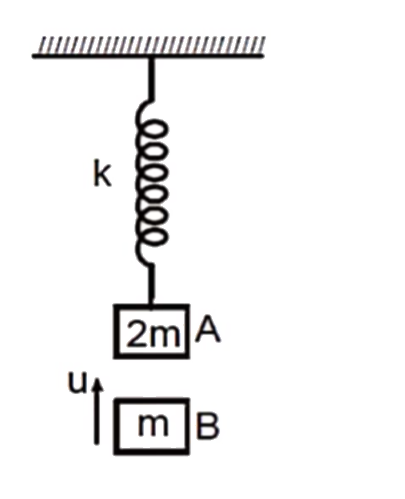
View SolutionA block A of mass 2m is hanging from a vertical massless spring of spring constant k and is in equilibrium. Another block B of mass m strikes the block A with velocity u and sticks to it as shown in the figure. The magnitude of the acceleration of the combined system of the blocks just after the collision is
View SolutionThe expansion of unit mass of a perfect gas at constant pressure as shown in the figure, then b and a
View Solution