Question

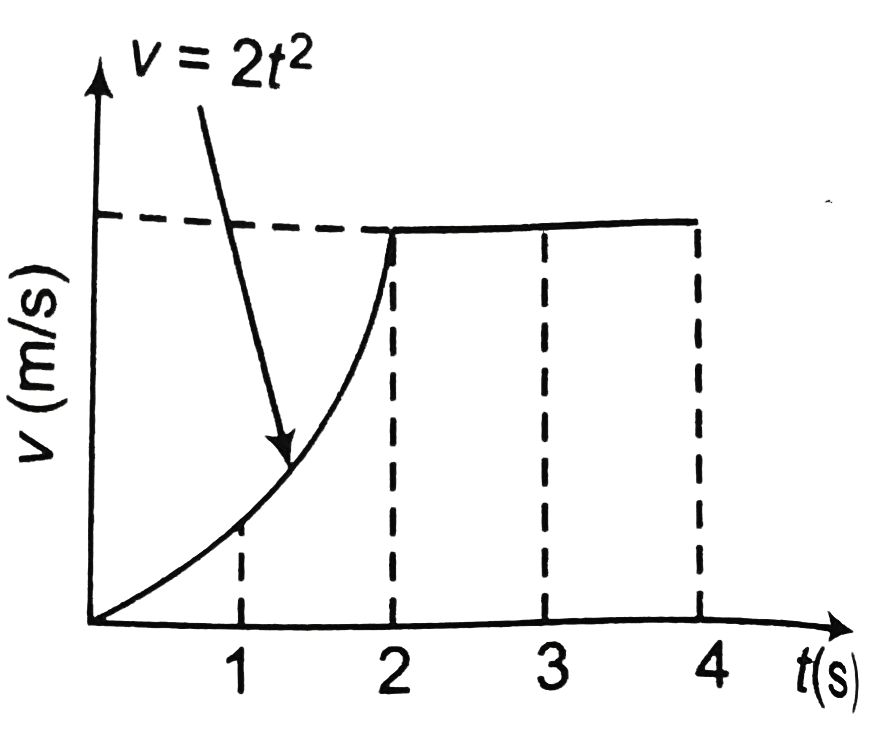
A long plank begins to move at $t=0$ and accelerates along a straight track wit a speed given by $v=2t^2$ for $0\le t\le 2$ (where $v$ is in m/s and $t$ is in second). After 2 sec the plane continues to move at the constant speed acquired. A small initially at rest on the plank begins to slip at $t=1$ sec and stops sliding at $t=3$ sec. If the coefficient of static friction and kinetic friction between the plank and the block is $0.s$ and $0.k$ (where $s$ and $k$ are digits) respectively, find $s+k$ (take $g=10m/s^2$)

Answer

` mu_(s) = 0.4 , mu_(k) = 0.3`

Explanation

To solve the problem, we will follow these steps:

Step 1: Determine the velocity of the plank at t = 2 seconds
The velocity of the plank is given by the equation:
\( v = 2t^2 \)
At \( t = 2 \) seconds:
\( v = 2(2^2) = 2 \times 4 = 8 \, \text{m/s} \)

Step 2: Calculate the acceleration of the plank at t = 1 second
To find the acceleration, we differentiate the velocity with respect to time:
\( a = \frac{dv}{dt} = \frac{d(2t^2)}{dt} = 4t \)
At \( t = 1 \) second:
\( a = 4(1) = 4 \, \text{m/s}^2 \)

Step 3: Determine the static friction coefficient (μs)
At \( t = 1 \) second, the block is about to slip. The force of static friction can be expressed as:
\( f_s = \mu_s N \)
Where \( N \) is the normal force, which equals \( mg \) (mass times gravitational acceleration). Therefore:
\( \mu_s mg = ma \)
Cancelling mass \( m \) from both sides:
\( \mu_s g = a \)
Substituting \( g = 10 \, \text{m/s}^2 \) and \( a = 4 \, \text{m/s}^2 \):
\( \mu_s \cdot 10 = 4 \)
\( \mu_s = \frac{4}{10} = 0.4 \)

Step 4: Calculate the average acceleration from t = 1 to t = 3 seconds
At \( t = 3 \) seconds, the velocity of the plank is still 8 m/s (constant speed after t = 2 seconds). The initial velocity at \( t = 1 \) second can be calculated as:
\( v(1) = 2(1^2) = 2 \, \text{m/s} \)
The average acceleration \( a_{avg} \) from \( t = 1 \) to \( t = 3 \) seconds is:
\( a_{avg} = \frac{v_f - v_i}{t_f - t_i} = \frac{8 - 2}{3 - 1} = \frac{6}{2} = 3 \, \text{m/s}^2 \)

Step 5: Determine the kinetic friction coefficient (μk)
Now we apply the same formula for kinetic friction:
\( f_k = \mu_k N \)
Where \( N = mg \). Thus:
\( \mu_k mg = ma_{avg} \)
Cancelling mass \( m \):
\( \mu_k g = a_{avg} \)
Substituting \( g = 10 \, \text{m/s}^2 \) and \( a_{avg} = 3 \, \text{m/s}^2 \):
\( \mu_k \cdot 10 = 3 \)
\( \mu_k = \frac{3}{10} = 0.3 \)

Step 6: Find the sum of s and k
From our calculations:
- \( s = 4 \) (from \( \mu_s = 0.4 \))
- \( k = 3 \) (from \( \mu_k = 0.3 \))

Thus:
\( s + k = 4 + 3 = 7 \)

Final Answer
The value of \( s + k \) is \( 7 \).

---