We write the relation for Boyle's law in the form, PV=KB when the temperature remains constant. In this relation, the magnitude of KB depends upon the
The nature of the gas used in the experiment
the molecular mass of gas in the laboratory
the atmospheric pressure
the quantity of gas enclosed
The correct Answer is:D
To solve the question regarding Boyle's law and the relation PV=KB, we can follow these steps:
Step 1: Understand Boyle's Law
Boyle's Law states that for a given mass of gas at constant temperature, the product of pressure (P) and volume (V) is a constant. This can be expressed mathematically as:
PV=KB
where KB is a constant for a specific amount of gas at a constant temperature.
Step 2: Relate to the Ideal Gas Law
The Ideal Gas Law is given by the equation:
PV=nRT
where:
- P = pressure of the gas
- V = volume of the gas
- n = number of moles of the gas
- R = universal gas constant
- T = absolute temperature
Step 3: Compare the Two Equations
From Boyle's Law, we have:
PV=KB
From the Ideal Gas Law, at constant temperature, we can rewrite it as:
PV=nRT
Since both equations equal PV, we can set them equal to each other:
KB=nRT
Step 4: Analyze the Dependence of KB
In the equation KB=nRT, we see that KB depends on:
- n (the number of moles of gas)
- R (the gas constant, which is a constant)
- T (the absolute temperature, which is constant in this case)
Since R and T are constants, the magnitude of KB is directly proportional to n, which is the number of moles of the gas.
Conclusion
Thus, the magnitude of KB depends upon the number of moles of the gas, which can also be related to the mass of the gas if the molecular mass is known.
Final Answer
The magnitude of KB depends upon the number of moles of the gas (or the mass of the gas).
---
Boyle's Law states that for a given mass of gas at constant temperature, the product of pressure (P) and volume (V) is a constant. This can be expressed mathematically as:
PV=KB
where KB is a constant for a specific amount of gas at a constant temperature.
The Ideal Gas Law is given by the equation:
PV=nRT
where:
- P = pressure of the gas
- V = volume of the gas
- n = number of moles of the gas
- R = universal gas constant
- T = absolute temperature
From Boyle's Law, we have:
PV=KB
From the Ideal Gas Law, at constant temperature, we can rewrite it as:
PV=nRT
Since both equations equal PV, we can set them equal to each other:
KB=nRT
In the equation KB=nRT, we see that KB depends on:
- n (the number of moles of gas)
- R (the gas constant, which is a constant)
- T (the absolute temperature, which is constant in this case)
Thus, the magnitude of KB depends upon the number of moles of the gas, which can also be related to the mass of the gas if the molecular mass is known.
The magnitude of KB depends upon the number of moles of the gas (or the mass of the gas).
Topper's Solved these Questions
- KINETIC THEORYBook:AAKASH INSTITUTE ENGLISHChapter:KINETIC THEORYExercise:Assignment (Section-B) Objective type questions (One option is correct)
Explore 10 Videos
- KINETIC THEORYBook:AAKASH INSTITUTE ENGLISHChapter:KINETIC THEORYExercise:Assignment (Section-C) Objective type questions (More than one option are correct)
Explore 9 Videos
- KINETIC THEORYBook:AAKASH INSTITUTE ENGLISHChapter:KINETIC THEORYExercise:Try Yourself
Explore 43 Videos
- GRAVITATION Book:AAKASH INSTITUTE ENGLISHChapter:GRAVITATION Exercise:ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
Explore 15 Videos
- LAWS OF MOTIONBook:AAKASH INSTITUTE ENGLISHChapter:LAWS OF MOTIONExercise:Assignment (SECTION-D) (Assertion-Reason Type Questions)
Explore 15 Videos
Similar Questions
Correct the statements :
Boyle's law relates volume with temperature.
View SolutionWrite down the relation connecting the pressure and temperature of a gas if volume is kept constant.
View SolutionWrite the identity relation on set A={a, b, c} .
View SolutionIn the figure shown find relation between magnitudes of aA and aB.
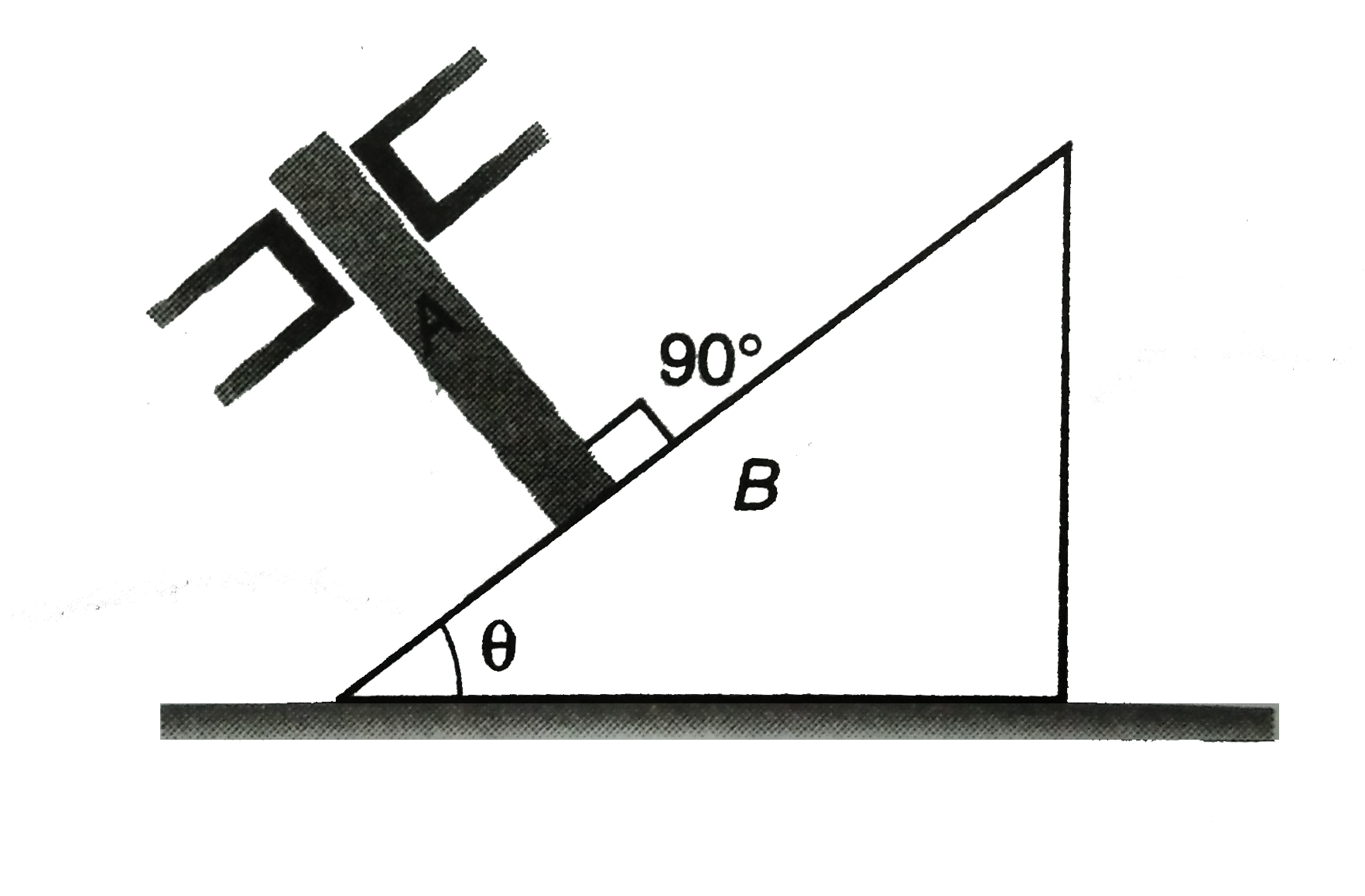
View SolutionIn the figure shown find relation between magnitudes of aA and aB.

View SolutionWhile stating Boyle's law why should we use the phrase " at constant temperature"?
View SolutionA : Increasing the concentration of H2 will increases
magnitude of equilibrium constant of the reaction
H2+I2⇔2HI
R: Value of Kc depends upon the concentration of reactants and products taken.
View SolutionWhen air is pumped in a football bladder, both volume and pressure increase simultaneously whereas the temperature remains constant. Is it not a violation of the Boyle's law?
View SolutionA : At higher temperature , Kw of water remains unaltered.
R: kw is a constant.
View SolutionWrite expressions for Boyle temperature and inversion temperature of a gas in terms of van der Waals constants. How are the two related to each other ?
View Solution