A particle of mass m carries an electric positive charge Q and is subjected to the combined action of gravity g and a uniform horizontal electric field of strength E,it is projected with speed u to the vertical plane parallel to the electrical field and at an angle θto the horizontal .The maximum distance the particle travels horizontally when it is at the same level as its starting point is
To solve the problem of finding the maximum horizontal distance traveled by a charged particle projected in a uniform electric field and under the influence of gravity, we can follow these steps:
Step 1: Analyze the Motion
The particle is projected with an initial speed u at an angle θ to the horizontal. We can resolve this initial velocity into horizontal and vertical components:
- Horizontal component: ux=ucosθ
- Vertical component: uy=usinθ
Step 2: Determine the Forces Acting on the Particle
The forces acting on the particle are:
1. The gravitational force Fg=mg acting downward.
2. The electric force Fe=QE acting horizontally (to the right, assuming the electric field is directed to the right).
The acceleration due to gravity is g downward, and the acceleration due to the electric field is ae=QEm horizontally.
Step 3: Write the Equations of Motion
For horizontal motion:
x(t)=uxt+12aet2=ucosθ⋅t+12(QEm)t2
For vertical motion:
y(t)=uyt−12gt2=usinθ⋅t−12gt2
Step 4: Find the Time of Flight
The particle returns to the same vertical level when y(t)=0:
usinθ⋅t−12gt2=0
Factoring out t:
t(usinθ−12gt)=0
This gives t=0 (initial time) or:
t=2usinθg
Step 5: Substitute Time into the Horizontal Motion Equation
Now we substitute t into the horizontal motion equation:
xmax=ucosθ⋅(2usinθg)+12(QEm)(2usinθg)2
Calculating the first term:
xmax=2u2sinθcosθg
Using the identity sin2θ=2sinθcosθ:
xmax=u2sin2θg
Calculating the second term:
12(QEm)(4u2sin2θg2)=2QEu2sin2θmg2
Step 6: Combine the Terms
Thus, the total horizontal distance is:
xmax=u2sin2θg+2QEu2sin2θmg2
Step 7: Final Expression
Combining both terms, we have:
xmax=u2g(sin2θ+2QEsin2θmg)
Conclusion
The maximum horizontal distance the particle travels when it is at the same level as its starting point is:
xmax=u2g(sin2θ+2QEsin2θmg)
The particle is projected with an initial speed u at an angle θ to the horizontal. We can resolve this initial velocity into horizontal and vertical components:
- Horizontal component: ux=ucosθ
- Vertical component: uy=usinθ
The forces acting on the particle are:
1. The gravitational force Fg=mg acting downward.
2. The electric force Fe=QE acting horizontally (to the right, assuming the electric field is directed to the right).
For horizontal motion:
x(t)=uxt+12aet2=ucosθ⋅t+12(QEm)t2
y(t)=uyt−12gt2=usinθ⋅t−12gt2
The particle returns to the same vertical level when y(t)=0:
usinθ⋅t−12gt2=0
Factoring out t:
t(usinθ−12gt)=0
This gives t=0 (initial time) or:
t=2usinθg
Now we substitute t into the horizontal motion equation:
xmax=ucosθ⋅(2usinθg)+12(QEm)(2usinθg)2
Calculating the first term:
xmax=2u2sinθcosθg
Using the identity sin2θ=2sinθcosθ:
xmax=u2sin2θg
12(QEm)(4u2sin2θg2)=2QEu2sin2θmg2
Thus, the total horizontal distance is:
xmax=u2sin2θg+2QEu2sin2θmg2
Combining both terms, we have:
xmax=u2g(sin2θ+2QEsin2θmg)
The maximum horizontal distance the particle travels when it is at the same level as its starting point is:
xmax=u2g(sin2θ+2QEsin2θmg)
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - comprehension
3 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION-I(SUBJECTIVE TYPE QUESTIONS)
2 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT(SECTION -D) Assertion-Reason type Question)
15 Videos
Similar Questions
Knowledge Check
A particle is thrown with a speed is at an angle θ with the horizontal. When the particle makes an angle ϕ with the horizontal, its speed changes to v, then
Av=v0cosθBv=v0cosθ⋅cosϕCv=v0cosθ⋅secϕDv=v0secθ⋅cosϕ
A particle is projected horizontally with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?

View SolutionConsider a finite charged rod. Electric field at point P (shown) makes an angle θ with horizontal dotted line then angle θ is:-
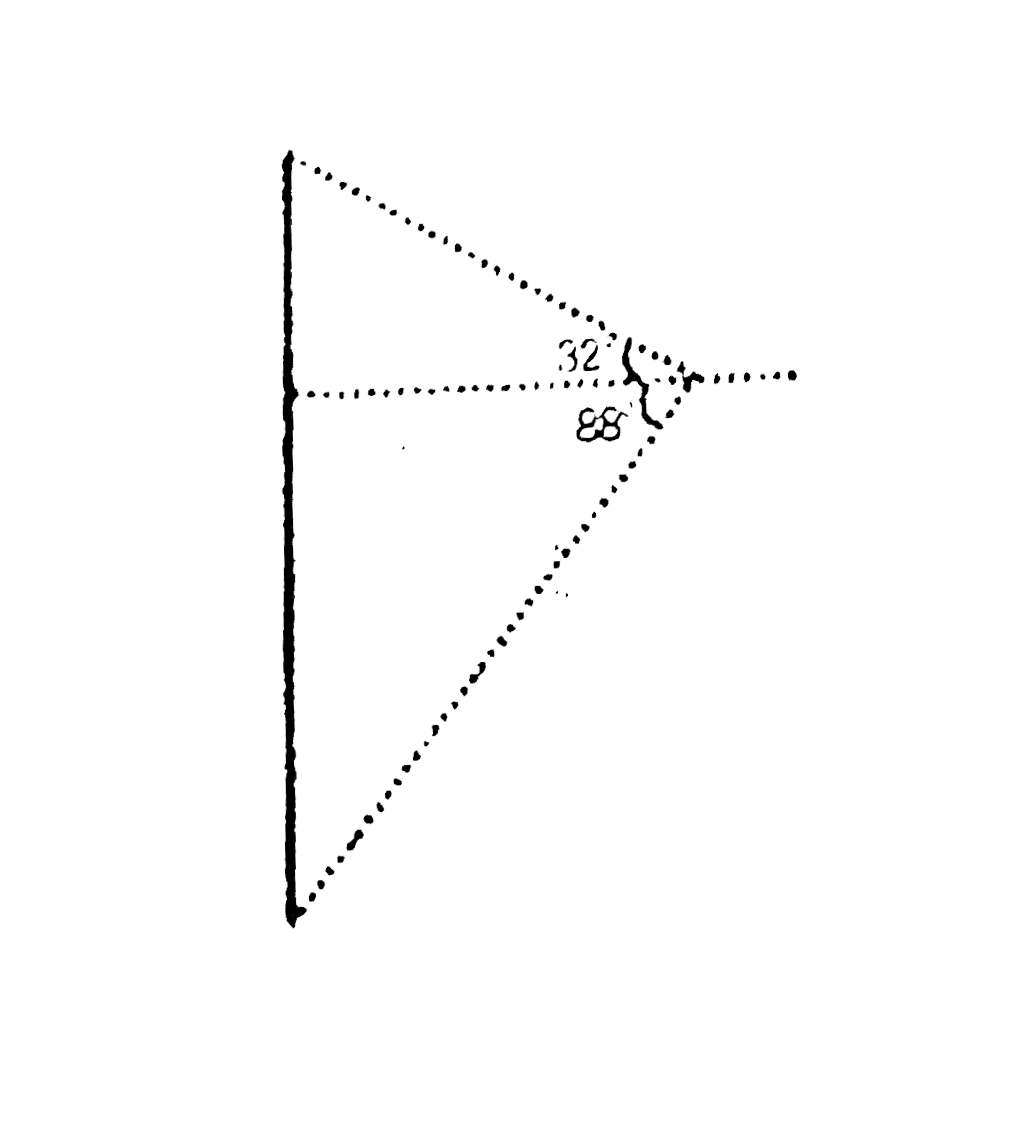
View SolutionA pendulum bob of mass m charge q is at rest with its string making an angle θ with the vertical in a uniform horizontal electric field E. The tension in the string in
View SolutionA particle of mass m is projected at an angle α to the horizontal with an initial velocity u. The work done by gravity during the time it reaches its highest point is
View SolutionThe path of a charged particle projected into a uniform transverse electric field is
View SolutionA particle is projected with speed u at angle θ to the horizontal. Find the radius of curvature at highest point of its trajectory
View SolutionA particle is projected up an inclines plane. Plane is inclined at an angle θ with horizontal and particle is projected at an angle α with horizontal. If particle strikes the plane horizontally prove that tanα=2tanθ
View Solution
