A particle of mass 1g and charge 2 C is released from a point A in a region of space containing electric field. Its speed is measured to be 2 m/s when it passes a point B some distance away from A. Potential difference the two points A and B is
1 mV
2mV
4 Mv
Both the points are a the same potentials
The correct Answer is:1
To find the potential difference between points A and B, we can follow these steps:
Step 1: Identify the given values
- Mass of the particle, m=1g=0.001kg
- Charge of the particle, q=2C
- Initial speed at point A, u=0m/s (released from rest)
- Final speed at point B, v=2m/s
Step 2: Use the kinematic equation
We will use the kinematic equation:
v2=u2+2as
Substituting the known values:
(2)2=(0)2+2as
This simplifies to:
4=2as
From this, we can express as:
as=2
Step 3: Express acceleration in terms of force
The force acting on the particle due to the electric field is given by:
F=qE
By Newton's second law, we also have:
F=ma
Setting these equal gives:
ma=qE
From this, we can express acceleration a:
a=qEm
Step 4: Substitute acceleration back into the equation
Substituting a back into the equation as=2:
(qEm)s=2
Thus, we can express s:
s=2mqE
Step 5: Calculate the work done
The work done by the electric field is equal to the change in potential energy, which can be expressed as:
ΔU=F⋅s=qEs
Substituting s:
ΔU=qE(2mqE)=2m
Step 6: Relate potential difference to change in potential energy
The potential difference ΔV is given by:
ΔV=ΔUq
Substituting ΔU:
ΔV=2mq
Step 7: Substitute the known values
Now substituting the values of m and q:
ΔV=2×0.001kg2C=0.0022=0.001V
Thus,
ΔV=1mV
Final Answer
The potential difference between points A and B is 1mV.
---
- Mass of the particle, m=1g=0.001kg
- Charge of the particle, q=2C
- Initial speed at point A, u=0m/s (released from rest)
- Final speed at point B, v=2m/s
We will use the kinematic equation:
v2=u2+2as
Substituting the known values:
(2)2=(0)2+2as
This simplifies to:
4=2as
From this, we can express as:
as=2
The force acting on the particle due to the electric field is given by:
F=qE
By Newton's second law, we also have:
F=ma
Setting these equal gives:
ma=qE
From this, we can express acceleration a:
a=qEm
Substituting a back into the equation as=2:
(qEm)s=2
Thus, we can express s:
s=2mqE
The work done by the electric field is equal to the change in potential energy, which can be expressed as:
ΔU=F⋅s=qEs
Substituting s:
ΔU=qE(2mqE)=2m
The potential difference ΔV is given by:
ΔV=ΔUq
Substituting ΔU:
ΔV=2mq
Now substituting the values of m and q:
ΔV=2×0.001kg2C=0.0022=0.001V
Thus,
ΔV=1mV
The potential difference between points A and B is 1mV.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - SECTION-B (OBJECTIVE TYPE QUESTIONS (ONLY ONE ANSWER) )
1 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - SECTION-B(OBJECTIVE TYPE QUESTIONS(ONLY ONE ANSWER) )
29 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - ASSIGNMENT SECTION - D
9 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions
25 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
15 Videos
Similar Questions
A particle of mass m and positive charge q is released from point A. Its speed is found to be v when it passes through a point B. which of the two points is at higher potential ? What is the potential difference between the points?
View SolutionTwo points A and B are located in a region of space containing electric field as shown in the figure. As we move from A to B, the potentia
View SolutionA charge particle of mass m and charge q is released from rest in uniform electric field. Its graph between velocity (v) and distance travelled (x) will be :
View SolutionA charge particle of mass m and charge q is released from rest in uniform electric field. Its graph between velocity (v) and distance travelled (x) will be :
View SolutionA particle of mass m is released from point A as shown in the gigure. Would it loop the loop if H=4r? What is the force on the circular track when it is at point (a) B, (b) C?
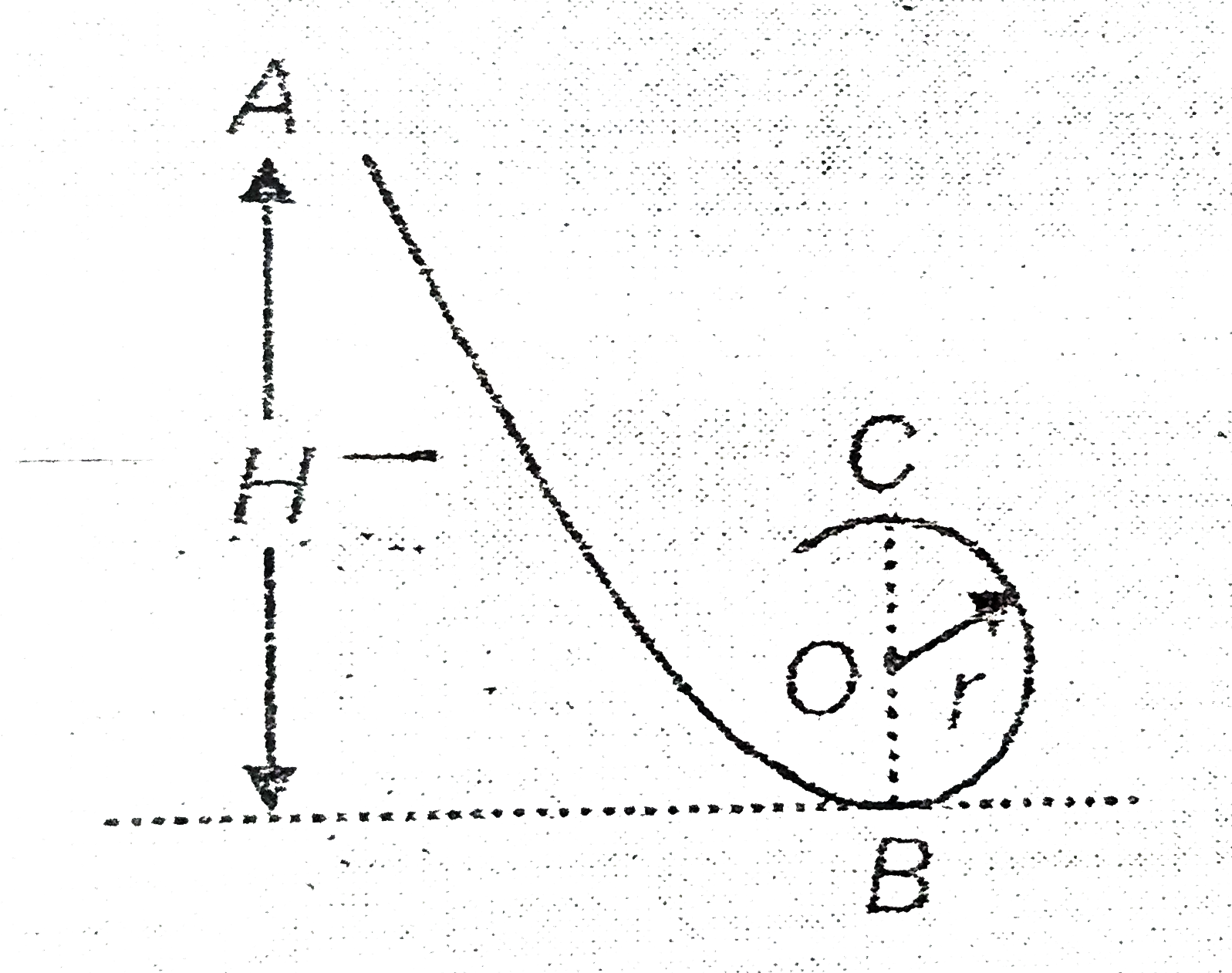
View SolutionA particle of mass 1 kg is released from rest at a point where gravitational potential is 5 J/kg. After some time, it passes through a point B with a speed of 1 m/s. What is the gravitational potential at B ?
View SolutionA point charge is taken from a point A to a pint B in an electric field. Does the work done by the electric field depend on the path of the charge ?
View SolutionA particle of mass m carrying charge q is projected with velocity v from point P toward aninfinite line of charge from a distance a . Its speed reduces to zero momentarily at point Q, which is at a distance a/2 from the line of charge. If another particle with mass m and charge −q is projected with the same velosity v from P toward the line of charge, what will be its speed at Q ?
View SolutionWhen a 2μC charge is carried from point A to point B, the amount of work done by the electric field is 50μJ. What is the potential difference and which point is at a higher potential ?
View SolutionTwo point charges, each of mass m and charge q are released when they are at a distance d from each other. What is the speed of each charged particle when they are at a distance 2r ?
View Solution
