A charge particle of mass m and charge q is released from rest in uniform electric field. Its graph between velocity (v) and distance travelled (x) will be :
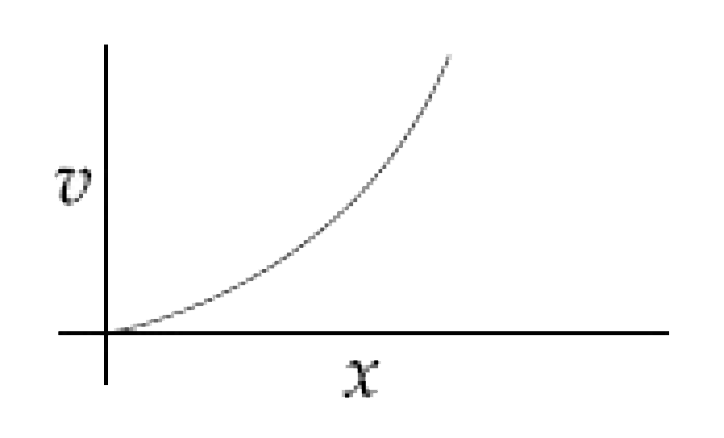

The correct Answer is:3
To solve the problem of how the velocity v of a charged particle changes with the distance x traveled in a uniform electric field, we can follow these steps:
1. Understanding the Forces:
A charged particle of mass m and charge q experiences a force F due to the electric field E. According to Newton's second law, this force can be expressed as:
F=ma
where a is the acceleration of the particle.
2. Relating Force to Electric Field:
The force acting on the particle due to the electric field is given by:
F=qE
By equating the two expressions for force, we have:
ma=qE
3. Finding Acceleration:
From the equation ma=qE, we can express the acceleration a as:
a=qEm
4. Using Kinematic Relations:
We know that acceleration can also be expressed in terms of velocity and distance:
a=vdvdx
Setting the two expressions for acceleration equal gives us:
vdvdx=qEm
5. Separating Variables:
Rearranging the equation, we can separate the variables:
vdv=qEmdx
6. Integrating Both Sides:
Now we integrate both sides. The left side integrates to:
∫vdv=v22
The right side integrates to:
∫qEmdx=qEmx
Thus, we have:
v22=qEmx+C
Since the particle is released from rest, when x=0, v=0, which gives C=0.
7. Final Equation:
Therefore, the equation simplifies to:
v2=2qEmx
8. Identifying the Graph:
The equation v2=2qEmx represents a parabolic relationship between v and x. This means that if we plot v on the y-axis and x on the x-axis, the graph will be a parabola opening to the right.
Conclusion
The graph between velocity v and distance x for a charged particle in a uniform electric field is a parabola.
A charged particle of mass m and charge q experiences a force F due to the electric field E. According to Newton's second law, this force can be expressed as:
F=ma
where a is the acceleration of the particle.
The force acting on the particle due to the electric field is given by:
F=qE
By equating the two expressions for force, we have:
ma=qE
From the equation ma=qE, we can express the acceleration a as:
a=qEm
We know that acceleration can also be expressed in terms of velocity and distance:
a=vdvdx
Setting the two expressions for acceleration equal gives us:
vdvdx=qEm
Rearranging the equation, we can separate the variables:
vdv=qEmdx
Now we integrate both sides. The left side integrates to:
∫vdv=v22
The right side integrates to:
∫qEmdx=qEmx
Thus, we have:
v22=qEmx+C
Since the particle is released from rest, when x=0, v=0, which gives C=0.
Therefore, the equation simplifies to:
v2=2qEmx
The equation v2=2qEmx represents a parabolic relationship between v and x. This means that if we plot v on the y-axis and x on the x-axis, the graph will be a parabola opening to the right.
The graph between velocity v and distance x for a charged particle in a uniform electric field is a parabola.
Topper's Solved these Questions
Similar Questions
A particle of mass m and charge −q is released from rest in a uniform electric field. If there is no other force on the particle, the dependence of its potential energy on the distance x travelled by it is correctly given by (assume initial potential energy to be zero ,graphs are schematic and not drawn to scale)
View SolutionA particle of mass m and charge −q is released from rest in a uniform electric field. If there is no other force on the particle, the dependence of its potential energy on the distance x travelled by it is correctly given by (assume initial potential energy to be zero ,graphs are schematic and not drawn to scale)
View SolutionA charge of mass .m. charge .2e. is released from rest in a uniform electric field of strength .E.. The time taken by it to travel a distance .d. in the field is
View SolutionA particle of mass and charge is released from rest in a uniform electric field. If there is no other force on the particle, the dependence of its kinetic energy on the distance travelled by it is correctly given by (graphs are schematic and not drawn to scale)
View SolutionA particle of mass and charge is released from rest in a uniform electric field. If there is no other force on the particle, the dependence of its kinetic energy on the distance travelled by it is correctly given by (graphs are schematic and not drawn to scale)
View SolutionA charged particle of mass m and charge q is released from rest in an electric field of constant magnitude E. The kinetic energy of the particle after time t is
View SolutionA particle of mass m and charge q is released from rest in uniform electric field of intensity E. Calculate the kinetic energy it attains after moving a distance y between the plates.
View SolutionA charged particle of mass m and charge q is released from rest the position (x0,0) in a uniform electric field E0ˆj. The angular momentum of the particle about origin.
View SolutionA particle of mass ma and charge q is released from rest in a uniform filed of magnitude E. the uniform field is created between two parallel plates of charge densities +σ and −σ, respectively. The particle accelerates toward the other plate a distance d apart. Determine the speed at which it stirkes the opposite plate.

View SolutionA particle of mass m and charge q is placed at rest in a uniform electric field E and then released, the kinetic energy attained by the particle after moving a distance y will be
View Solution