STATEMENT-1 : At a point electrostatic field is zero, then potential at that point may be zero .
and
STATEMENT-2 : Negative of the potential gradient is equal to electrostatic field.
Statement-1 is True, Statement-2 is True, Statement-2 is a correct explanation for Statement-1
Statement-1 is True, Statement-2 is True, Statement-2 is NOT a correct explanation for Statement-1
Statement-1 is True, Statement-2 is False
Statement-1 is False , Statement-2 is True
The correct Answer is:2
To analyze the given statements, we will break down the reasoning step by step.
Step 1: Understand Statement 1
Statement 1: At a point where the electric field is zero, the potential at that point may be zero.
- The electric field (E) is related to the electric potential (V) by the equation:
E=−dVdr
- If the electric field (E) is zero at a point, it implies:
−dVdr=0
- This means that the derivative of the potential with respect to distance is zero, indicating that the potential (V) could be constant.
Step 2: Analyze the Implications of the Derivative Being Zero
- If the derivative of V is zero, it means that V does not change with respect to r. Therefore, V could be:
- A constant value (which could be any number, including zero).
- Specifically, V could be zero, but it could also be some other constant value (like 5 volts, 10 volts, etc.).
Conclusion for Statement 1
- Thus, Statement 1 is true because the potential at that point can indeed be zero, but it is not necessarily zero; it could be any constant value.
Step 3: Understand Statement 2
Statement 2: The negative of the potential gradient is equal to the electrostatic field.
- The potential gradient is defined as:
Potential Gradient=dVdr
- According to the relationship between electric field and potential:
E=−dVdr
- This confirms that the negative of the potential gradient is indeed equal to the electric field.
Conclusion for Statement 2
- Therefore, Statement 2 is also true.
Final Conclusion
- Both statements are true, and Statement 2 serves as an explanation for Statement 1. Thus, the correct answer is that both statements are true.
---
Statement 1: At a point where the electric field is zero, the potential at that point may be zero.
E=−dVdr
- If the electric field (E) is zero at a point, it implies:
−dVdr=0
- This means that the derivative of the potential with respect to distance is zero, indicating that the potential (V) could be constant.
- If the derivative of V is zero, it means that V does not change with respect to r. Therefore, V could be:
- A constant value (which could be any number, including zero).
- Specifically, V could be zero, but it could also be some other constant value (like 5 volts, 10 volts, etc.).
- Thus, Statement 1 is true because the potential at that point can indeed be zero, but it is not necessarily zero; it could be any constant value.
Statement 2: The negative of the potential gradient is equal to the electrostatic field.
Potential Gradient=dVdr
- According to the relationship between electric field and potential:
E=−dVdr
- This confirms that the negative of the potential gradient is indeed equal to the electric field.
- Therefore, Statement 2 is also true.
- Both statements are true, and Statement 2 serves as an explanation for Statement 1. Thus, the correct answer is that both statements are true.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - SECTION-F(MATRIX-MATCH TYPE QUESTIONS)
3 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - SECTION-G(INTEGER ANSWER TYPE QUESTIONS)
9 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - COMPREHENSION-II
3 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions
25 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
15 Videos
Similar Questions
Why should electrostatic field be zero inside a conductor ?
View SolutionConsider the three statements in context of electrostatic field lines.
STATEMENT-1 : Electrostatic field lines are continuous curves in space.
STATEMENTS-2 : Electrostatic field lines are always perpendicular to equipotential surface.
STATEMENT-3 : A charge when released in electrostatic field moves along electrostatic field lines.
View Solution
Knowledge Check
In the given circuit the potential at point B is zero, the potential at points A and D will be
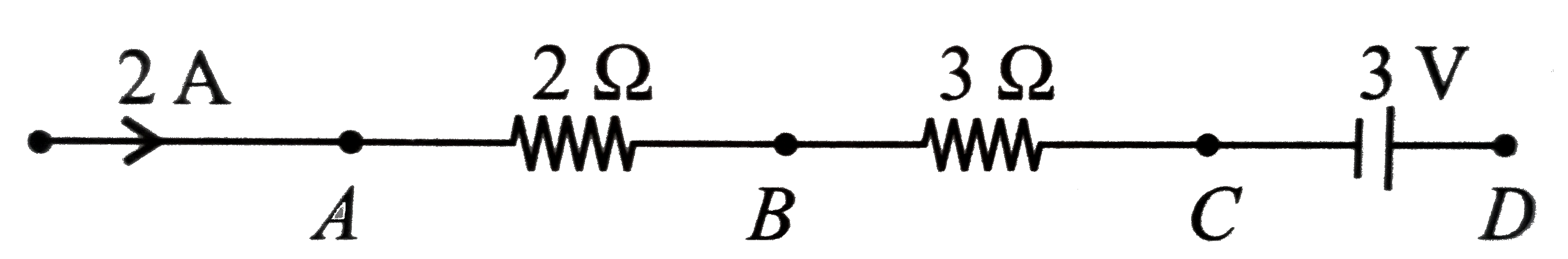 AVA=4V,VD=9VBVA=3V,VD=4VCVA=9V,VD=3VDVA=4V,VD=3V
AVA=4V,VD=9VBVA=3V,VD=4VCVA=9V,VD=3VDVA=4V,VD=3V
STATEMENT -1 : Induced charge does not contribute to electric field or potential at a give point .
STATEMENT -2 : A point charge q0 is kept outside a solid metallic sphere , the electric field inside the sphere is zero.
View SolutionSTATEMENT-1 : The electrostatic force is much stronger than the gravitational force.
STATEMENT-2 : Electrostatic force can be attractive or repulsive.
STATEMENT-3 : Electrostatic force is central force.
View SolutionShow that the electric field at any point is equal to negative potential gradient at the point.
View SolutionShow that the electric field at any point is equal to negative potential gradient at the point.
View SolutionSTATEMENT -1 : No two electric field line can intersect each other.
and
STATEMENT-2 : At point of intersection electric fieldmust be zero.
View SolutionSTATEMENT-1 : If a charge particle enters into a uniform electric field, then its path must be straight line.
and
STATEMENT -2 : Electrostatic force on a positive charge particle is always in the direction of electric field.
View SolutionSTATEMENT-1 : Absolute value of potential is not defined.
STATEMENT-2 : For a given potential function, electric intensity function can be uniquely found.
STATEMENT- 3 : Potential increases in the direction of electric field
View Solution
