A cylindrical tube of length l has inner radius a while outer radius b. What is the resistance of the tube between (a) its ends (b) inner and outer surfaces ? (The resistivitiy of its material is p)
To solve the problem, we need to find the resistance of a cylindrical tube in two different scenarios:
(a) Between its ends (along the length of the tube)
(b) Between its inner and outer surfaces (radially)
1. Identify the formula for resistance:
The resistance R of a conductor is given by the formula:
R=ρLA
where ρ is the resistivity of the material, L is the length of the conductor, and A is the cross-sectional area.
2. Calculate the cross-sectional area:
The tube has an inner radius a and an outer radius b. The cross-sectional area A of the tube can be calculated by subtracting the area of the inner circle from the area of the outer circle:
A=πb2−πa2=π(b2−a2)
3. Substitute the values into the resistance formula:
Now, substituting the values into the resistance formula:
R=ρLπ(b2−a2)
Thus, the resistance of the tube between its ends is:
R=ρLπ(b2−a2)
---
(b) Resistance between the inner and outer surfaces:
1. Consider a differential element:
To find the resistance between the inner and outer surfaces, consider a thin cylindrical shell of thickness dx at a radius x (where a<x<b).
2. Calculate the differential resistance dR:
The resistance of this thin shell can be expressed as:
dR=ρdxA
where A is the surface area of the cylindrical shell, given by:
A=2πxL
Therefore, the differential resistance becomes:
dR=ρdx2πxL
3. Integrate to find total resistance:
To find the total resistance R from the inner surface (radius a) to the outer surface (radius b), integrate dR from a to b:
R=∫badR=∫baρdx2πxL
This can be simplified as:
R=ρ2πL∫badxx
The integral ∫dxx evaluates to logx, so we have:
R=ρ2πL(logb−loga)=ρ2πLlog(ba)
Thus, the resistance of the tube between its inner and outer surfaces is:
R=ρ2πLlog(ba)
Final Answers
- (a) Resistance between the ends:
R=ρLπ(b2−a2)
- (b) Resistance between the inner and outer surfaces:
R=ρ2πLlog(ba)
(b) Between its inner and outer surfaces (radially)
The resistance R of a conductor is given by the formula:
R=ρLA
where ρ is the resistivity of the material, L is the length of the conductor, and A is the cross-sectional area.
The tube has an inner radius a and an outer radius b. The cross-sectional area A of the tube can be calculated by subtracting the area of the inner circle from the area of the outer circle:
A=πb2−πa2=π(b2−a2)
Now, substituting the values into the resistance formula:
R=ρLπ(b2−a2)
R=ρLπ(b2−a2)
To find the resistance between the inner and outer surfaces, consider a thin cylindrical shell of thickness dx at a radius x (where a<x<b).
The resistance of this thin shell can be expressed as:
dR=ρdxA
where A is the surface area of the cylindrical shell, given by:
A=2πxL
Therefore, the differential resistance becomes:
dR=ρdx2πxL
To find the total resistance R from the inner surface (radius a) to the outer surface (radius b), integrate dR from a to b:
R=∫badR=∫baρdx2πxL
This can be simplified as:
R=ρ2πL∫badxx
The integral ∫dxx evaluates to logx, so we have:
R=ρ2πL(logb−loga)=ρ2πLlog(ba)
R=ρ2πLlog(ba)
- (a) Resistance between the ends:
R=ρLπ(b2−a2)
- (b) Resistance between the inner and outer surfaces:
R=ρ2πLlog(ba)
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - ASSIGNMENT SECTION-J
10 Videos
- CURRENT ELECTRICITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - ASSIGNMENT SECTION-H ( MULTIPLE TRUE-FALE TYPE QUESTIONS)
5 Videos
- COMMUNICATION SYSTEMS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - COMMUNICATION SYSTEMS EXERCISE - ASSIGNMENT SECTION D (Assertion-Reason)
10 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
Similar Questions
A cylinderical tube of length L has inner radius a and outer radius b. What is the resistance of the tube between its inner and outer surface ? Given that the resistivity of its material is ρ.
View SolutionSphere of inner radius a and outer radius b is made of p uniform resistivity find resistance between inner and outer surface
View Solution
Knowledge Check
A copper cylindrical tube has inner radius a and outer radius b. The resistivity is p. The resistance of . the cylinder between the two ends is
Aρlb2−a2Bρl2π(b−a)Cρlπ(b2−a2)Dπ(b2−a2)ρl
Sphere of inner radius a and outer radius b is made of p uniform resistivity find resistance between inner and outer surface
View SolutionA circular running track has inner radius 126 m and outer radius 140 m . Find the area of the track .
View SolutionA coaxial cable is made up of two conductors. The inner conductor is solid is of radius R1 and the outer coductor is hollow of inner radius R2 and outer radius R3 . The space between the conductors are carrying currents of equal magnitudes and in oppsite direactions. then the variation of magnetic field with distance form the axis is best plotted as :

View SolutionA hollow cylinder of specific resistance ρ, inner radius R, outer radius 2R and length l is as shown in figure. What is the net resistance between the inner and outer surfaces?
View SolutionThe hollow cylinder of length l and inner and outer radius R1 and R2 respectively. Find resistance of cylinder if current flows radially outward in the cylinder. Resistivity of material of cylinder id ρ .
View Solution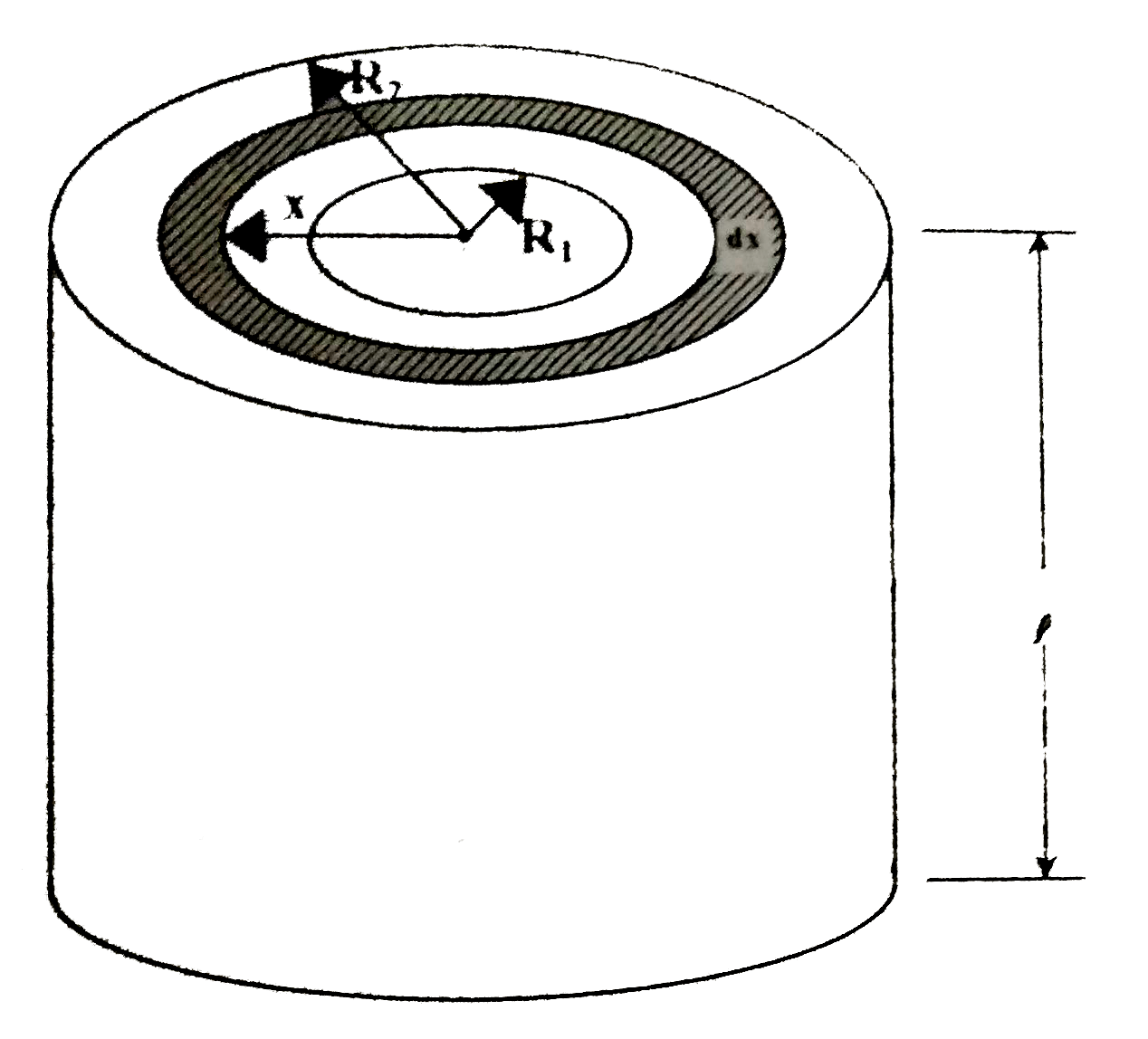
A cylindrical conductor of length l and inner radius R1 and outer radius R2 has specific resistance ρ. A cell of emf ε is connected across the two lateral faces of the conductor. Find the current drown from the cell.
View SolutionA solid conducting sphere of radius a has a net positive charge 2Q. A conducting spherical shell of inner radius b and outer radius c is concentric with the solid sphere and has a net charge −Q. The surface charge density on the inner and outer surfaces of the spherical shell will be
View Solution
