The hollow cylinder of length l and inner and outer radius and respectively. Find resistance of cylinder if current flows radially outward in the cylinder. Resistivity of material of cylinder id .
The correct Answer is:D
To find the resistance of a hollow cylinder with inner radius R1, outer radius R2, and length l, where the current flows radially outward, we can follow these steps:
Step 1: Understand the Resistance Formula
The resistance R of a conductor is given by the formula:
R=ρ⋅LA
where:
- R is the resistance,
- ρ is the resistivity of the material,
- L is the length of the conductor,
- A is the cross-sectional area.
Step 2: Consider an Elemental Thickness
For a hollow cylinder, we consider an elemental thickness dr at a distance r from the center. The length L of this elemental section is l, and the area A at this distance is the lateral surface area of the cylindrical shell:
A=2πr⋅l
Step 3: Expression for Resistance of the Element
The resistance dR of this elemental thickness dr can be expressed as:
dR=ρ⋅drA=ρ⋅dr2πr⋅l
Step 4: Integrate to Find Total Resistance
To find the total resistance R of the hollow cylinder from radius R1 to R2, we integrate dR:
R=∫R2R1dR=∫R2R1ρ⋅dr2πr⋅l
This simplifies to:
R=ρ2πl∫R2R1drr
Step 5: Solve the Integral
The integral ∫drr evaluates to lnr:
R=ρ2πl(lnr)R2R1=ρ2πl(lnR2−lnR1)
Using properties of logarithms, this can be rewritten as:
R=ρ2πlln(R2R1)
Final Result
Thus, the total resistance R of the hollow cylinder is:
R=ρ2πlln(R2R1)
The resistance R of a conductor is given by the formula:
R=ρ⋅LA
where:
- R is the resistance,
- ρ is the resistivity of the material,
- L is the length of the conductor,
- A is the cross-sectional area.
For a hollow cylinder, we consider an elemental thickness dr at a distance r from the center. The length L of this elemental section is l, and the area A at this distance is the lateral surface area of the cylindrical shell:
A=2πr⋅l
The resistance dR of this elemental thickness dr can be expressed as:
dR=ρ⋅drA=ρ⋅dr2πr⋅l
To find the total resistance R of the hollow cylinder from radius R1 to R2, we integrate dR:
R=∫R2R1dR=∫R2R1ρ⋅dr2πr⋅l
This simplifies to:
R=ρ2πl∫R2R1drr
The integral ∫drr evaluates to lnr:
R=ρ2πl(lnr)R2R1=ρ2πl(lnR2−lnR1)
Using properties of logarithms, this can be rewritten as:
R=ρ2πlln(R2R1)
Thus, the total resistance R of the hollow cylinder is:
R=ρ2πlln(R2R1)
Topper's Solved these Questions
Similar Questions
A hollow cylinder (ρ=2.2×10−8Ω−m) of length 3 m has inner and outer diameters are 2 mm and 4 mm respectively. The resistance of the cylinder is
View SolutionConsider a hollow cylinder of length L and inner radius a and outer radius b, as shown in figure. The material has resistivity ρ.
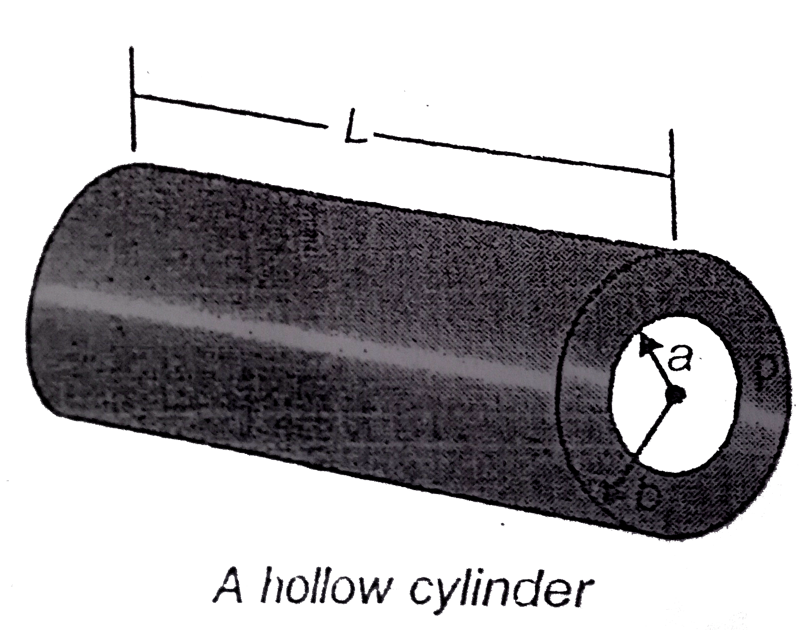
(a) Suppose a potential difference is applied between te ends of the cylinder and produces a current parallel to the axis. What is the resistance measure ?
(b) If instead the potential difference is applied between the inner outer surfaces so that current flows radially outward, what is the resistance measure ?
View Solution
Knowledge Check
A copper cylindrical tube has inner radius a and outer radius b. The resistivity is p. The resistance of . the cylinder between the two ends is
Aρlb2−a2Bρl2π(b−a)Cρlπ(b2−a2)Dπ(b2−a2)ρl
A cylinderical tube of length L has inner radius a and outer radius b. What is the resistance of the tube between its inner and outer surface ? Given that the resistivity of its material is ρ.
View SolutionFind the resistance of a hollow cylindrical conductor of length 1.0m and inner and outer radii 1.0mm and 2.0mm respectively. The resistivity of the material is 2.0×10−8(Ω)m.
View SolutionA resistor is constructed as hollow cylinder with inner and outer radii ra=0.5cm,rb=1.0 cm respectively and resistivity ρ=3.5×10−5Ω. The resistance of the configuration for the length of 5 cm cylinder is _______×10−3Ω.
View SolutionThe inner and outer radii of a hollow cylinder are 15 cm and 20 cm, respectively. The cylinder is melted and recast into a solid cylinder of the same height. Find the radius of the base of new cylinder.
View SolutionA cylinder of radius R and length l is placed in a uniform electric field E parallel to the axis of the cylinder. The total flux over the curved surface of the cylinder is
View SolutionA hollow cylinder of specific resistance ρ, inner radius R, outer radius 2R and length l is as shown in figure. What is the net resistance between the inner and outer surfaces?
View SolutionCalculate thermal conductance for radial flow of an annular cylinder of length l and inner and outer radius r1 and r2. Assume that thermal conductivity of the material is K

View Solution