If v is the velocity of a body moving along x-axis, then acceleration of body is
dvdx
vdvdx
xdudx
vdxdv
The correct Answer is:B
To solve the problem of finding the acceleration of a body moving along the x-axis when given its velocity v, we can follow these steps:
1. Understand the Definitions:
- Velocity v is defined as the rate of change of displacement x with respect to time t:
v=dxdt
- Acceleration a is defined as the rate of change of velocity v with respect to time t:
a=dvdt
2. Relate Time to Displacement:
- We can express dt in terms of dx and v:
dt=dxv
3. Substitute dt into the Acceleration Formula:
- Now, we substitute dt in the acceleration formula:
a=dvdt=dvdx⋅dxdt
- Since dxdt=v, we can rewrite the equation as:
a=dvdx⋅v
4. Final Expression for Acceleration:
- Thus, the expression for acceleration becomes:
a=vdvdx
5. Identify the Correct Option:
- From the options given, the correct expression for the acceleration of the body is:
a=vdvdx
- Therefore, the correct answer is option 2: vdvdx.
- Velocity v is defined as the rate of change of displacement x with respect to time t:
v=dxdt
- Acceleration a is defined as the rate of change of velocity v with respect to time t:
a=dvdt
- We can express dt in terms of dx and v:
dt=dxv
- Now, we substitute dt in the acceleration formula:
a=dvdt=dvdx⋅dxdt
- Since dxdt=v, we can rewrite the equation as:
a=dvdx⋅v
- Thus, the expression for acceleration becomes:
a=vdvdx
- From the options given, the correct expression for the acceleration of the body is:
a=vdvdx
- Therefore, the correct answer is option 2: vdvdx.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - B)
33 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - C)
36 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - EXERCISE
29 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -J (Aakash Challengers Questions)
7 Videos
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - J)
2 Videos
Similar Questions
If the average velocity of a body moving with uniform acceleration under the action of a force is ''v'' and the impulse it receives during a displacement of ''s'' is ''I'', the constant force acting on the body is given by
View SolutionThe force acting on a body moving along x-axis variation of the particle particle shown in the figure. The body is in stable equilibrium at
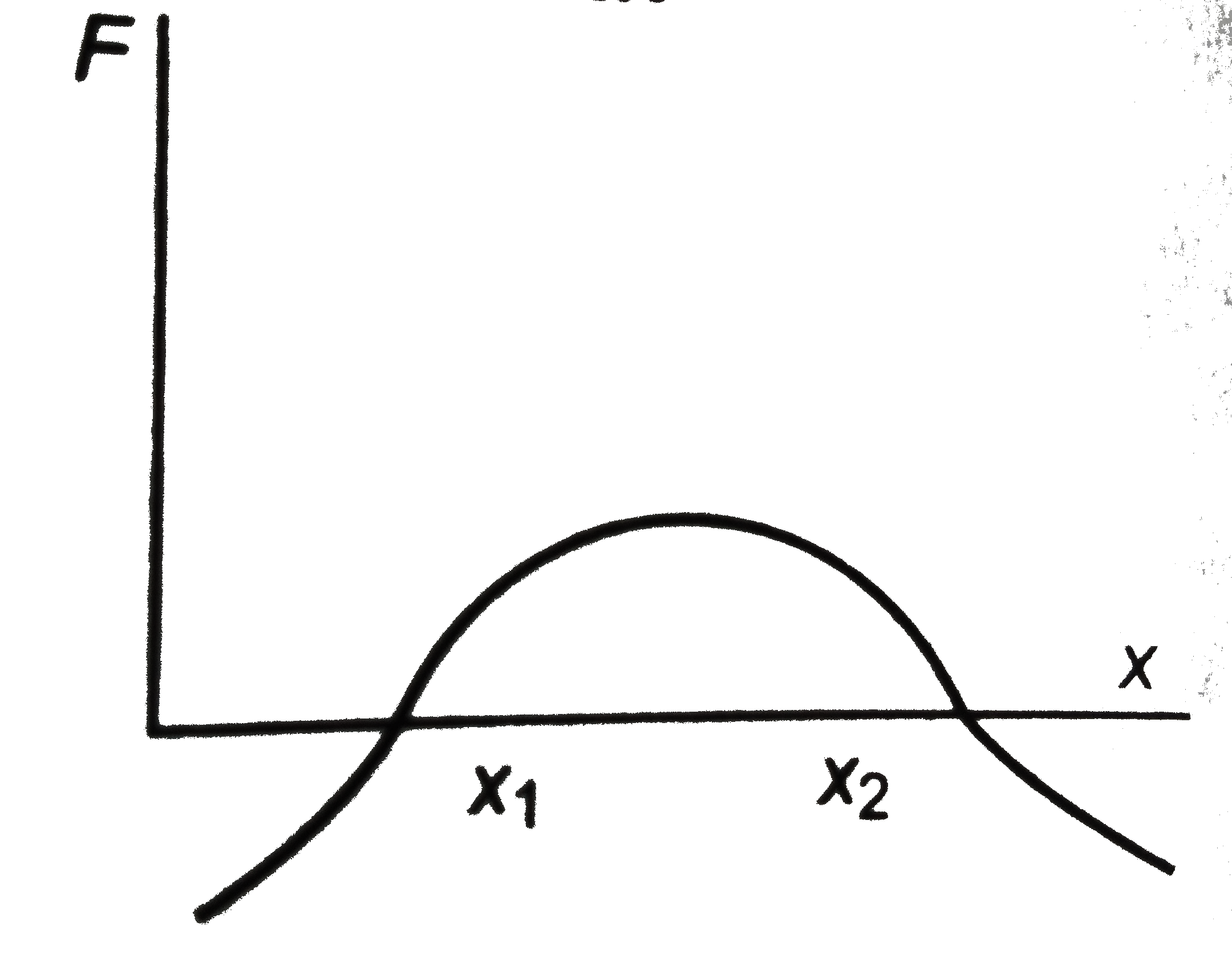 .
.
View Solution
Knowledge Check
A body moving with some initial velocity and having uniform acceleration attains a final velocity v m/s after travelling x m. If its final velocity is v = √180−7x, find the acceleration of the body.
A−3.5m/s2B−7m/s2C−15m/s2D−30m/s2
The force acting on a body moving along x -axis varies with the position of the particle as shown in the fig. The body is in stable equilibrium at.
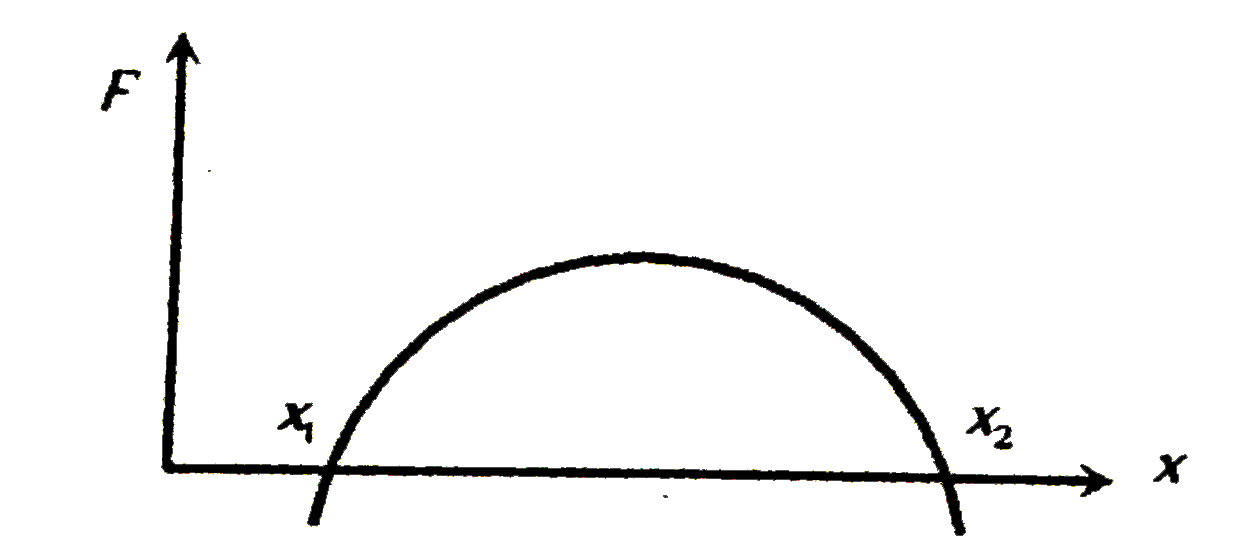
View SolutionThe force acting on a body moving along x-axis variation of the particle particle shown in the figure. The body is in stable equilibrium at
 .
.
View SolutionA : For a particle moving in a straight line, its acceleration must be either parallel or antiparallel to velocity.
R : A body moving along a curved path may have constant acceleration.
View SolutionThe velocity v of a body moving along a straight line varies with time t as v=2t2e−t, where v is in m/s and t is in second. The acceleration of body is zero at t =
View SolutionA body is moving with a velocity 5m/s along a striaght line.A force of 10N is applied to the body perpendicular to the line of motion. If the body is of mass 2kg, then the acceleration of the body along the initial straight line of motion is :
View SolutionAt time t, the position of a body moving along the x - axis is x=t3−6t2+9tm The deceleration of the body at 1 s is
View SolutionA particle starts with some initial velocity with an acceleration along the direction of motion. Draw a graph depicting the variation of velocity (v) along y-axis with the variation of displacement(s) along x-axis.
View Solution
