An object of mass 3kg is at rest. Now a force of →F=6t2ˆi+4tˆj is applied on the object, the velocity of object at t=3s is.
18ˆi+3ˆj
18ˆi+6ˆj
3ˆi+18ˆj
18ˆi+4ˆj
To find the velocity of the object at t=3s, we will follow these steps:
Step 1: Identify the given information
- Mass of the object, m=3kg
- Force applied, →F=6t2^i+4t^j
- Initial velocity, →u=0 (since the object is at rest)
- Time, t=3s
Step 2: Calculate the impulse
Impulse →I is given by the integral of the force over time:
→I=∫30→Fdt=∫30(6t2^i+4t^j)dt
Step 3: Evaluate the integral
We will evaluate the integral component-wise.
For the ^i component:
∫306t2dt=6(t33)30=6(333−033)=6(273)=6×9=54
For the ^j component:
∫304tdt=4(t22)30=4(322−022)=4(92)=18
Thus, the total impulse is:
→I=54^i+18^j
Step 4: Relate impulse to change in momentum
Impulse is equal to the change in momentum:
→I=m→v−m→u
Since →u=0:
→I=m→v
Substituting the values:
54^i+18^j=3→v
Step 5: Solve for velocity →v
Now, divide both sides by the mass m=3:
→v=54^i+18^j3=18^i+6^j
Final Answer
The velocity of the object at t=3s is:
→v=18^i+6^jm/s
- Mass of the object, m=3kg
- Force applied, →F=6t2^i+4t^j
- Initial velocity, →u=0 (since the object is at rest)
- Time, t=3s
Impulse →I is given by the integral of the force over time:
→I=∫30→Fdt=∫30(6t2^i+4t^j)dt
We will evaluate the integral component-wise.
∫306t2dt=6(t33)30=6(333−033)=6(273)=6×9=54
∫304tdt=4(t22)30=4(322−022)=4(92)=18
→I=54^i+18^j
Impulse is equal to the change in momentum:
→I=m→v−m→u
Since →u=0:
→I=m→v
Substituting the values:
54^i+18^j=3→v
Now, divide both sides by the mass m=3:
→v=54^i+18^j3=18^i+6^j
The velocity of the object at t=3s is:
→v=18^i+6^jm/s
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-D) (Assertion-Reason Type Questions)
15 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-B)
44 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
Similar Questions
An object of mass 3 kg is at rest . Now a force F=6t2ˆi+2tˆj is applied on the object . Find the velocity of the object at t =3 sec.
View SolutionAn object of mass 2 kg at rest at origin starts moving under the action of a force
→F=(3t2ˆi+4tˆj)N The velocity of the object at t = 2 s will be -
View Solution
Knowledge Check
A body with mass 5 kg is acted upon by a force →F=(−3ˆi+4ˆj)N. If its initial velocity at t =0 is →v=6ˆi−12ˆjms−1, the time at which it will just have a velocity along the y-axis is :
A0B10sC2sD15s
A body with mass 5 kg is acted upon by a force →F=(−3ˆi+4ˆj)N. If its initial velocity at t =0 is →v=(6ˆi−12ˆj)ms−1, the time at which it will just have a velocity along the y-axis is :
View SolutionA body with mass 5 kg is acted upon by a force →F=(−3ˆi+4ˆj)N. If its initial velocity at t =0 is →v=6ˆi−12ˆjms−1, the time at which it will just have a velocity along the y-axis is :
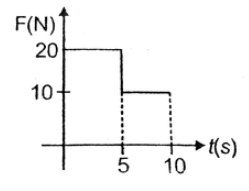
View SolutionForce is applied on an object of mass 2 kg at rest on a frictionless horizontal surface as shown in the graph. The speed of object at t=10 s will be
View SolutionA body of mass 1kg begins to move under the action of a time dependent force →F=(2tˆi+3t2ˆj)N, where ˆi and ˆj are unit vectors along x-and y-axes. What power will be developed by the force at the time t ?
View SolutionThe displacement s of an object is given as a function of time t by the following equation s=2t+5t2+3t3. Calculate the instantaneous velocity of the object at t = 1 s.
View SolutionThe displacement x of an object is given as a funstion of time, x=2t+3t2. The instantaneous velocity of the object at t = 2 s is
View SolutionThe time dependence of the position of a particle of mass m = 2 is given by →r(t)=2tˆi−3t2ˆj Its angular momentum, with respect to the origin, at time t = 2 is :
View Solution
