A rope 1 cm in diameter breaks, if the tension in it exceeds 500 N. The maximum tension that may be given to similar rope of diameter 3 cm is
500 N
3000 N
4500 N
2000 N
To solve the problem, we need to determine the maximum tension that a rope of diameter 3 cm can withstand, given that a rope of diameter 1 cm breaks under a tension of 500 N.
1. Identify Given Information:
- Diameter of the first rope (D1) = 1 cm
- Maximum tension for the first rope (T1) = 500 N
- Diameter of the second rope (D2) = 3 cm
2. Understand the Concept of Breaking Stress:
- Breaking stress is defined as the maximum tension (T) divided by the cross-sectional area (A) of the rope.
- The formula for the area of a circular cross-section is given by:
A=π4d2
- Therefore, breaking stress (σ) can be expressed as:
σ=TA=Tπ4d2
3. Set Up the Equation for Breaking Stress:
- Since the breaking stress is the same for both ropes, we can write:
T1π4D12=T2π4D22
- The π4 cancels out from both sides, simplifying to:
T1D12=T2D22
4. Rearrange the Equation to Find T2:
- Rearranging gives us:
T2=T1⋅D22D12
5. Substitute the Values:
- Substitute T1=500N, D1=1cm, and D2=3cm:
T2=500⋅(3)2(1)2=500⋅91=500⋅9=4500N
6. Conclusion:
- The maximum tension that the rope of diameter 3 cm can withstand is 4500 N.
- Diameter of the first rope (D1) = 1 cm
- Maximum tension for the first rope (T1) = 500 N
- Diameter of the second rope (D2) = 3 cm
- Breaking stress is defined as the maximum tension (T) divided by the cross-sectional area (A) of the rope.
- The formula for the area of a circular cross-section is given by:
A=π4d2
- Therefore, breaking stress (σ) can be expressed as:
σ=TA=Tπ4d2
- Since the breaking stress is the same for both ropes, we can write:
T1π4D12=T2π4D22
- The π4 cancels out from both sides, simplifying to:
T1D12=T2D22
- Rearranging gives us:
T2=T1⋅D22D12
- Substitute T1=500N, D1=1cm, and D2=3cm:
T2=500⋅(3)2(1)2=500⋅91=500⋅9=4500N
- The maximum tension that the rope of diameter 3 cm can withstand is 4500 N.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment(Section-D)
14 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment(Section-B)
26 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - J
9 Videos
- Mock test 03BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - Mock test 03EXERCISE - EXAMPLE
44 Videos
Similar Questions
Knowledge Check
If the surface tension of water is 0.06 N/m, then the capillary rise in a tube of diameter 1mm is (θ=0∘)
A1.22 cmB2.44 cmC3.12 cmD3.86 cmSurface tension of mercury is 0.465Nm−1. The excess pressure inside a mercury drop of diameter 6mm is
A310 paB410 paC510 paD610 pa
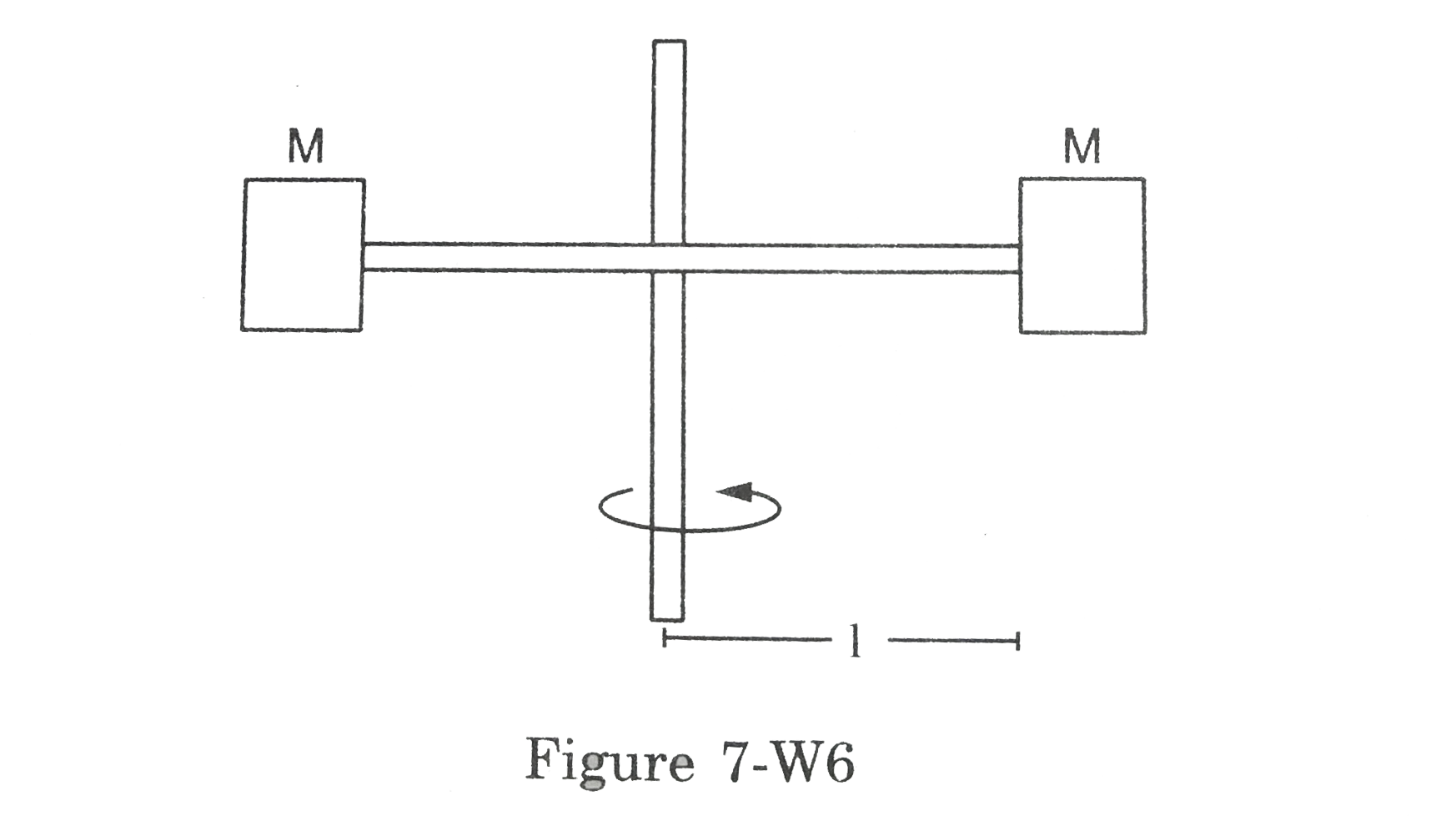
Two blocks each of mass M are connected to the ends of a light frame as shown in figure. The frame is rotated about the vertical line of symmetry. The rod breaks if the tension in it exceeds T0. Find the maximum frequency with which the frame may be rotted without breaking the rod.
View SolutionSurface tension of water is 0.072 Nm−1. The excess pressure inside a water drop of diameter 1.2 mm is :-
View SolutionA steel wire of diameter 2 mm has a breaking strength of 4×105N. What is the breaking strength of similar steel wire of diameter 1.5mm ?
View SolutionA steel wire of diameter 2 mm has a breaking strength of 4×105N. What is the breaking strength of similar steel wire of diameter 1.5mm ?
View SolutionFind the volume of a spherewhose diameter is 1 cm.
View SolutionA 50 kg man stuck in flood is being lifted vertically by an army helicopter with the help of light rope which can bear a maximum tension of 70 kg-wt. The maximum acceleration with which helicopter can rise so that rope does not breaks is (g=9.8ms−2)
View Solution