A steel wire of diameter 2 mm has a breaking strength of N. What is the breaking strength of similar steel wire of diameter ?
a.
b.
d.
The correct Answer is:A
To find the breaking strength of a steel wire of diameter 1.5 mm, given that a similar wire of diameter 2 mm has a breaking strength of 4×105 N, we can follow these steps:
Step 1: Understand the relationship between breaking strength and diameter
The breaking strength (F) of a wire is directly proportional to the cross-sectional area (A) of the wire. For a circular wire, the area can be expressed in terms of the diameter (d):
A=πd24
Thus, we can express the breaking strength as:
F∝A∝d2
Step 2: Set up the ratio of breaking strengths
Let F1 be the breaking strength of the first wire (diameter d1=2mm) and F2 be the breaking strength of the second wire (diameter d2=1.5mm). From the proportionality, we have:
F1F2=d21d22
Step 3: Substitute known values
We know:
- F1=4×105N
- d1=2mm
- d2=1.5mm
Substituting these values into the ratio gives:
4×105F2=(2)2(1.5)2
Step 4: Calculate the squares of the diameters
Calculating the squares:
- d21=22=4
- d22=(1.5)2=2.25
Thus, we can rewrite the ratio:
4×105F2=42.25
Step 5: Solve for F2
Cross-multiplying gives:
4×105⋅2.25=4⋅F2
F2=4×105⋅2.254
F2=2.25×105N
Conclusion
The breaking strength of the steel wire with a diameter of 1.5 mm is:
F2=2.25×105N
The breaking strength (F) of a wire is directly proportional to the cross-sectional area (A) of the wire. For a circular wire, the area can be expressed in terms of the diameter (d):
A=πd24
Thus, we can express the breaking strength as:
F∝A∝d2
Let F1 be the breaking strength of the first wire (diameter d1=2mm) and F2 be the breaking strength of the second wire (diameter d2=1.5mm). From the proportionality, we have:
F1F2=d21d22
We know:
- F1=4×105N
- d1=2mm
- d2=1.5mm
4×105F2=(2)2(1.5)2
Calculating the squares:
- d21=22=4
- d22=(1.5)2=2.25
4×105F2=42.25
Cross-multiplying gives:
4×105⋅2.25=4⋅F2
F2=4×105⋅2.254
F2=2.25×105N
The breaking strength of the steel wire with a diameter of 1.5 mm is:
F2=2.25×105N
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment (SECTION - B)
15 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment (SECTION - C)
2 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Try Yourself
32 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - J
9 Videos
- Mock test 03BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - Mock test 03EXERCISE - EXAMPLE
44 Videos
Similar Questions
Knowledge Check
A steel wire of length 20 cm and uniform cross section 1 mm2 is tied rigidly at both the ends. The temperature of the wire is altered from 40∘ C to 20∘C Coefficient of linear expansion for steel α=1.1×10−5l∘C and Y for steel is 2.0×1011N/m2. The change in tension of the wire is
A2.2×106 newtonB16 newtonC8 newtonD44 newton
If the breaking force of a nylon rope of diameter 4cm is 1.6×105N, find the breaking force of the same nylon rope of diameter 2 cm
View SolutionA 1m long wire of diameter 0.31mm has a resistance of 4.2Ω. If it is replaced by another wire of same material of length 1.5m and diameter 0.155mm, then the resistance of wire is
View SolutionA steel wire of length 4 m and diameter 5 mm is stretched by 5kg-wt. Find the increase in its length if the Young's modulus of steel wire is 2.4×1012dynecm−2
View SolutionA steel wire of length 4 m and diameter 5 mm is strethed by 5 kg-wt. Find the increase in its length, if the Young's modulus of steel is 2×1012 dyne cm2
View SolutionTwo wires of diameter 0.25 cm, one made of steel and the other made of brass are loaded as shown in fig. The unloaded length of steel wire is 1.5m and that of brass wire is 1.0m. Compute the elongations of the steel and the brass wires.
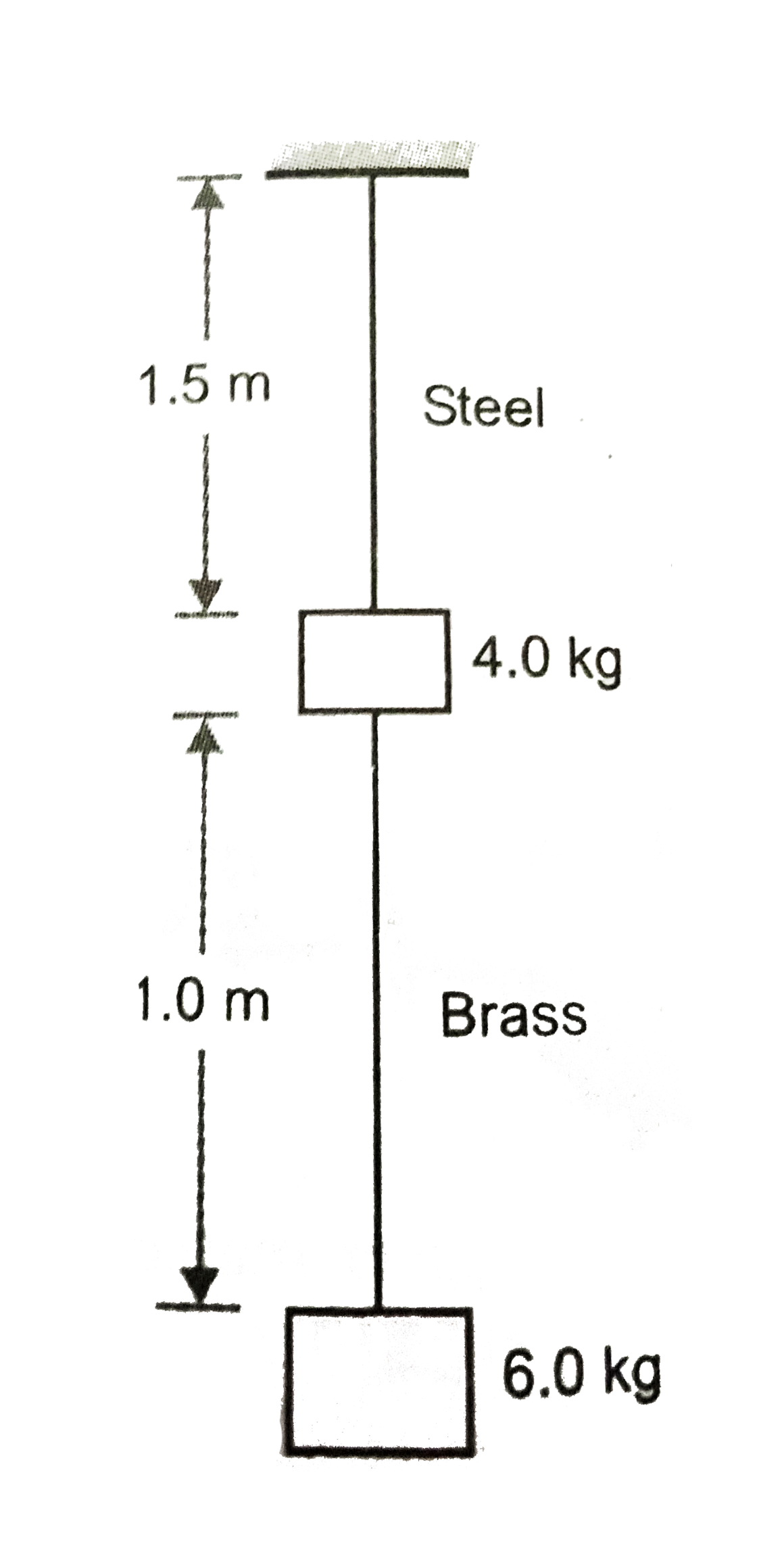
View SolutionA steel wire of length 1m and diameter 0.8 mm is kept stretched between two rigid supports. What additional stretching force the wire will experience when its temperature falls by 30K? Linear expansivity of steel α=12×10−6/k . Young's modulus of steel Y=20×1010N/m2.
View SolutionA rope 1 cm in diameter breaks if the tension in it exceeds 500 N. The maximum tension that may be given to a similar rope of diameter 2 cm is
View Solution
