The minimum and maximum distances of a planet revolving around sun are r and R . If the minimum speed of planet of its trajectory is v0 , its maximum speed will be
v0Rr
v0rR
v0R2r2
v0r2R2
The correct Answer is:A
To find the maximum speed of a planet revolving around the Sun, given its minimum speed v0 at a distance r (perihelion) and maximum distance R (aphelion), we can use the principle of conservation of angular momentum.
1. Understanding Angular Momentum:
The angular momentum L of a planet moving in an elliptical orbit is conserved. It can be expressed as:
L=m⋅v⋅r
where m is the mass of the planet, v is its speed, and r is the distance from the Sun.
2. Setting Up the Equation:
At perihelion (minimum distance r), the speed is vmin=v0.
At aphelion (maximum distance R), the speed is vmax.
Therefore, we can write:
Lperihelion=Laphelion
This gives us:
m⋅v0⋅r=m⋅vmax⋅R
3. Canceling the Mass:
Since the mass m is the same on both sides, we can cancel it out:
v0⋅r=vmax⋅R
4. Solving for Maximum Speed:
Rearranging the equation to solve for vmax:
vmax=v0⋅rR
5. Substituting the Given Values:
Since v0 is given as the minimum speed, we can directly substitute:
vmax=v0⋅rR
Final Answer:
Thus, the maximum speed of the planet is:
vmax=v0⋅rR
The angular momentum L of a planet moving in an elliptical orbit is conserved. It can be expressed as:
L=m⋅v⋅r
where m is the mass of the planet, v is its speed, and r is the distance from the Sun.
At perihelion (minimum distance r), the speed is vmin=v0.
At aphelion (maximum distance R), the speed is vmax.
Therefore, we can write:
Lperihelion=Laphelion
This gives us:
m⋅v0⋅r=m⋅vmax⋅R
Since the mass m is the same on both sides, we can cancel it out:
v0⋅r=vmax⋅R
Rearranging the equation to solve for vmax:
vmax=v0⋅rR
Since v0 is given as the minimum speed, we can directly substitute:
vmax=v0⋅rR
Thus, the maximum speed of the planet is:
vmax=v0⋅rR
Topper's Solved these Questions
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - B (OBJECTIVE TYPE QUESTIONS)
22 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - C (PREVIOUS YEARS QUESTIONS)
51 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - EXERCISE
17 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - ASSIGNMENT SECTION - D
9 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
Similar Questions
Knowledge Check
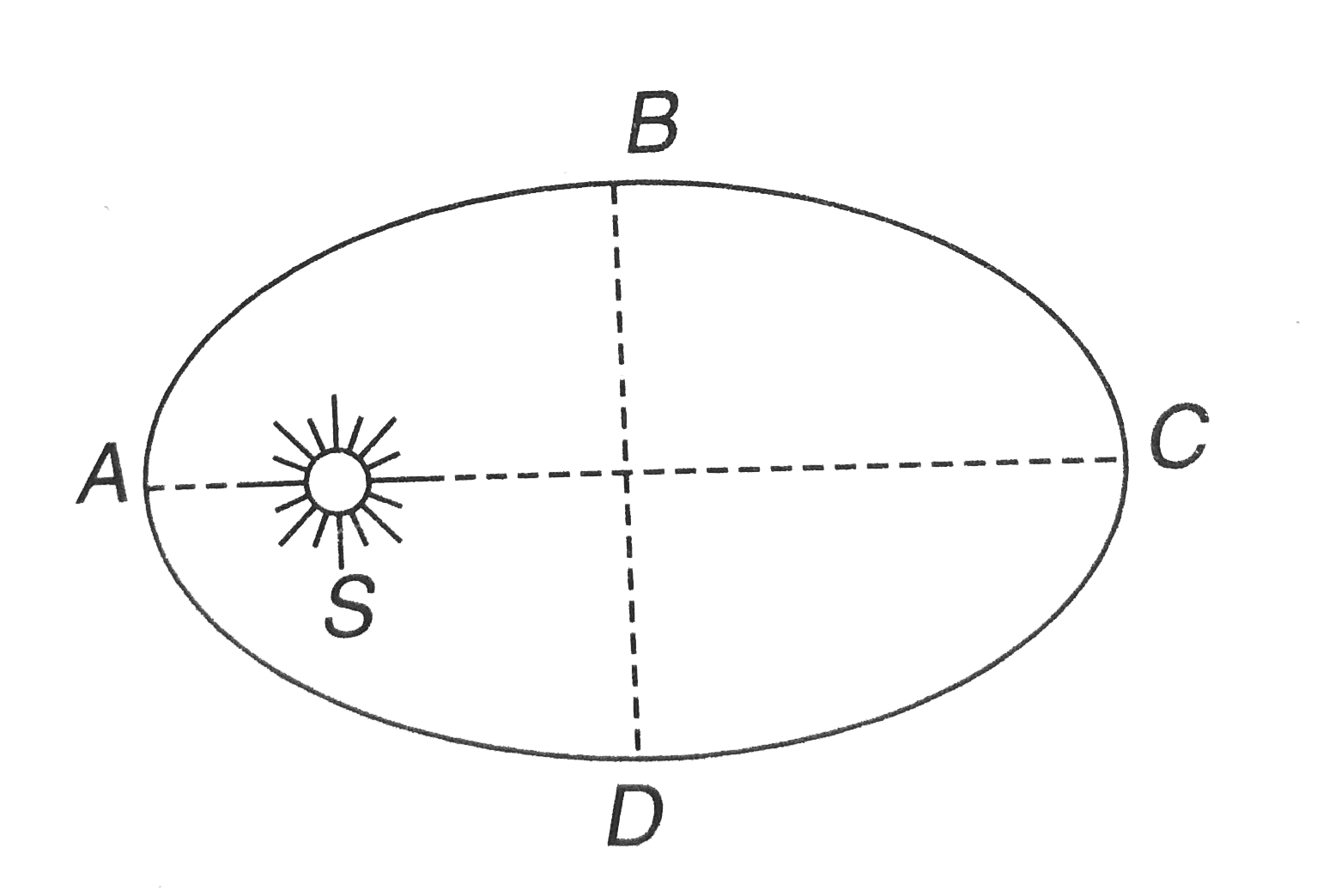
A planet revolves in elliptical orbit around the sun. (see figure). The linear speed of the planet will be maximum at
 AABBCCDD
AABBCCDD
A planet is revolving around the sun as shown in elliptical path. The correct option is
View SolutionA planet of mass m moves around the Sun of mass Min an elliptical orbit. The maximum and minimum distance of the planet from the Sun are r1 and r2, respectively. Find the relation between the time period of the planet in terms of r1 and r2.
View SolutionThe earth revolves round the sun in an elliptical orbit. Its speed
View SolutionA planet revolves in elliptical orbit around the sun. (see figure). The linear speed of the planet will be maximum at

View SolutionThe minimum and maximum distances of a satellite from the center of the earth are 2R and 4R respectively, where R is the radius of earth and M is the mass of the earth . Find
(a) its minimum and maximum speeds,
(b) radius of curvature at the point of minimum distance.
View SolutionFor a planet revolving around sun, if a and b are the respective semi-major and semi-minor axes, then the square of its time period is proportional to
View SolutionFor a planet revolving around Sun in an elliptical orbit, to obtain its velocity at any point we have to apply
View Solution

