A vertical U-tube of uniform cross-section contains water jn both the arms. A 10 cm glycerine column (R.D. = 1.2) is added to one of the limbs. The level difference between the two free surfaces in the two limbs wiil be
4cm
2cm
6cm
8cm
Step by step video & image solution for A vertical U-tube of uniform cross-section contains water jn both the arms. A 10 cm glycerine column (R.D. = 1.2) is added to one of the limbs. The level difference between the two free surfaces in the two limbs wiil be by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
_S01_015_Q01.png)
A vertical U-tube of uniform cross-section contains mercury in both arms A glycerine (relative density =1.3) column of length 10 cm is introduced into one of the arms. Oil of density 800 gm m−3 is poured into the other arm until the upper surface of the oil and hlycerine are at the same horizontal level, find the length of the column. Density of mercury is 13.6×103kgm−3View SolutionA vertical U-tube of uniform cross-section contains water upto a height of 30 cm. show that if the water in one limb is depressed and then released, its up and down motion in the two limbs of the tube is simple harmonic and calculate its time-period.
View SolutionA vertical U shaped tube of uniform cross-section contains mercury in both limbs. Another liquid of density 3.4gcm3 is poured In one limb of column length 4 cm as shown in figure. Then difference (h) between the upper level of liquid in both the limbs is (densityofmercury=13.6gcm3)
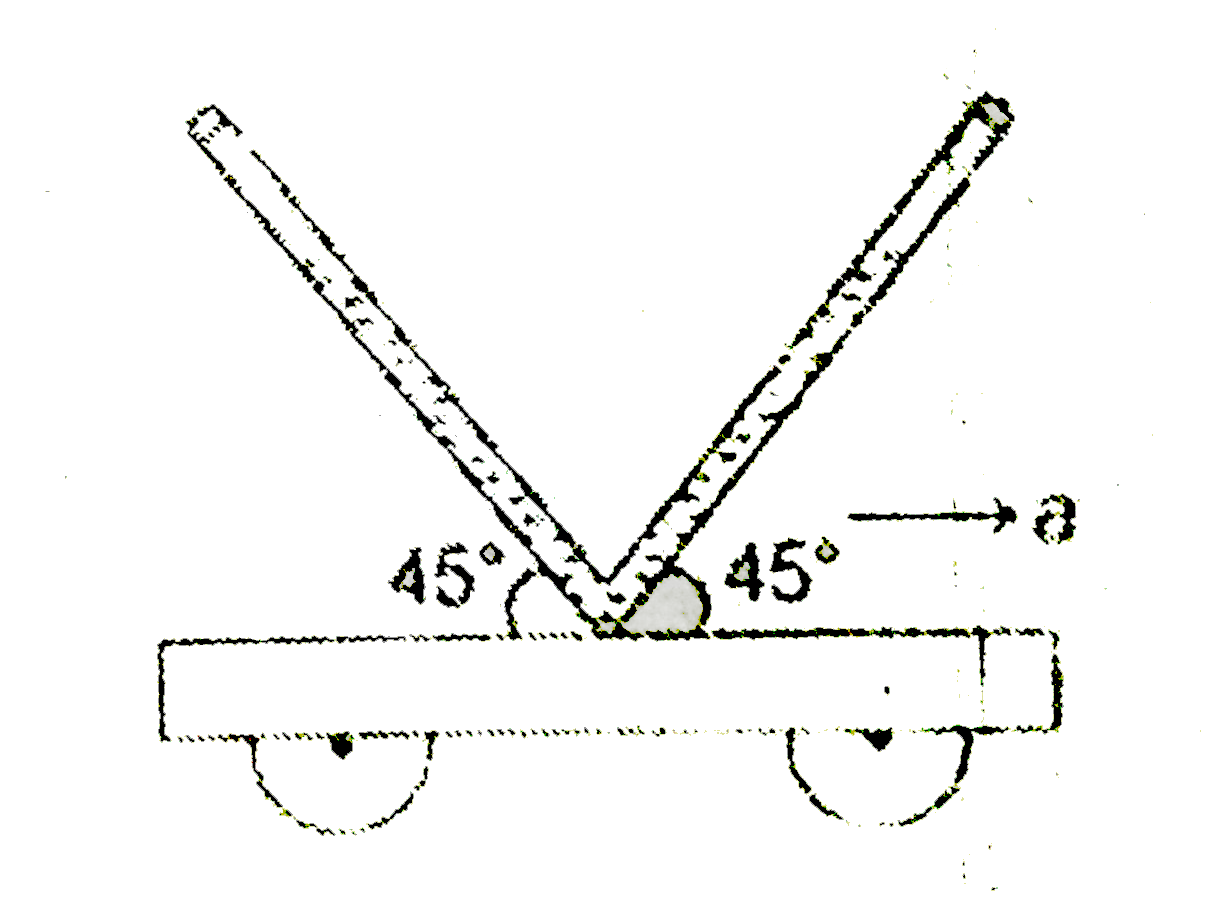
View SolutionA bent tube of uniform cross section is mounted on a cart which is accelerating towards right with constant acceleration 'a'. The total length of the liquid is 2√2l. Find the level difference between two limbs in equilibrium (a=2m/s2,l=10m)
View SolutionA vertical U-tube of uniform cross-section contains water upto a height to 0.8 m. Show. that if the water on one side is depressed and then released, its motion up and down the tube is shm. Calculate the period.
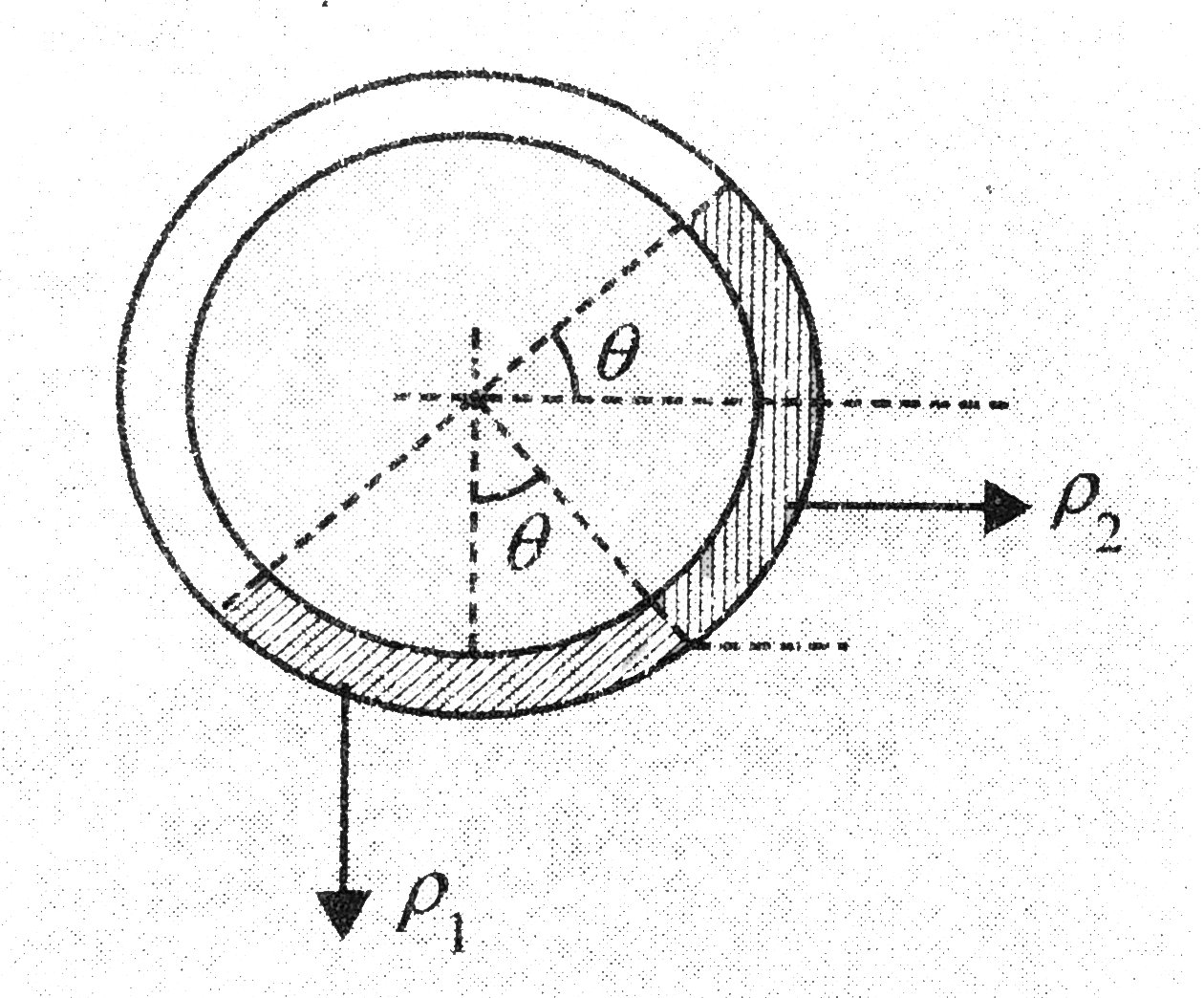
View SolutionA circular tube of uniform cross section is filled with two liquids densities ρ1 and ρ2 such that half of each liquid occupies a quarter to volume of the tube. If the line joining the free surfaces of the liquid makes an angle θ with horizontal find the value of θ.
View Solution_E01_064_Q01.png)
A U-tube of small and uniform cross section contains water of total length 4H the height difference between the water columns on the left and on the right is H when the valve K is closed, the valve is suddenly open, and water is flowing from left to right. ignore friction find the speed of water when the heights of the left and the right water columns are the same.View SolutionIn a U-tube diamter of two limbs are 0.5 cm and 1 cm respectively and tube has filled with water (T=72dyne/cm) then liquid level difference between two limbs will be
View Solution_E01_110_Q01.png)
The U-tube shown has uniform cross-section A liquid is filled in the two arms up to height h1 and h2 and then the liquid is allowed to move neglect viscosity and surface tension. When the levels equalize in the two arms, the liquid willView SolutionIn the figure, the cross-sectional area of the smaller tube is a and that of the larger tube is 2a. A block of mass m is kept in the smaller tube having the base area that of the tube. The difference between water levels of the two tubes is
View Solution