A square of side L meters lies in the x-y plane in a region, where the magnetic field is given by B=B0(2ˆi+3ˆj+4ˆk)T, where B0 is constant. The magnitude of flux passing through the square is
2B0L2Wb
3B0L2Wb
4B0L2Wb
√29B0L2Wb
The correct Answer is:C
To find the magnitude of the magnetic flux passing through a square of side L meters lying in the x-y plane, we can follow these steps:
Step 1: Identify the Area Vector
Since the square lies in the x-y plane, the area vector →A of the square will be perpendicular to the plane. The direction of the area vector will be along the z-axis. The magnitude of the area A of the square is given by:
A=L2
Thus, the area vector can be expressed as:
→A=L2^k
Step 2: Write the Magnetic Field Vector
The magnetic field →B is given as:
→B=B0(2^i+3^j+4^k)
where B0 is a constant.
Step 3: Calculate the Magnetic Flux
The magnetic flux Φ through the square is calculated using the formula:
Φ=→B⋅→A
Substituting the expressions for →B and →A:
Φ=B0(2^i+3^j+4^k)⋅(L2^k)
Step 4: Perform the Dot Product
Now, we perform the dot product:
Φ=B0L2(2^i⋅^k+3^j⋅^k+4^k⋅^k)
Since ^i⋅^k=0 and ^j⋅^k=0, we have:
Φ=B0L2(0+0+4)=4B0L2
Step 5: State the Final Answer
Thus, the magnitude of the magnetic flux passing through the square is:
Φ=4B0L2 Weber
Since the square lies in the x-y plane, the area vector →A of the square will be perpendicular to the plane. The direction of the area vector will be along the z-axis. The magnitude of the area A of the square is given by:
A=L2
Thus, the area vector can be expressed as:
→A=L2^k
The magnetic field →B is given as:
→B=B0(2^i+3^j+4^k)
where B0 is a constant.
The magnetic flux Φ through the square is calculated using the formula:
Φ=→B⋅→A
Substituting the expressions for →B and →A:
Φ=B0(2^i+3^j+4^k)⋅(L2^k)
Now, we perform the dot product:
Φ=B0L2(2^i⋅^k+3^j⋅^k+4^k⋅^k)
Since ^i⋅^k=0 and ^j⋅^k=0, we have:
Φ=B0L2(0+0+4)=4B0L2
Thus, the magnitude of the magnetic flux passing through the square is:
Φ=4B0L2 Weber
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTION BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION EXERCISE - very short answer
6 Videos
- ELECTROMAGNETIC INDUCTION BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION EXERCISE - Short answer type questions
5 Videos
- ELECTRIC CHARGES AND FIELDBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDEXERCISE - Long Answer Type Question
6 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - LONG ANSWER
5 Videos
Similar Questions
A square of side L meters lies in the x-y plane in a region, where the magnetic field is give by B=B0(2ˆi+3ˆj+4ˆk)T, where B0 is constant. The magnitude of flux passing through the square is
View SolutionA square of side L meters lies in the xy-plane in a region, where the magnetic field is given by =B0(2ˆi+3ˆj+4ˆk)T,, where B0 is constant. The magnitude of flux passing through the square is
View Solution
Knowledge Check
A square of side x m lies in the x-y plane in a region, when the magntic field is given by →B=B0(3ˆi+4ˆj+5ˆk) T, where B0 is constant. The magnitude of flux passing through the square is
A5B0x2WbB3B0x3WbC2B0x2WbDB0x2Wb
A cube has sides of length L. It is placed with one corner at the origin ( refer to Fig.2.119). The electric field is uniform and given by →€=−Bˆi+Cˆj−Dˆk , where B,C,andD are positive constants.
The total flux passing through the cube is
View SolutionThe electric field in a region is given by E=aˆi+bˆj. Hence as and b are constants. Find the net flux passing through a square area of side I parallel to y-z plane.
View SolutionThe electric field in a region is given by E=aˆi+bˆj. Hence as and b are constants. Find the net flux passing through a square area of side I parallel to y-z plane.
View SolutionA circular loop of radius R carrying current I is kept in XZ plane. A uniform and constant magnetic field →B=(B0ˆi+2B0ˆj+3B0ˆk) exists in the region (B0− a positive constant). Then the magnitude of the torque acting on the loop will be
View SolutionA square loop of side 'a' is placed in x−y plane as shown in figure. In this region there is non-uniform time dependent magnetic field →B=(cy3t2)→k, [where t is time and c is constant] then magnitude of emf induced in loop is
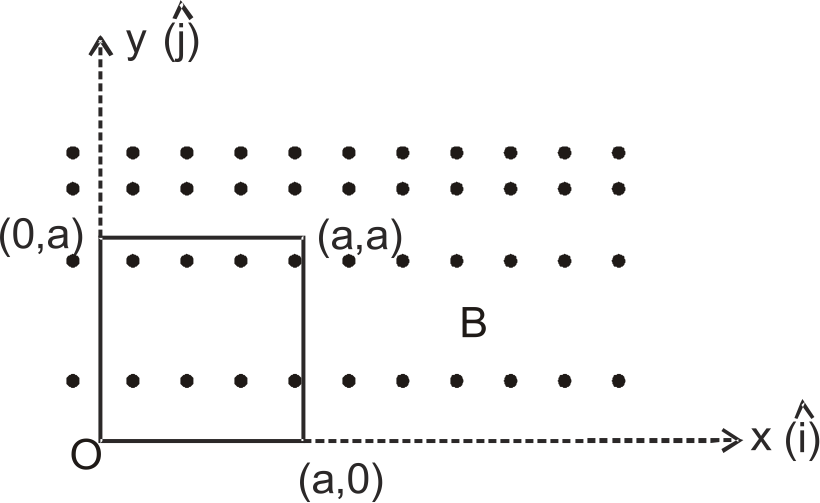
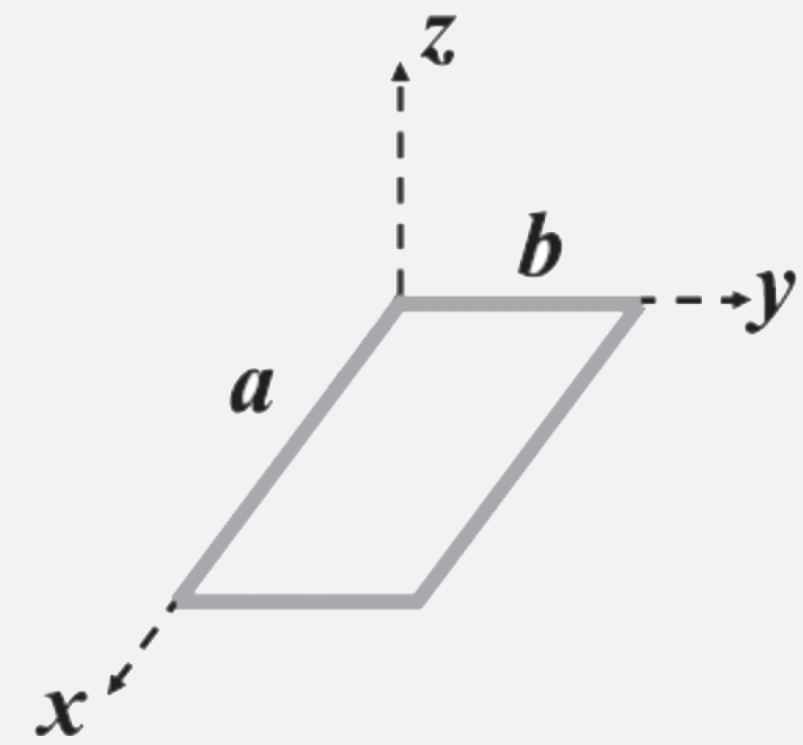
View SolutionA rectangular wire loop with length a and width b lies in the xy - plane as shown. Within the loop, there is a time dependent magnetic field given by →B=c[(xcosωt)ˆi+(ysinωt)ˆk]. Here, c and ω are constants. The magnitude of emf induced in the loop as a function of time is
View SolutionA wire of length a'a carries a current I along the y-axis.A magnetic field exists given by
B=B0(3ˆi+2ˆj+ˆk)T
Calculate magnetic force in vector from and its magnitude
View Solution
