A: At any instant, if the current through an inductor is zero, then the induced emf will also be zero.
R: In one time constant, the current flows to 37 percent of its maximum value in a series LR circuit.
If both Assertion & Reason are true and the reason is the correct explanation of the assertion.
If both Assertion & Reason are true but the reason is not the correct explanation of the assertion.
If Assertion is true statement but Reason is false.
If both Assertion and Reason are false statements.
The correct Answer is:D
To solve the assertion and reason question, we need to analyze both statements separately and determine their validity.
Step 1: Analyze the Assertion
The assertion states: "At any instant, if the current through an inductor is zero, then the induced emf will also be zero."
- Understanding Inductors: An inductor opposes changes in current. When the current through an inductor is zero, it means there is no steady current flowing through it.
- Induced EMF: The induced electromotive force (emf) in an inductor is given by Faraday's law of electromagnetic induction, which states that the induced emf (E) is equal to the negative rate of change of magnetic flux (Φ) through the inductor:
E=−dΦdt
- Conclusion: If the current is zero, it does not necessarily mean that the induced emf is zero. The induced emf can still exist if there is a change in magnetic flux. Therefore, the assertion is false.
Step 2: Analyze the Reason
The reason states: "In one time constant, the current flows to 37 percent of its maximum value in a series LR circuit."
- Time Constant in LR Circuit: In a series LR circuit, the current (I) at any time (t) can be expressed as:
I(t)=Imax(1−e−tτ)
where τ is the time constant.
- At One Time Constant: When t=τ:
I(τ)=Imax(1−e−1)≈Imax×0.632
This means that at one time constant, the current reaches approximately 63.2% of its maximum value, not 37%.
- Conclusion: Therefore, the reason is also false.
Final Conclusion
Both the assertion and the reason are false. Thus, the correct answer is that both statements are false.
The assertion states: "At any instant, if the current through an inductor is zero, then the induced emf will also be zero."
- Induced EMF: The induced electromotive force (emf) in an inductor is given by Faraday's law of electromagnetic induction, which states that the induced emf (E) is equal to the negative rate of change of magnetic flux (Φ) through the inductor:
E=−dΦdt
- Conclusion: If the current is zero, it does not necessarily mean that the induced emf is zero. The induced emf can still exist if there is a change in magnetic flux. Therefore, the assertion is false.
The reason states: "In one time constant, the current flows to 37 percent of its maximum value in a series LR circuit."
I(t)=Imax(1−e−tτ)
where τ is the time constant.
- At One Time Constant: When t=τ:
I(τ)=Imax(1−e−1)≈Imax×0.632
This means that at one time constant, the current reaches approximately 63.2% of its maximum value, not 37%.
- Conclusion: Therefore, the reason is also false.
Both the assertion and the reason are false. Thus, the correct answer is that both statements are false.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBook:AAKASH INSTITUTE ENGLISHChapter:ELECTROMAGNETIC INDUCTIONExercise:ASSIGNMENT(SECTION -C) Previous Years Questions
Explore 34 Videos
- ELECTRIC CHARGES AND FIELDS Book:AAKASH INSTITUTE ENGLISHChapter:ELECTRIC CHARGES AND FIELDS Exercise:comprehension
Explore 3 Videos
- ELECTROMAGNETIC WAVESBook:AAKASH INSTITUTE ENGLISHChapter:ELECTROMAGNETIC WAVESExercise:ASSIGNMENT SECTION - D Assertion-Reason Type Questions
Explore 25 Videos
Similar Questions
The current in an L−R circuit builds upto 3/4th of its steady state value in 4sec. Then the time constant of this circuit is
View SolutionA current i0 is flowing through an L−R circuit of time constant t0. The source of the current is switched off at time t=0. Let r be the value of (−di/dt) at time t=0.Assuming this rate to be constant, the current will reduce to zero in a time interval of
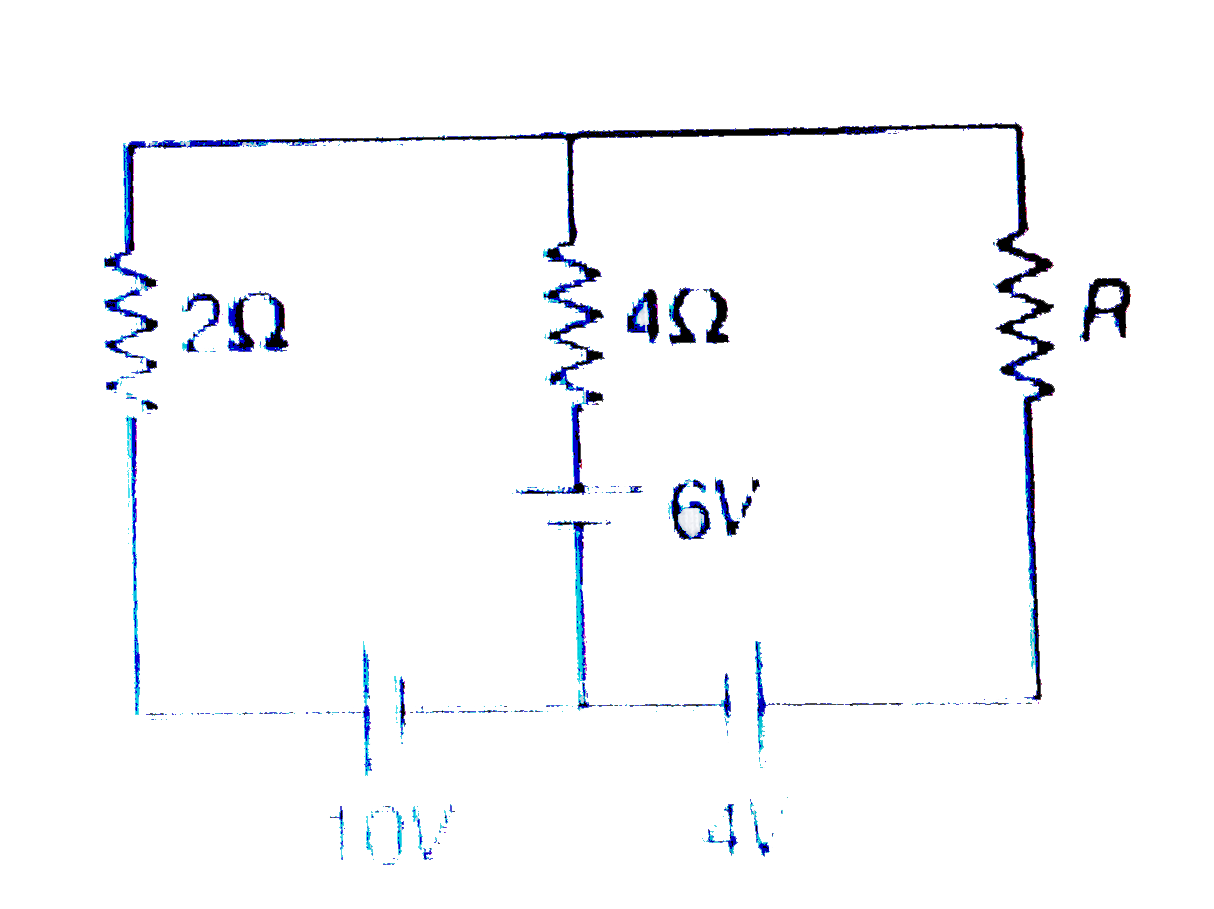
View SolutionFor what value of R in the circuit as shown, current passing through 4Ω resistance will be zero
View SolutionAn L−C circuit consists of an inductor with L=0.0900H and a capacitor of C=4x10−4F. The initial charge on the capacitor is 5.00μC, and the initial current in the inductor is zero.
(a) What is the maximum voltage across the capacitor?
(b) What is the maximum current in the inductor?
(c) What is the maximum energy stored in the inductor?
(d) When the current in the inductor has half its maximum value, what is the charge on the capacitor and what is the energy stored in the inductor?
View SolutionIn an LR circuit as shows in Fig.when the swtich is closed, how much time will it take for the current to grow to a value n times the maximum value of current (where n<1)?

View SolutionSwitch S of the circuit shows in Fig is closed at t=0. If e denotes the induced emf in L and I is the current flowing through the circuit at time t, which of the following graphs is//are correct?

View SolutionAn inductor of 3H is connected to a battery of emf 6V through a resistance of 100 Ω. Calculate the time constant. What will be the maximum value of current in the circuit ?
View SolutionAt the instant when the current in an inductor magnitude of the self-induced emf is 0.0640A/s, the magnitude fo the self- induced emf is 0.0160V.
(a) What is the inductance of the inductor?
(b) If the inductor is a solenoid with 400 turns, what is the average magnetic flux through each turn when the current is 0.720A?
View SolutionA simple LR circuit is connected to a battery at time t=0. The energy stored in the inductor reaches half its maximum value at time
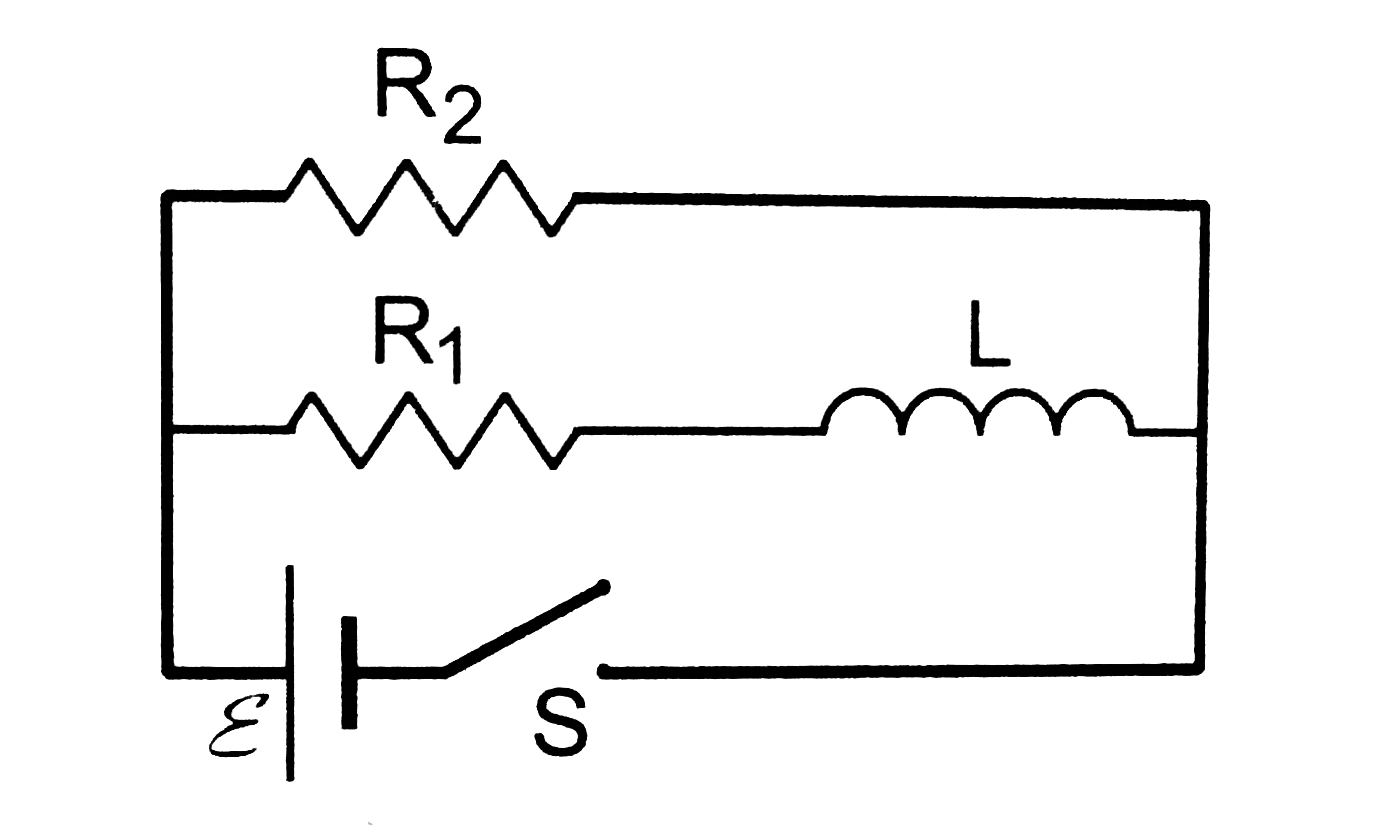
View SolutionConsider the circuit shown in figure.(a) find the current through the battery a long time after the switch S is closed. (b) Suppose the switch is again opened at t = 0. What is the time constant of the discharging circuit? (c ) Find t current through the inductor after one time constant.
View Solution