Question

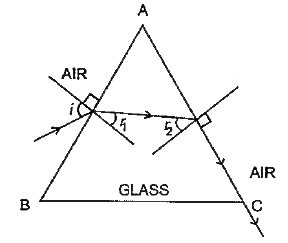
The angle at which a ray of light be incident on one face of an equilateral prism,so that the emergent ray may graze the second surface of the prism is ${\displaystyle \frac{\pi }{x}}$ rad.then find "x" .(${\displaystyle \mu =2}$)]

Options

A. `30^(@)`
B. `90^(@)`
C. `45^(@)`
D. `60^(@)`

Answer

B

Explanation

To solve the problem, we need to determine the angle of incidence on one face of an equilateral prism such that the emergent ray grazes the second surface of the prism. Given that the refractive index (μ) of the prism is 2, we can follow these steps:

1. Understand the Geometry of the Prism:
- An equilateral prism has angles of 60 degrees each. Therefore, the angle of the prism (A) is 60°.

2. Identify the Emergent Ray Condition:
- The emergent ray grazes the second surface of the prism. This means that at the point of emergence, the angle of refraction (r2) is 90°.

3. Determine the Critical Angle:
- The critical angle (C) can be found using Snell's law at the second surface of the prism:
\( \mu_1 \sin C = \mu_2 \sin 90° \)
- Here, μ1 = 2 (for the prism) and μ2 = 1 (for air). Therefore:
\( 2 \sin C = 1 \implies \sin C = \frac{1}{2} \implies C = 30° \)

4. Analyze the Angles Inside the Prism:
- At the first surface, let the angle of incidence be \(i\) and the angle of refraction be \(r_1\).
- The relationship between the angles at the first surface can be expressed as:
\( i + r_1 = A \implies i + r_1 = 60° \)
- Since the emergent ray grazes the second surface, we have:
\( r_2 = 90° \implies r_1 = C = 30° \)

5. Calculate the Angle of Incidence:
- Substitute \(r_1 = 30°\) into the equation:
\( i + 30° = 60° \implies i = 60° - 30° = 30° \)

6. Apply Snell's Law at the First Surface:
- Using Snell's law:
\( \mu_1 \sin i = \mu_2 \sin r_1 \)
- Substitute the values:
\( 2 \sin i = 1 \sin 30° \)
- Since \(\sin 30° = \frac{1}{2}\):
\( 2 \sin i = 1 \cdot \frac{1}{2} \implies \sin i = \frac{1}{4} \)
- Therefore, the angle of incidence \(i\) can be calculated as:
\( i = \arcsin\left(\frac{1}{4}\right) \)

7. Convert to Radians:
- The problem states that the angle of incidence is given as \(\frac{\pi}{x}\). Therefore, we need to find \(x\):
\( \frac{\pi}{x} = \arcsin\left(\frac{1}{4}\right) \)

8. Find the Value of x:
- To find \(x\), we can rearrange the equation:
\( x = \frac{\pi}{\arcsin\left(\frac{1}{4}\right)} \)

Final Answer:
The value of \(x\) can be approximated using a calculator:
\( x \approx 7.64 \)