Four waves are expressed as
1. y1=a1sinωt
2. y2=a2sin2ωt
3. y3=a3cosωt
4. y4=a4sin(ωt+ϕ)
The interference is possible between
(i) and (iii)
(i) and (ii)
(ii) and (iv)
Not possible at all
The correct Answer is:A
To determine which waves can interfere with each other, we need to analyze the angular frequencies of the given waves. Interference is possible only when the waves have the same angular frequency.
1. Identify the Angular Frequencies:
- For the waves given:
- y1=a1sin(ωt) has angular frequency ω.
- y2=a2sin(2ωt) has angular frequency 2ω.
- y3=a3cos(ωt) has angular frequency ω.
- y4=a4sin(ωt+ϕ) has angular frequency ω.
2. Compare Angular Frequencies:
- Waves y1, y3, and y4 all have the same angular frequency ω.
- Wave y2 has a different angular frequency 2ω.
3. Determine Possible Interference:
- Between y1 and y3:
- Both have angular frequency ω. They can interfere.
- Between y1 and y2:
- y1 has ω and y2 has 2ω. They cannot interfere.
- Between y2 and y3:
- y2 has 2ω and y3 has ω. They cannot interfere.
- Between y2 and y4:
- y2 has 2ω and y4 has ω. They cannot interfere.
- Between y3 and y4:
- Both have angular frequency ω. They can interfere.
4. Conclusion:
- The waves that can interfere are:
- y1 and y3
- y1 and y4
- y3 and y4
Final Answer:
Interference is possible between:
- y1 and y3
- y1 and y4
- y3 and y4
- For the waves given:
- y1=a1sin(ωt) has angular frequency ω.
- y2=a2sin(2ωt) has angular frequency 2ω.
- y3=a3cos(ωt) has angular frequency ω.
- y4=a4sin(ωt+ϕ) has angular frequency ω.
- Waves y1, y3, and y4 all have the same angular frequency ω.
- Wave y2 has a different angular frequency 2ω.
- Between y1 and y3:
- Both have angular frequency ω. They can interfere.
- Between y1 and y2:
- y1 has ω and y2 has 2ω. They cannot interfere.
- Between y2 and y3:
- y2 has 2ω and y3 has ω. They cannot interfere.
- Between y2 and y4:
- y2 has 2ω and y4 has ω. They cannot interfere.
- Between y3 and y4:
- Both have angular frequency ω. They can interfere.
- The waves that can interfere are:
- y1 and y3
- y1 and y4
- y3 and y4
Interference is possible between:
- y1 and y3
- y1 and y4
- y3 and y4
Similar Questions
For different independent waves are represented by
a) Y1=a1sinω1t , b) Y2=a2sinω2t
c) Y3=a3sinω3t , d) Y4=a4sin(ω4t+π3)
The sustained interference is possible between which two waves ?
View SolutionTwo SHM's are represented by y1=Asin(ωt+ϕ),y2=A2[sinωt+√3cosωt]. Find ratio of their amplitudes.
View SolutionFour sound sources produce the following four waves
(i) y1=asin(ωt+ϕ1)
(ii) y2=asin2ωt
(iii) y3=a'sin(ωt+ϕ2)
(iv) y4=a'sin(3ωt+ϕ)
Superposition of which two waves gives rise to interference?
View SolutionIf i1=3sinωtand(i2)=4cosωt, then (i3) is

View SolutionFour simple harmonic vibrations
y1=8sinωt, y2=6sin(ωt+π/2), y3=4sin(ωt+π), y4=2sin(ωt+3π/2)
are susperimposed on each other. The resulting amplitude and phase are respectively.
View SolutionIn y= A sin ωt + A sin (ωt+(2π)/3) match the following table.
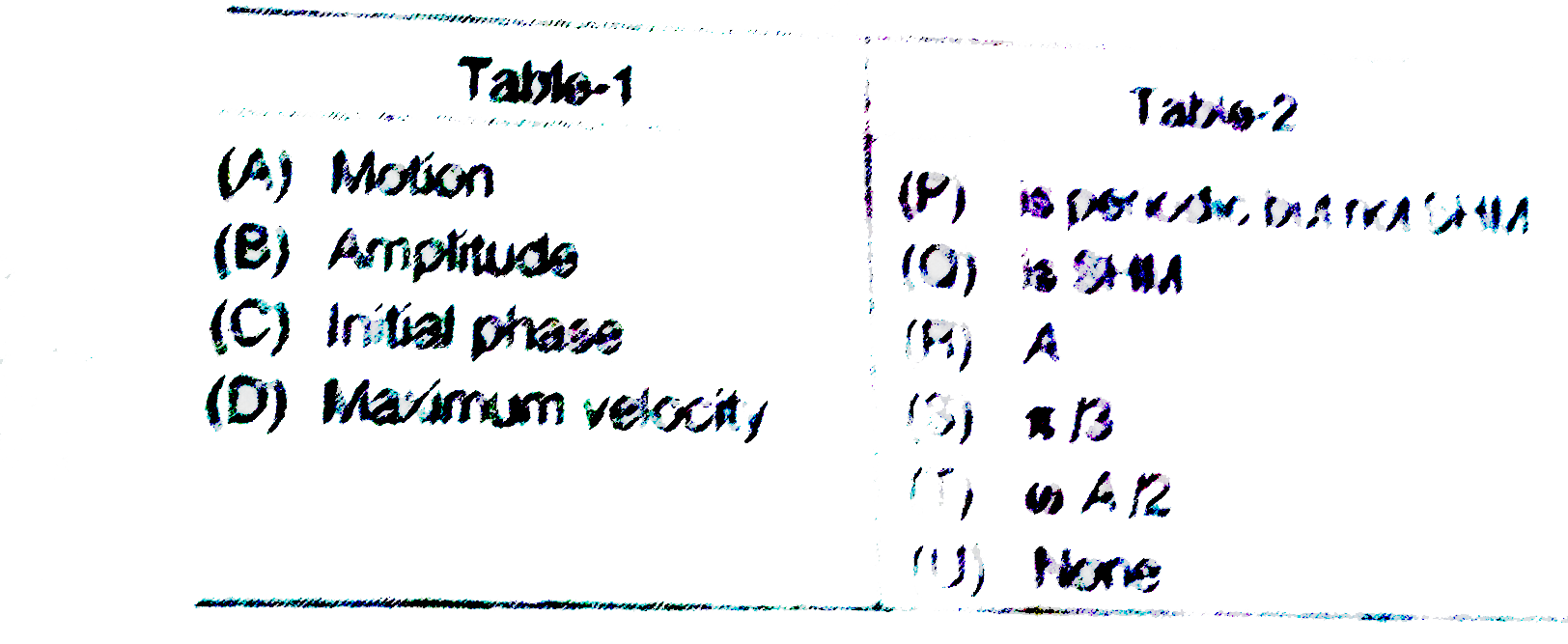
View SolutionTwo waves are represented by the equations
y1=asin(ωt+kx+0.785)
and y2=acos(ωt+kx)
where, x is in meter and t in second
The phase difference between them and resultant amplitude due to their superposition are
View Solutionx1=5sinωt
x2=5sin(ωt+53∘)
x3=−10cosωt
Find amplitude of resultant SHM.
View Solutionx1=5sinωt
x2=5sin(ωt+53∘)
x3=−10cosωt
Find amplitude of resultant SHM.
View SolutionA particule moves in x - y plane acording to rule x = a sin ωt and y = a cos ωt. The particle follows
View Solution
