For a reaction of type
aA+bB→products,−d[A]dt is equal to
−d[B]dt
−bdd[B]dt
−ab×d[B]dt
−ba×d[B]dt
The correct Answer is:C
To solve the problem, we need to express the rate of change of concentration of reactant A in terms of the rate of change of concentration of reactant B. The reaction is given as:
aA+bB→products
1. Write the Rate Expression:
The rate of the reaction can be expressed in terms of the change in concentration of A and B. The rate of disappearance of A can be written as:
−d(A)dt=1ad(A)dt
Similarly, for B, it can be expressed as:
−d(B)dt=1bd(B)dt
2. Relate the Rates:
From the stoichiometry of the reaction, we can relate the rates of change of A and B:
−1ad(A)dt=−1bd(B)dt
3. Rearranging the Equation:
Rearranging the above equation gives us:
d(A)dt=−abd(B)dt
4. Final Expression:
Thus, we can express the rate of change of concentration of A as:
−d(A)dt=abd(B)dt
5. Conclusion:
Therefore, the expression for −d(A)dt in terms of −d(B)dt is:
−d(A)dt=−abd(B)dt
The rate of the reaction can be expressed in terms of the change in concentration of A and B. The rate of disappearance of A can be written as:
−d(A)dt=1ad(A)dt
Similarly, for B, it can be expressed as:
−d(B)dt=1bd(B)dt
From the stoichiometry of the reaction, we can relate the rates of change of A and B:
−1ad(A)dt=−1bd(B)dt
Rearranging the above equation gives us:
d(A)dt=−abd(B)dt
Thus, we can express the rate of change of concentration of A as:
−d(A)dt=abd(B)dt
Therefore, the expression for −d(A)dt in terms of −d(B)dt is:
−d(A)dt=−abd(B)dt
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - ASSIGNMENT (SECTION B : Objective Type Questions)
20 Videos
- CHEMICAL KINETICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - ASSIGNMENT (SECTION C : Previous Year Questions)
62 Videos
- CHEMICAL KINETICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - EXERCISE
30 Videos
- CHEMICAL BONDING AND MOLECULAR STRUCTURE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTURE EXERCISE - Assignment Section J (Aakash Challengers Questions)
10 Videos
- CHEMISTRY IN EVERYDAY LIFE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CHEMISTRY IN EVERYDAY LIFE EXERCISE - Assignment ( SECTION - A)
45 Videos
Similar Questions
Knowledge Check
At any stage of the reaction 3A →2B, the reaction rate +dBdt will be equal to
A−3dAdtB−dAdtC−23dAdtD−32dAdtAt any stage of the reaction 3A →2B, the reaction rate +dBdt will be equal to
A−3dAdtB−dAdtC−23dAdtD−32dAdtAt any stage of the reaction 3A →2B, the reaction rate +dBdt will be equal to
A−3dAdtB−dAdtC−23dAdtD−32dAdt
2A+2C→2B, rate of reaction +d(B)dt is equal to
View SolutionIn the reaction , A+2B→6C+2D, if the initial rate −d[A]dt at t= 0 is 2.6×10−2Msec−1, what will be the value of −d[B]dt at t=0?
View SolutionThe graph between concentration (X) of the Product and time of the reaction A→B is of the type 1. Hence, graph between −d[A]dt and time will be of the type:
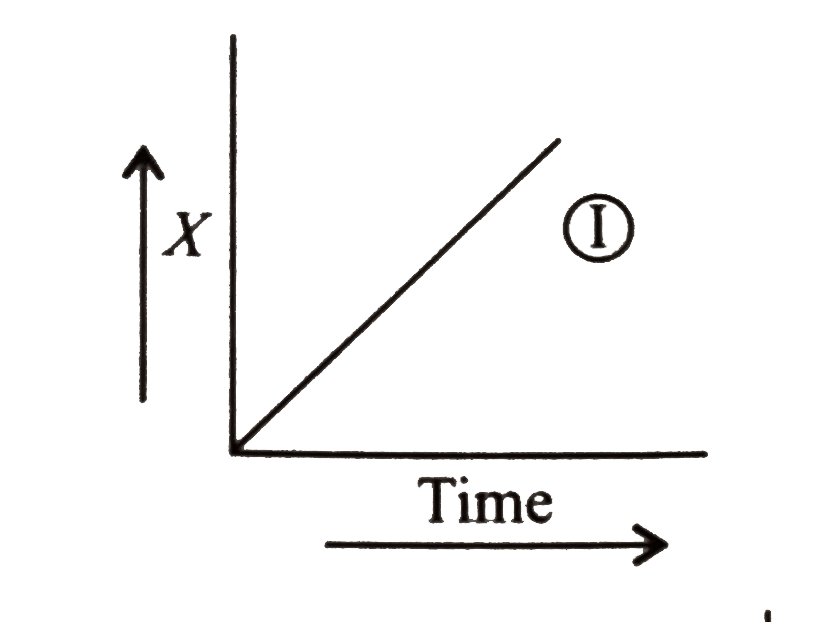
View SolutionThe graph between concentration (X) of the Product and time of the reaction A→B is of the type 1. Hence, graph between −d[A]dt and time will be of the type:

View SolutionFor the reaction : aA→bB, it is given that
log[−dAdt]=log[dBdt]+0.6020. What is a:b is ?
View Solution
