Two sources A and B are sounding notes of frequency 660 Hz. A listener moves from A to B with a constant velocity u. If the speed is 330 m/s , what must be the value of u so that he hears 8 beats per second ?
2.8 m/s
2 m/
3.0 m/s
3.5 m/s
The correct Answer is:B
To solve the problem, we need to determine the speed u of the listener moving from source A to source B, given that both sources emit sound at a frequency of 660 Hz and the listener hears 8 beats per second. The speed of sound is given as 330 m/s.
1. Understanding the Beat Frequency:
The beat frequency fbeat is the difference between the frequencies heard from the two sources. In this case, we have:
fbeat=|f′B−f′A|
where f′B is the frequency heard from source B and f′A is the frequency heard from source A.
2. Using the Doppler Effect:
We will apply the Doppler effect formula to find the apparent frequencies f′A and f′B:
- For source A (the listener is moving away):
f′A=f(vv−u)
Here, f=660Hz, v=330m/s, and u is the speed of the listener.
f′A=660(330330−u)
- For source B (the listener is moving towards):
f′B=f(vv+u)
f′B=660(330330+u)
3. Setting Up the Beat Frequency Equation:
We know the beat frequency is 8 Hz:
|f′B−f′A|=8
Substituting the expressions for f′A and f′B:
∣∣∣660(330330+u)−660(330330−u)∣∣∣=8
4. Simplifying the Equation:
Factor out 660:
660∣∣∣330330+u−330330−u∣∣∣=8
Dividing both sides by 660:
∣∣∣330330+u−330330−u∣∣∣=8660
Simplifying 8660=2165.
5. Finding a Common Denominator:
The common denominator for the fractions is (330+u)(330−u):
∣∣∣330(330−u)−330(330+u)(330+u)(330−u)∣∣∣=2165
Simplifying the numerator:
330(330−u−330−u)=−660u
Thus, we have:
∣∣∣−660u(330+u)(330−u)∣∣∣=2165
6. Cross-Multiplying:
Cross-multiplying gives:
165⋅660|u|=2⋅(330+u)(330−u)
Expanding the right-hand side:
3302−u2
7. Solving for u:
After simplifying and solving the equation, we find:
4u=8⟹u=2m/s
Final Answer:
The value of u must be 2m/s.
The beat frequency fbeat is the difference between the frequencies heard from the two sources. In this case, we have:
fbeat=|f′B−f′A|
where f′B is the frequency heard from source B and f′A is the frequency heard from source A.
We will apply the Doppler effect formula to find the apparent frequencies f′A and f′B:
- For source A (the listener is moving away):
f′A=f(vv−u)
Here, f=660Hz, v=330m/s, and u is the speed of the listener.
f′A=660(330330−u)
f′B=f(vv+u)
f′B=660(330330+u)
We know the beat frequency is 8 Hz:
|f′B−f′A|=8
Substituting the expressions for f′A and f′B:
∣∣∣660(330330+u)−660(330330−u)∣∣∣=8
Factor out 660:
660∣∣∣330330+u−330330−u∣∣∣=8
Dividing both sides by 660:
∣∣∣330330+u−330330−u∣∣∣=8660
Simplifying 8660=2165.
The common denominator for the fractions is (330+u)(330−u):
∣∣∣330(330−u)−330(330+u)(330+u)(330−u)∣∣∣=2165
Simplifying the numerator:
330(330−u−330−u)=−660u
Thus, we have:
∣∣∣−660u(330+u)(330−u)∣∣∣=2165
Cross-multiplying gives:
165⋅660|u|=2⋅(330+u)(330−u)
Expanding the right-hand side:
3302−u2
After simplifying and solving the equation, we find:
4u=8⟹u=2m/s
The value of u must be 2m/s.
Topper's Solved these Questions
- WAVESBook:AAKASH INSTITUTE ENGLISHChapter:WAVESExercise:ASSIGNMENT ( SECTION-A ( Objective type Questions ))
Explore 50 Videos
- WAVESBook:AAKASH INSTITUTE ENGLISHChapter:WAVESExercise:ASSIGNMENT ( SECTION-B ( Objective type Questions ))
Explore 45 Videos
- WAVESBook:AAKASH INSTITUTE ENGLISHChapter:WAVESExercise:Assignment (Section-J) (Paragraph Type Questions)
Explore 4 Videos
- WAVE OPTICSBook:AAKASH INSTITUTE ENGLISHChapter:WAVE OPTICSExercise:Assignment (Section-J (Aakash Challengers question))
Explore 1 Video
- WORK, ENERGY AND POWERBook:AAKASH INSTITUTE ENGLISHChapter:WORK, ENERGY AND POWERExercise:Assignment (SECTION - D)
Explore 13 Videos
Similar Questions
Two sources A and B are sounding notes of frequency 680 Hz. A listener moves from A to B with a constant velocity u. If the speed of sound is 340 m/s, What must be the value of u so that he hears 10 beats per second?
View SolutionTwo sources of sound S1 and S2 produce sound waves of same frequency 660Hz. A listener is moving from source S1 towards S2 with a constant speed um/s and he hears 10 beats/s. The velocity of sound is 330m/s. Then, u equals :
View SolutionA and B are two identical sound sources cachemitting a note of frequency 1000 Hz. A person moves from A to B along the line joining the two sources. How fast should be move to that he has 7 beats per second. velocity of sound 330 m/s ?
View SolutionA source of sound of frequency 500 Hz is moving towards an observer with velocity 30 m/s . The speed of sound is 330 m/s . the frequency heard by the observer will be
View Solution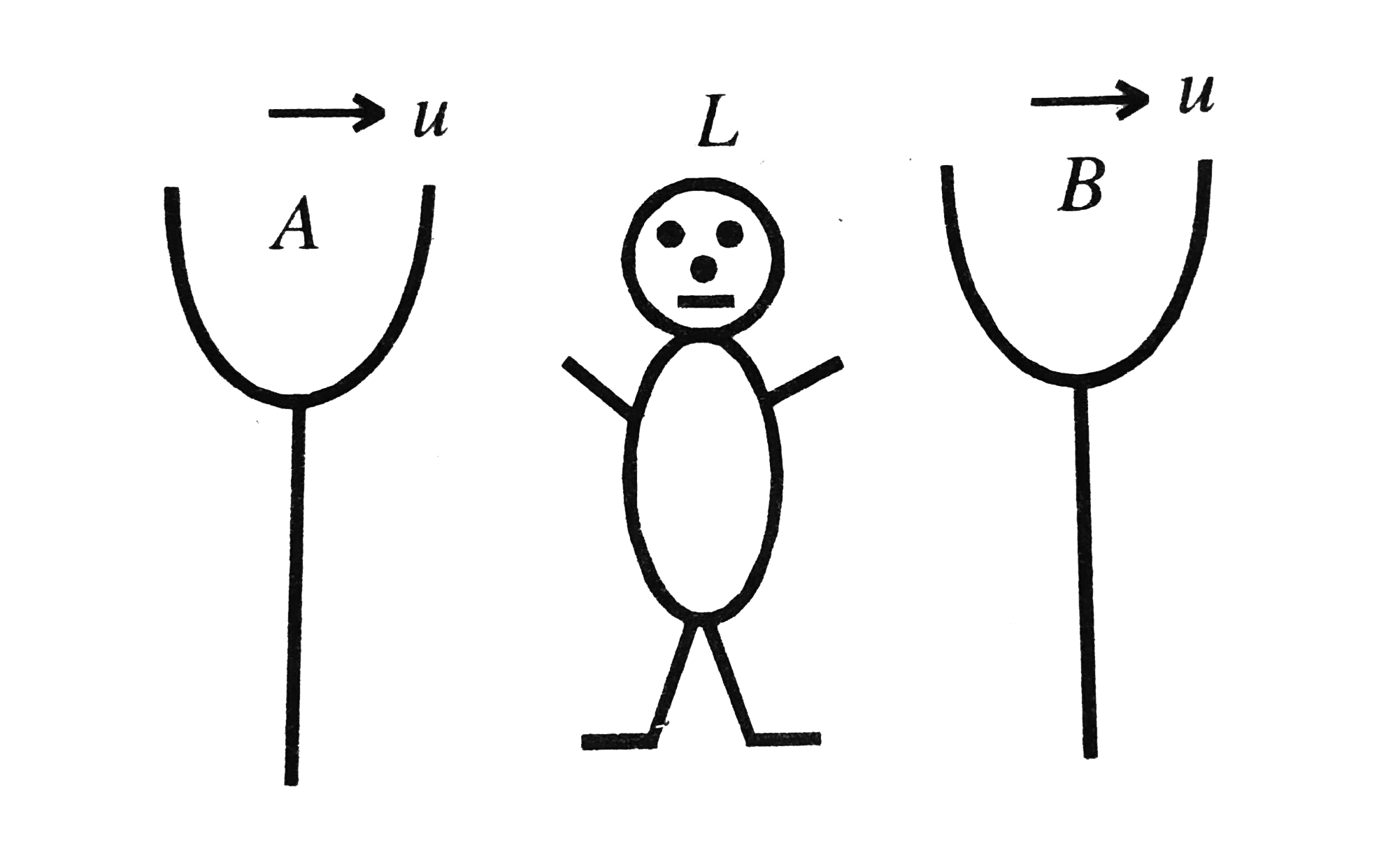 Two tuning forks A and B are vibrating at the same frequency 256 Hz. A listener is standing midway between the forks. If both tuning forks move to the right with a velocity of 5ms, find the number of beats heard per second by the listerner (speed of sound in air is 330ms).
Two tuning forks A and B are vibrating at the same frequency 256 Hz. A listener is standing midway between the forks. If both tuning forks move to the right with a velocity of 5ms, find the number of beats heard per second by the listerner (speed of sound in air is 330ms).
View Solution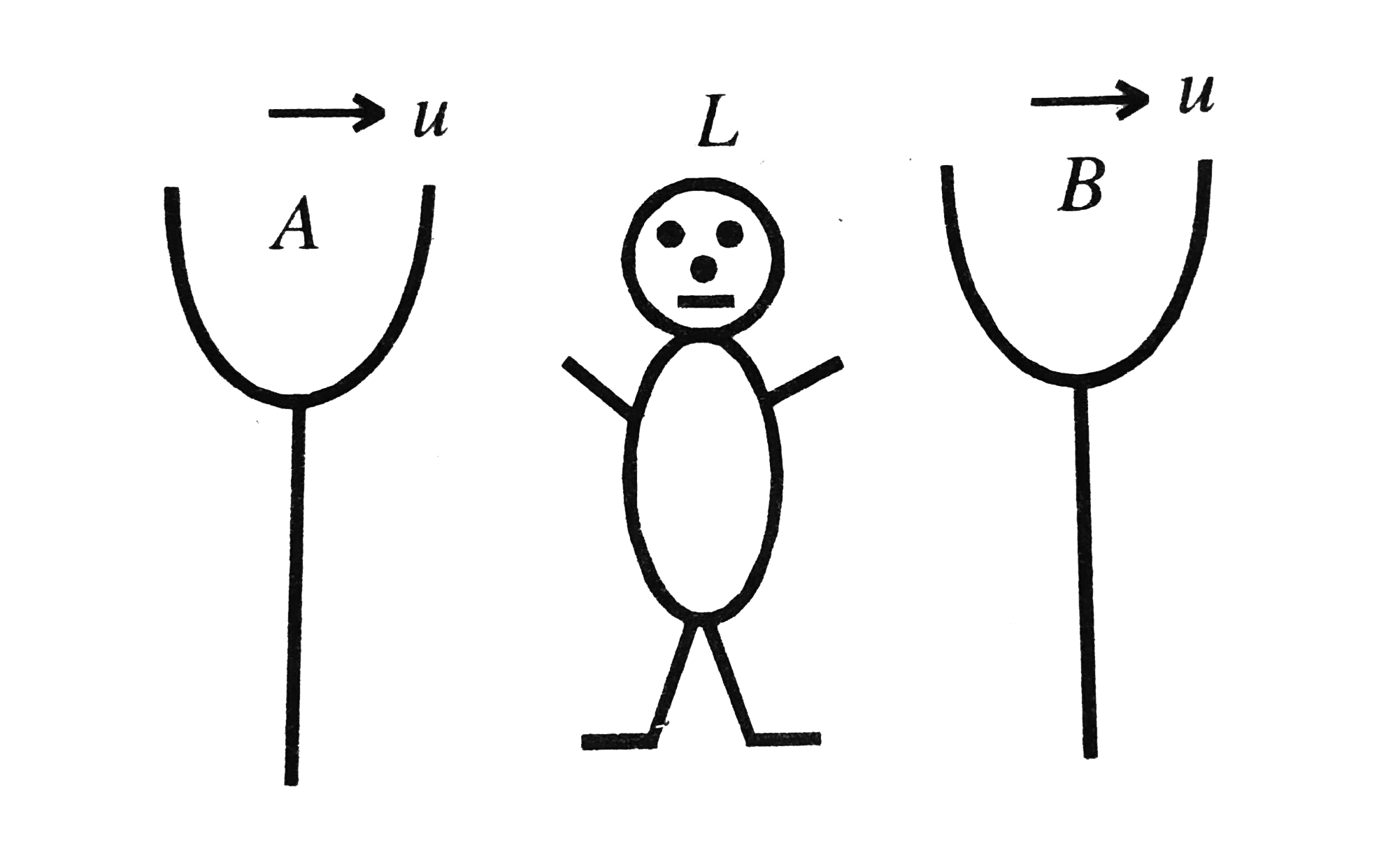 Two tuning forks A and B are vibrating at the same frequency 256 Hz. A listener is standing midway between the forks. If both tuning forks move to the right with a velocity of 5ms, find the number of beats heard per second by the listerner (speed of sound in air is 330ms).
Two tuning forks A and B are vibrating at the same frequency 256 Hz. A listener is standing midway between the forks. If both tuning forks move to the right with a velocity of 5ms, find the number of beats heard per second by the listerner (speed of sound in air is 330ms).
View SolutionA tuning fork of frequency 256hZ is moving towards a well with a velocity of 5m/s . If the speed of sound is 330m/s , then the number of beats heard per second by a stationary observer lying between tuning fork and the well is
View SolutionA siren emitting a sound of frequency 2000 Hz moves away from you towards a cliff at a speed of 8 m/s.
(a) What is the frequency of the sound you hear coming directly from the siren.
(b) What is the frequency of sound you hear reflected off the cliff. Speed of sound in air is 330ms.
View SolutionA source of sound of frequency 256Hz is moving rapidly towards wall with a velocity of 5m/sec. How many beats per second will be heard if sound travels at a speed of 330m/sec.
View SolutionTwo sound sources emitting sound each of wavelength λ are fixed at a given distance apart. A listener moves with a velocity u along the line joining the two sources. The number of beats heard by him per second is
View Solution
