Thousand cells of same emf E and same internal resistance r are connected is series in same order without an external resistance . The potential drop across 399 cells is found to be .
Zero
399E
601E
1000E
The correct Answer is:A
To solve the problem step by step, we will analyze the situation involving the cells connected in series.
Step 1: Understand the Configuration
We have 1000 cells connected in series. Each cell has the same electromotive force (emf) E and the same internal resistance r.
Hint: Remember that when cells are connected in series, the total emf and total internal resistance can be calculated by summing the individual emfs and resistances.
Step 2: Calculate Total emf and Total Resistance
The total emf Etotal of the series connection is:
Etotal=1000E
The total internal resistance Rtotal of the series connection is:
Rtotal=1000r
Hint: The total emf is the sum of the individual emfs, and the total resistance is the sum of the individual resistances.
Step 3: Determine the Current in the Circuit
Using Ohm's Law, the current I flowing through the circuit can be calculated as:
I=EtotalRtotal=1000E1000r=Er
Hint: The current can be found by dividing the total voltage by the total resistance.
Step 4: Calculate the Potential Drop Across One Cell
The potential drop V across one cell can be calculated using the formula:
V=E−I⋅r
Substituting the value of I:
V=E−(Er)⋅r=E−E=0
Hint: The potential drop across a cell can be found by subtracting the voltage drop due to the internal resistance from the emf of the cell.
Step 5: Determine the Potential Drop Across 399 Cells
Since the potential drop across each cell is 0, the potential drop across 399 cells is:
V399=399⋅0=0
Hint: If the potential drop across one cell is zero, it will also be zero for any number of cells in series.
Conclusion
Thus, the potential drop across 399 cells is 0.
Final Answer: The potential drop across 399 cells is 0.
We have 1000 cells connected in series. Each cell has the same electromotive force (emf) E and the same internal resistance r.
The total emf Etotal of the series connection is:
Etotal=1000E
The total internal resistance Rtotal of the series connection is:
Rtotal=1000r
Using Ohm's Law, the current I flowing through the circuit can be calculated as:
I=EtotalRtotal=1000E1000r=Er
The potential drop V across one cell can be calculated using the formula:
V=E−I⋅r
Substituting the value of I:
V=E−(Er)⋅r=E−E=0
Since the potential drop across each cell is 0, the potential drop across 399 cells is:
V399=399⋅0=0
Thus, the potential drop across 399 cells is 0.
Similar Questions
Two cells of the same e.mf. e but different internal resistances r1andr2 are connected in series with an external resistance 'R'. The potential drop across the first cell is found to be zero. The external resistance Ris
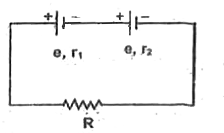
View SolutionA cell of e.mf. E and internal resistance r is connected in series with an external resistance nr. Then, the ratio of the terminal potential difference to E.M.F.is
View Solution
Knowledge Check
If n cells each of emf E and internal resistance rare connected in parallel, then the total emf and internal resistances will be
Aε,rnBε,nrCnε,rnDnε,nr
A capacitor is conneted to a cell emf E having some internal resistance r. The potential difference across the
View SolutionTwo cells of emf E each and internal resistance r1 and r2 are connected in series across a load resistance R. If potential difference across the first cell is zero, then find the relation between R,r1 and r2
View SolutionTwo cells of emf E each and internal resistance r1 and r2 are connected in series across a load resistance R. If potential difference across the first cell is zero, then find the relation between R,r1 and r2
View SolutionA cell of emf E and internal resistance r is connected in series with an external resistance nr. Than what will be the ratio of the terminal potential difference to emf, if n=9.
View SolutionFour cells, each of emf E and internal resistance r, are connected in series across an external resistance R. By mistake one of the cells is connected in reverse. Then, the current in the external circuit is
View SolutionThere are n cells, each of emf E and internal resistance r, connected in series with an external resistance R. One of the cells is wrongly connected, so that it sends current in the opposite direction. The current flowing in the circuit is
View Solution24 cells, each of emf 1.5V and internal resistance is 2Ω connected to 12Ω series external resistance. Then,
View Solution
