A : In earn may nave even reversea the direction of its field several time during its history of 4 to 5 billion years.
R: Earth's magnetic field gets weakly' recorded' in certain rocks during solidification.
If both Assertion & Reason are the true and the reason is the correct explanation of the assertion, then mark (1)
If both Assertion & Reason are true but the reson is not the correct explanation of the assertion, then mark (2)
If Assertion is true statement but Reason is false, then mark (3)
If both Assertion and Reason are false statements, then mark (4)
The correct Answer is:A
To analyze the given statements about Earth's magnetic field and its historical reversals, we can break down the reasoning step by step.
1. Understanding the Assertion:
- The assertion states that the Earth may have reversed the direction of its magnetic field several times during its history of 4 to 5 billion years. This phenomenon is known as "geomagnetic reversal."
- Geomagnetic reversals are documented occurrences where the magnetic north and south poles switch places.
2. Understanding the Reason:
- The reason provided states that Earth's magnetic field gets weakly recorded in certain rocks during their solidification.
- When magma cools and solidifies into rock, magnetic minerals within the rock can align themselves with the Earth's magnetic field at that time. This creates a record of the magnetic orientation at the time of solidification.
3. Connecting Assertion and Reason:
- The assertion is supported by the reason because the recording of the magnetic field in rocks allows scientists to study the history of geomagnetic reversals.
- By examining the orientation of magnetic minerals in various rock layers, researchers can determine when reversals occurred and how often they have happened over geological time.
4. Conclusion:
- Both the assertion and the reason are correct. The assertion accurately describes the phenomenon of geomagnetic reversal, and the reason explains how this phenomenon can be studied through geological records.
Final Answer:
Both assertion (A) and reason (R) are correct, and R explains A.
---
- The assertion states that the Earth may have reversed the direction of its magnetic field several times during its history of 4 to 5 billion years. This phenomenon is known as "geomagnetic reversal."
- Geomagnetic reversals are documented occurrences where the magnetic north and south poles switch places.
- The reason provided states that Earth's magnetic field gets weakly recorded in certain rocks during their solidification.
- When magma cools and solidifies into rock, magnetic minerals within the rock can align themselves with the Earth's magnetic field at that time. This creates a record of the magnetic orientation at the time of solidification.
- The assertion is supported by the reason because the recording of the magnetic field in rocks allows scientists to study the history of geomagnetic reversals.
- By examining the orientation of magnetic minerals in various rock layers, researchers can determine when reversals occurred and how often they have happened over geological time.
- Both the assertion and the reason are correct. The assertion accurately describes the phenomenon of geomagnetic reversal, and the reason explains how this phenomenon can be studied through geological records.
Both assertion (A) and reason (R) are correct, and R explains A.
Topper's Solved these Questions
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION C)
34 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-D) (Assertion-Reason Type Questions)
15 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - J
9 Videos
Similar Questions
The figure shows a circular loop of radius a with two long parallel wires (νmbered1and2) all in the plane of the paper . The distance of each wire from the centre of the loop is d. The loop and the wire are carrying the same current I. The current in the loop is in the counterclockwise direction if seen from above.
(q) The magnetic fields(B) at P due to the currents in the wires are in opposite directions.
(r) There is no magnetic field at P.
(s) The wires repel each other.
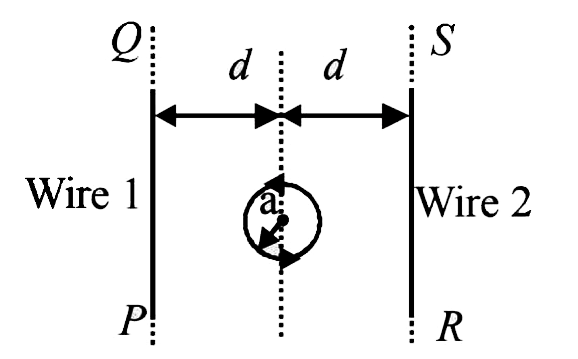
(5) Consider d>>a, and the loop is rotated about its diameter parallel to the wires by 30∘ from the position shown in the figure. If the currents in the wire are in the opposite directions, the torque on the loop at its new position will be ( assume that the net field due to the wires is constant over the loop).
View SolutionThe earth's magnetic field at geomagnetic poles has a magnitude 8×10−5T. Find the magnitude and the direction of the field at a point on the earth's surface where the radius makes an angle of 120∘ with its axis of the earth's assumed magnetic dipole. What is the inclination dip at this point?
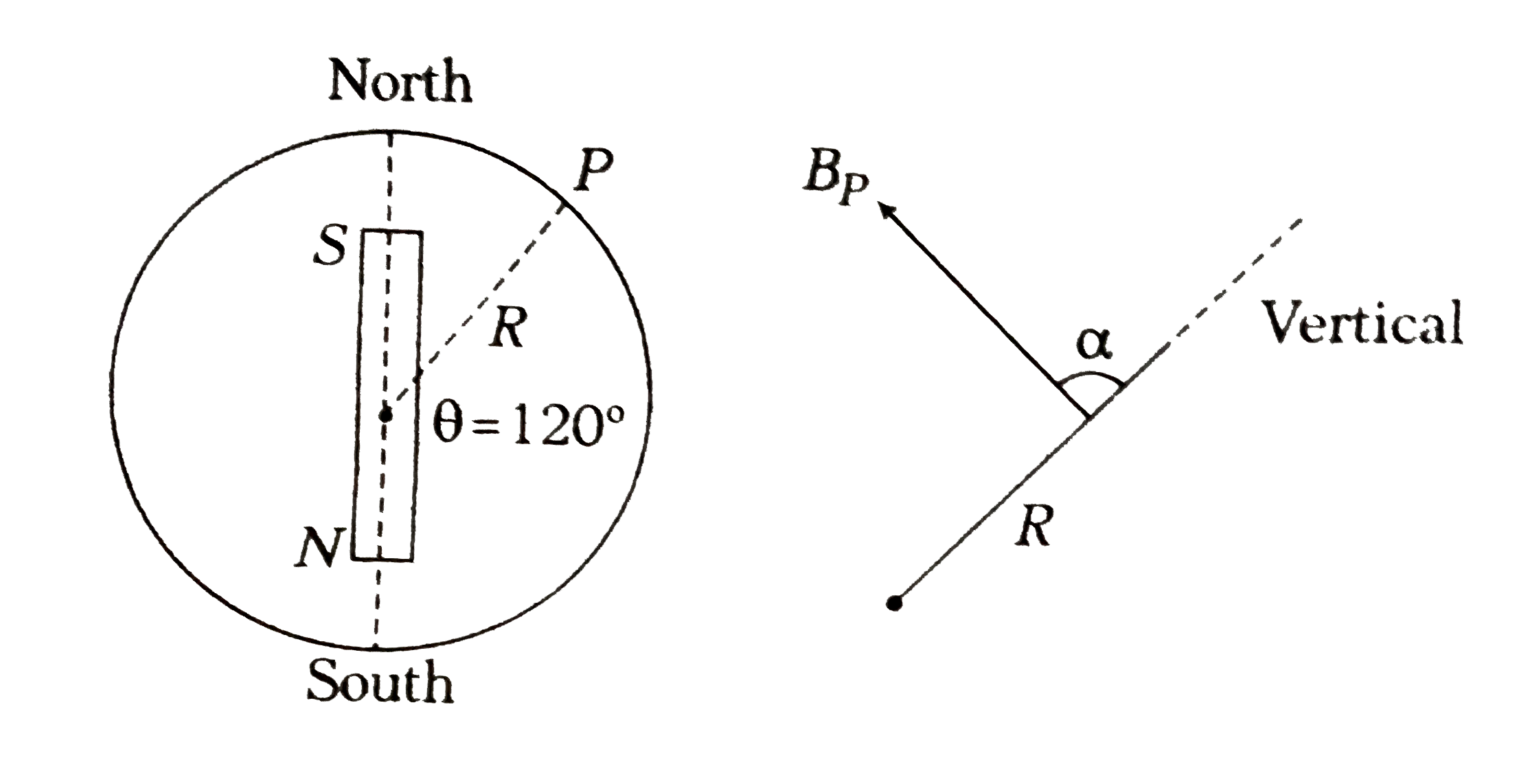
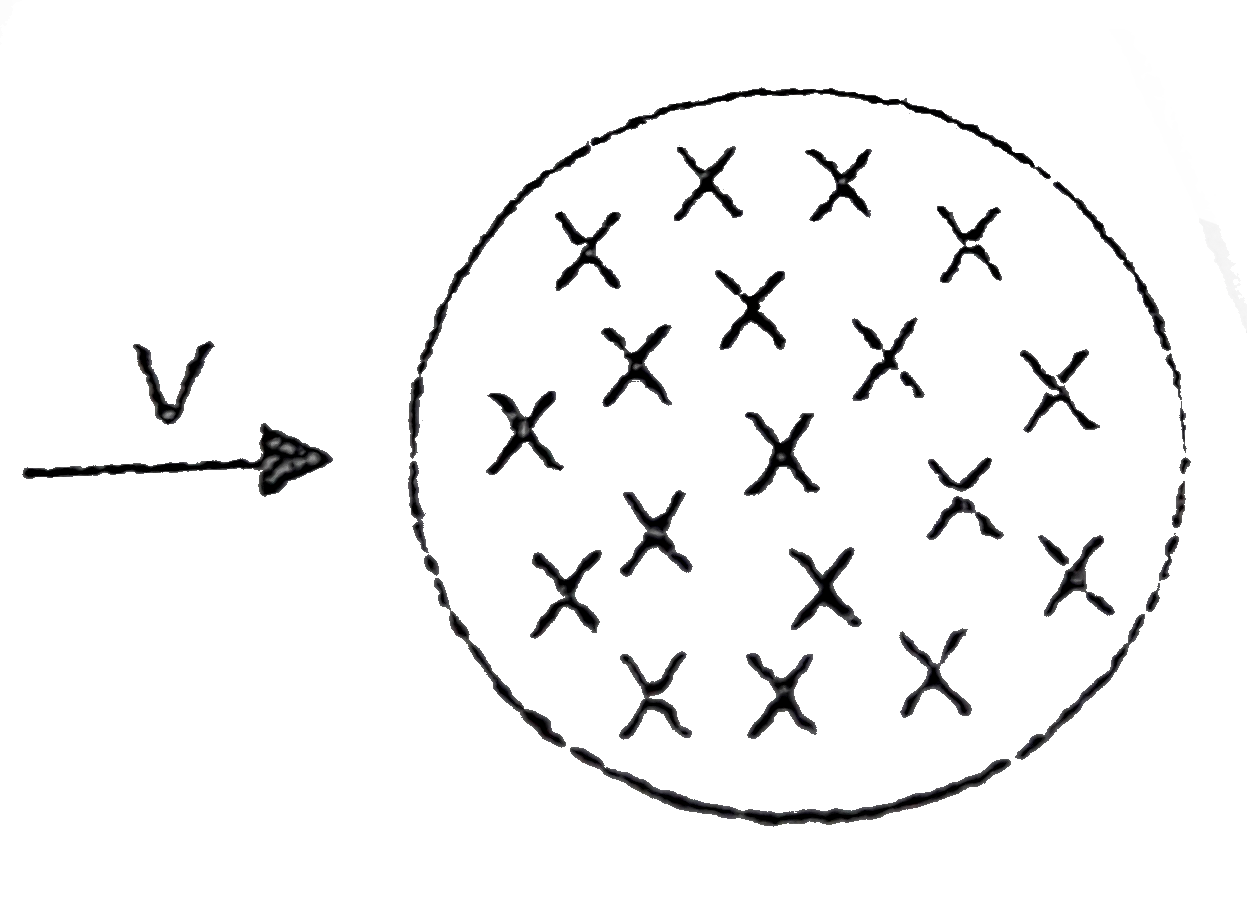
View SolutionA uniform magnetic field exists in a circular region of radius r.The magnitude of magnetic field is B and points inward.An electron flies into the region radially as shown in figure.After a certain time, the electron deflected by the magnetic field leaves the region.The time interval during which the electron moves in the region is Nmtan−1eBrmVeB.Then find value of N.(m is mass of electron and e is charge of electron)
View SolutionPQRS is a square region of side 2a in the plane of paper. A uniform magnetic field B, directed perpendicular to the plane of paper and into its plane is confined within this square region. A square loop of side 'a' and made of a conducting wire of resistance R is moved at a constant velocity →v from left to right in the plane of paper as shown. Obviously, the square loop will enter the magnetic field at some time and then leave it after some time. During the motion of loop, whenever magnetic flux through it changes, emf will be induced resulting in induced current. Let the motion of the square loop be along x-axis and let us measure x coordinate of the centre of square loop from the centre of the square magnetic field region (taken as origin). Thus, x coordinate will be positive if the centre of square loop is to the right of the origin O (centre of magnetic field) and negative if centre is to the left.

For x=−9a/5, magnitude of induced current and its direction as seen from above will be:
View SolutionA stationary circular loop of radius a is located in a magnetic field which varies with time from t = 0 to t = T according to law B=B0t(T−t). If plane of loop is normal to the direction of field and resistance of the loop is R, calculate
magnitude of charge flown through the loop from instant t = 0 to the instant when current reverses its direction.
View SolutionPQRS is a square region of side 2a in the plane of paper. A uniform magnetic field B, directed perpendicular to the plane of paper and into its plane is confined within this square region. A square loop of side 'a' and made of a conducting wire of resistance R is moved at a constant velocity →v from left to right in the plane of paper as shown. Obviously, the square loop will enter the magnetic field at some time and then leave it after some time. During the motion of loop, whenever magnetic flux through it changes, emf will be induced resulting in induced current. Let the motion of the square loop be along x-axis and let us measure x coordinate of the centre of square loop from the centre of the square magnetic field region (taken as origin). Thus, x coordinate will be positive if the centre of square loop is to the right of the origin O (centre of magnetic field) and negative if centre is to the left.

For x=a/4
(i) magnetic flux through the loop,
(ii) induced current in the loop and
(iii) external force required to maintain constant velocity of the loop, will be
View SolutionPQRS is a square region of side 2a in the plane of paper. A uniform magnetic field B, directed perpendicular to the plane of paper and into its plane is confined within this square region. A square loop of side 'a' and made of a conducting wire of resistance R is moved at a constant velocity →v from left to right in the plane of paper as shown. Obviously, the square loop will enter the magnetic field at some time and then leave it after some time. During the motion of loop, whenever magnetic flux through it changes, emf will be induced resulting in induced current. Let the motion of the square loop be along x-axis and let us measure x coordinate of the centre of square loop from the centre of the square magnetic field region (taken as origin). Thus, x coordinate will be positive if the centre of square loop is to the right of the origin O (centre of magnetic field) and negative if centre is to the left.

External force required to maintain constant velocity of the loop for x=−95a will be
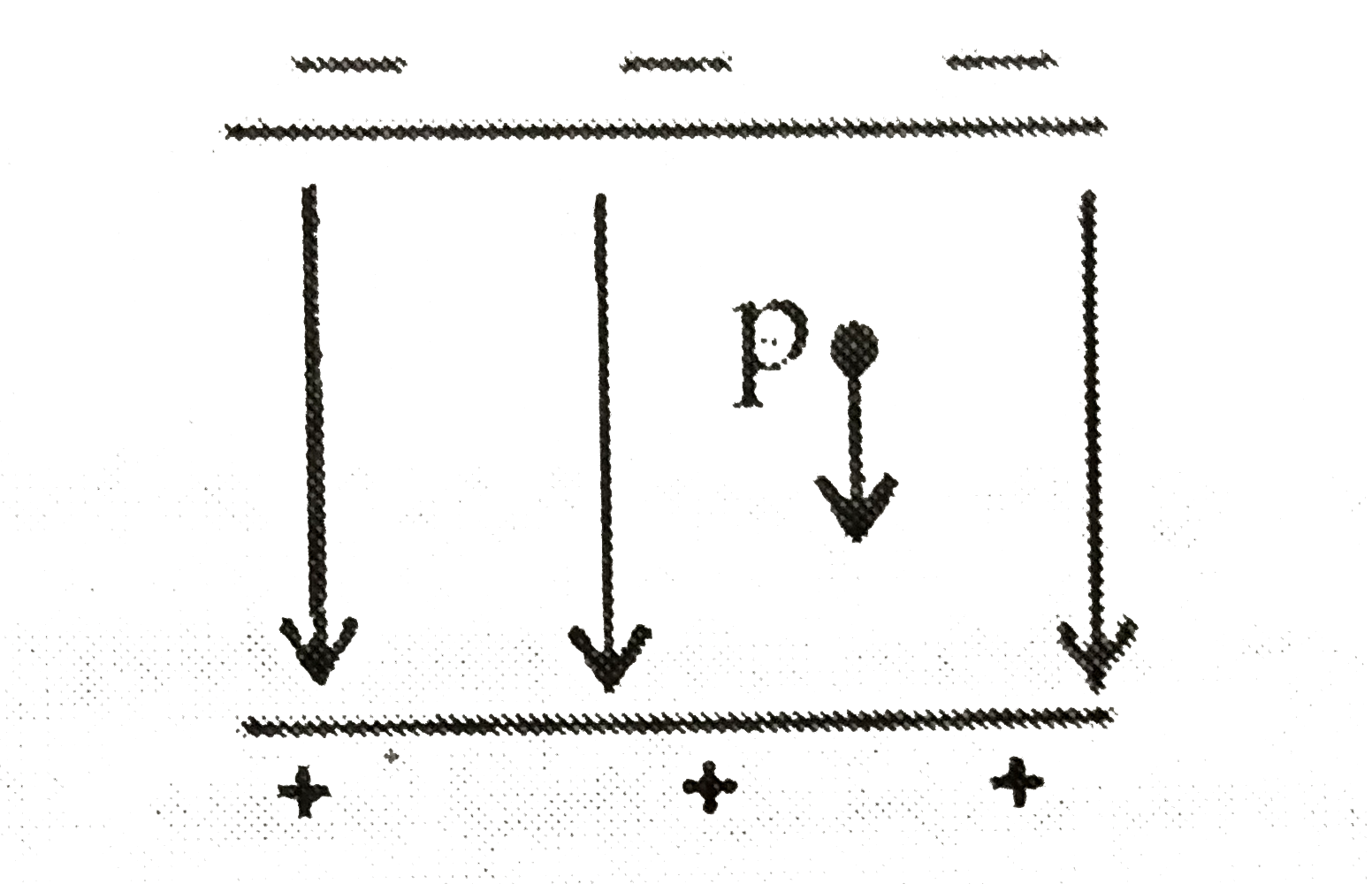
View SolutionAn electron falls through a distance of 1.5 cm in a uniform electric field of magnitude 2.0×104N/C(Fig.a)
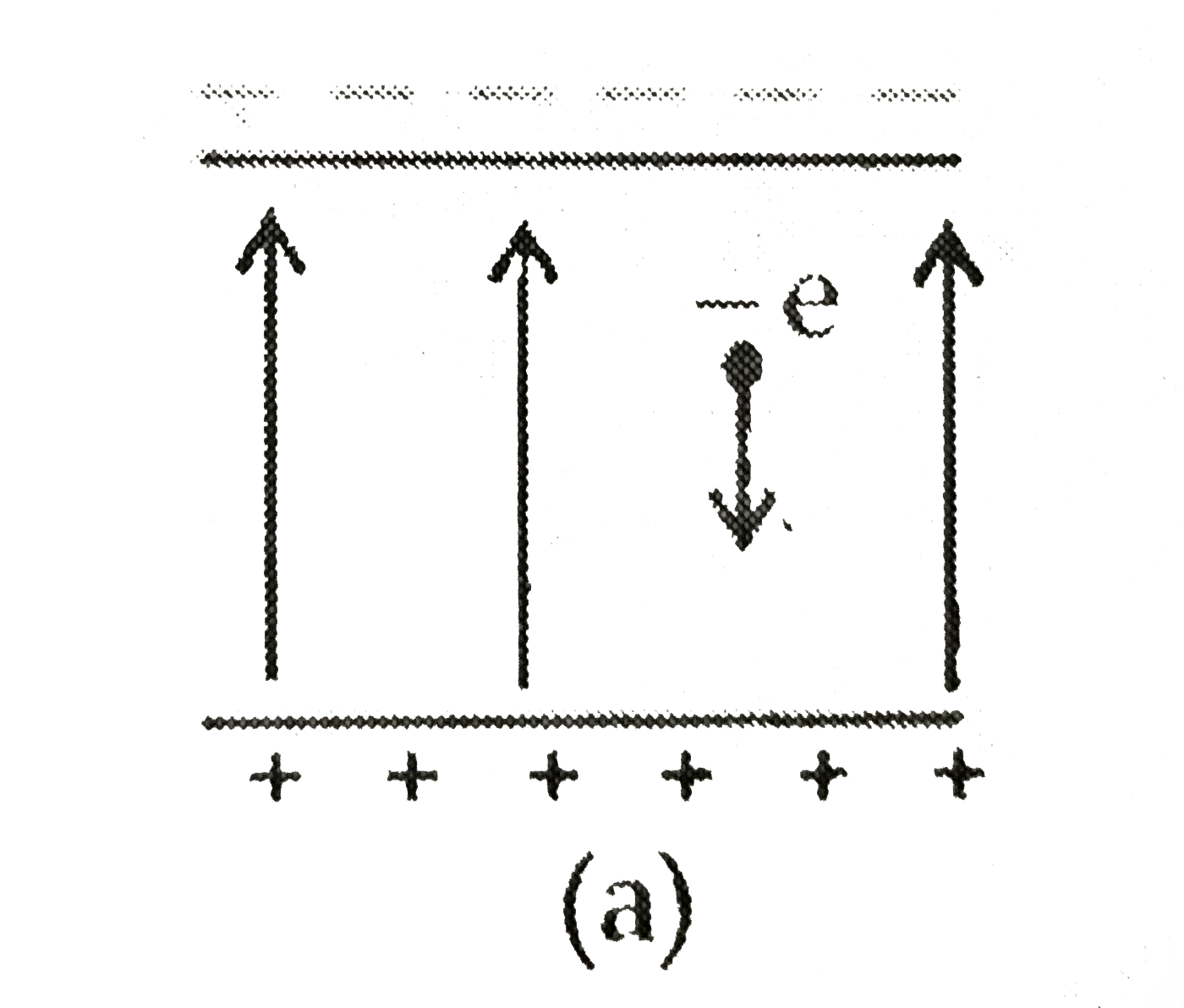
Calculate the time it takes to fall through this distance starting from rest. If the direction of the field is reversed (fig .b) keeping its magnitude unchanged , calculate the time taken by a proton to fall through this distance starting from rest.
View SolutionA cyclotron is device for accelerating ions and charged particles. It was developed by Lawerence in 1932.
The heart of the appritus consists of a split metal pillbox. Figure sHOws top and front views of the halves called dees. A rapidly oscilating potential difference is applied between the Dees. This produces an oscilating electric field in the gap between the dees, the region inside each dee being essentially free of electric field. The Dees are enclosed in an evaccutaed container, and the entire unit is placed in a uniform magnetic field B whose direction is normal to the plane Of Dees, A charged particle of mass 'm' and charge 'q' in the gap between the dees, it moves with constant speed in a semi-circle.
The period of uniform circular motion is T=2πmqB and is independant of speed. If the time-period of the oscilating elctric field is equal to this time, then the charged particle will be accelerated again and again

Answer the following questions (consider the mass of particles remains constant during motion):
A cyclotron has been adjusted to accelereted deutrons. It is now to be adjusted to accelerate other particles , for this following changes may be made:
(a) In order to keep the frequency of oscillating electric field same, the magnetic field is halved for proton
(b) If the magnetic field remain unchanged, the oscillation frequency of electric field should be halved for α particle
(c ) If the magnetic field remain unchanged, the oscillation frequency of electric field should be double for proton
(d) If the frequency of oscillating electric field is kept same, the magnetic field should be kept same for α particle
View SolutionA long solenoid of radius 2R contains another coaxial solenoid of radius R. The coils have the same number of turns per unit length and initially both carry zero current. At time, t=0, current start increasing linearly with time in both solenoids. At any moment the current flowing in the inner coil is twice as large as that in the outer one and their directions are same. A charged particle, initially at rest between the two solenoids, start moving along a circular trajectory due to increasing current in the solenoid as shown in the figure What is the radius of the circle ? (Assume magnetic field due to each solenoid remains uniform over its cross-section.)

View Solution
