In the arrangement $C_{1} = 10 μ, F C_{2} = 10 μ F$ and $C_{3} = 20 μ F$ . What is the equivalent capacitance ( in $μ F$ ) between points A and B ?

A

$7.5$

B

10

C

12

D

15

Video Solution

Text Solution

Verified by Experts

Answer (1)
$C_{A B} = \frac{C_{1} (C_{2} + C_{3})}{C_{1} + C_{2} + C_{3}} = \frac{10 (10 + 20)}{10 + 10 + 20} = \frac{300}{40} = 7.5 μ F$

Updated on:21/07/2023

|

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Similar Questions

A combination arrangement of the capacitors is shown in the figure
(i) $C_{1} = 3 μ F, C_{2} = 6 μ F$ and $C_{3} = 2 μ F$ then equivalent capacitance between 'a' and 'b' is :

View Solution
Consider two capacitors $: C_{1} = 10 μ C$ and $C_{2} = 40 μ F$ . What is the equivalent capacitance if the two are connected.
(1) in parallel ?
(2) in series ?
(3) If the two are connected in series to a 9.0 volt battery what are the charges $Q_{1}$ and $Q_{2}$ stored on the two capacitors ?
View Solution
Take $C_{1} = 4.0 μ F and C_{2} = 6.0 μ F$ in figure. Calculate the equivalent capacitance of the combination between the point s indicated.

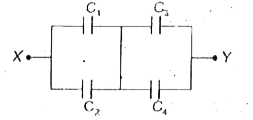
View Solution
In the circuit shown below $C_{1} = 10 μ F, C_{2} = C_{3} = 20 μ$ F, and $C_{4} = 40 μ$ F. if the charge on $C_{1}$ is $20 μ$ C then potential difference between X and Y is

View Solution
In the circuit below $C_{1} = 20 μ F , C_{2} = 40 μ$ F and $C_{3} = 50 μ$ F . If no capacitor can sustain more than 50 V, then maximum potential difference between X and y is

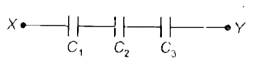
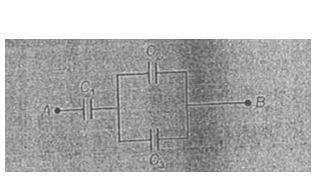
View Solution
Find the equivalent capacitance in $(μ F)$ of the infinite ladder shown in the figure between the points A and B.

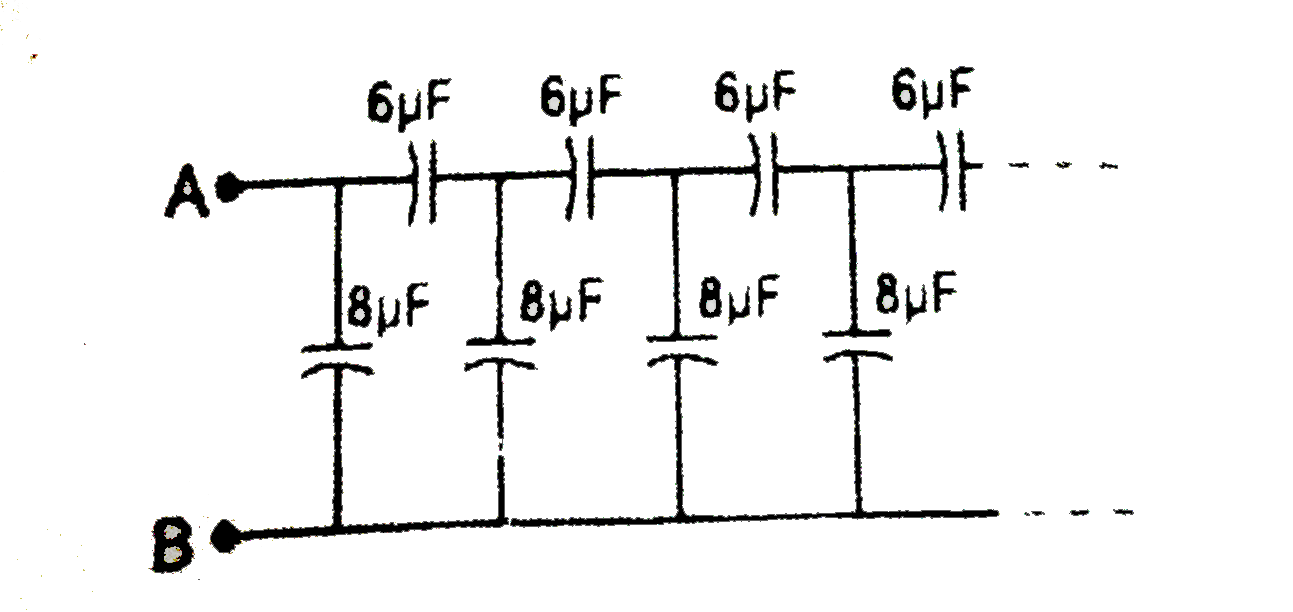
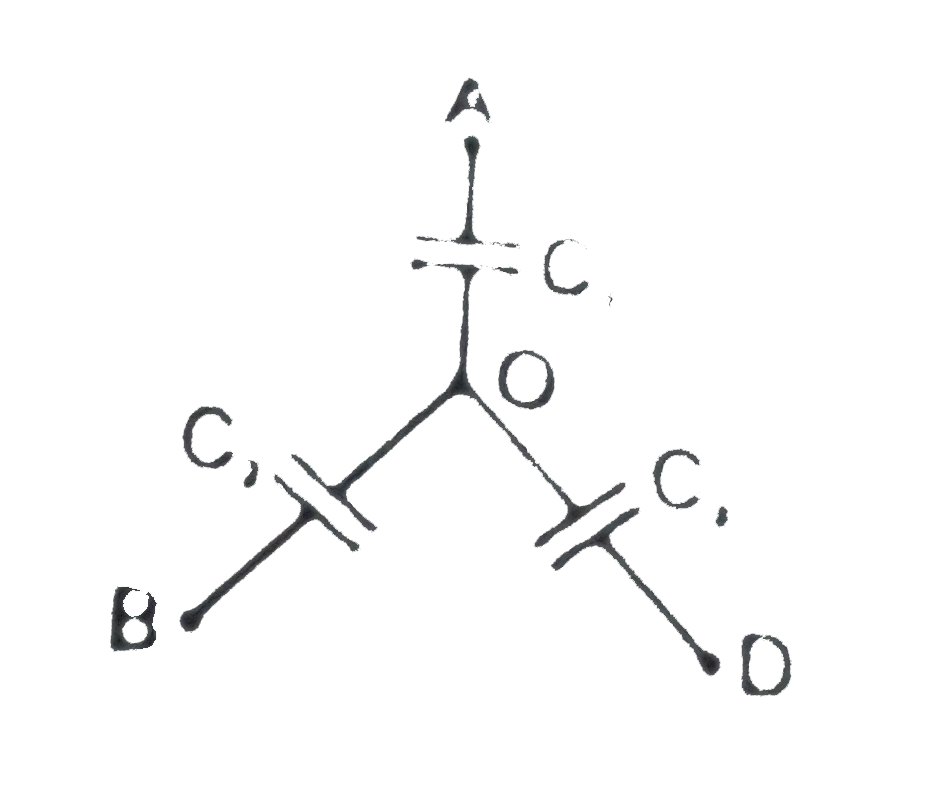
View Solution
Three uncharged capacitors of capacitance $C_{1} = 1 μ F, C_{2} = 2 μ F$ and $C_{3} = 3 μ F$ are connected as shown in the figure. The potential of point A, B and D are 10 volt, 25 volt and 20 volt respectively. Determine the potential at point O.

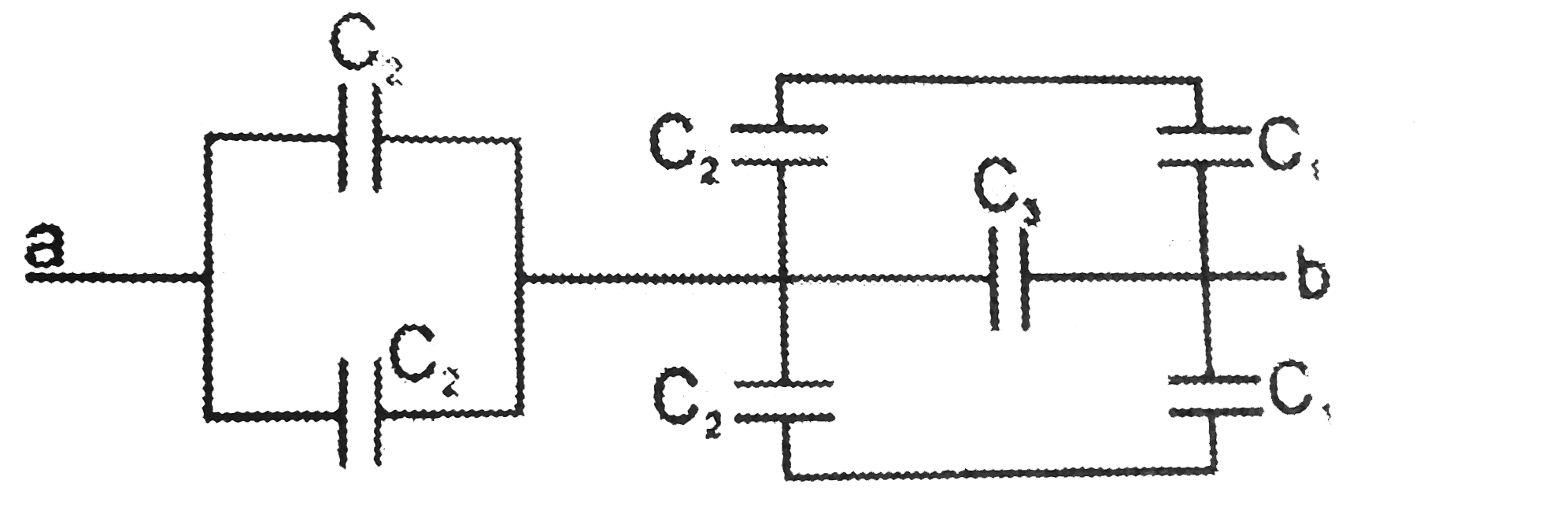
View Solution
In the arrangement of the capacitor shown in the figure, each $C_{1}$ capacitor has capacitance of $3 μ F$ and each $C_{2}$ capacitor has capacitance of $2 μ F$ then,

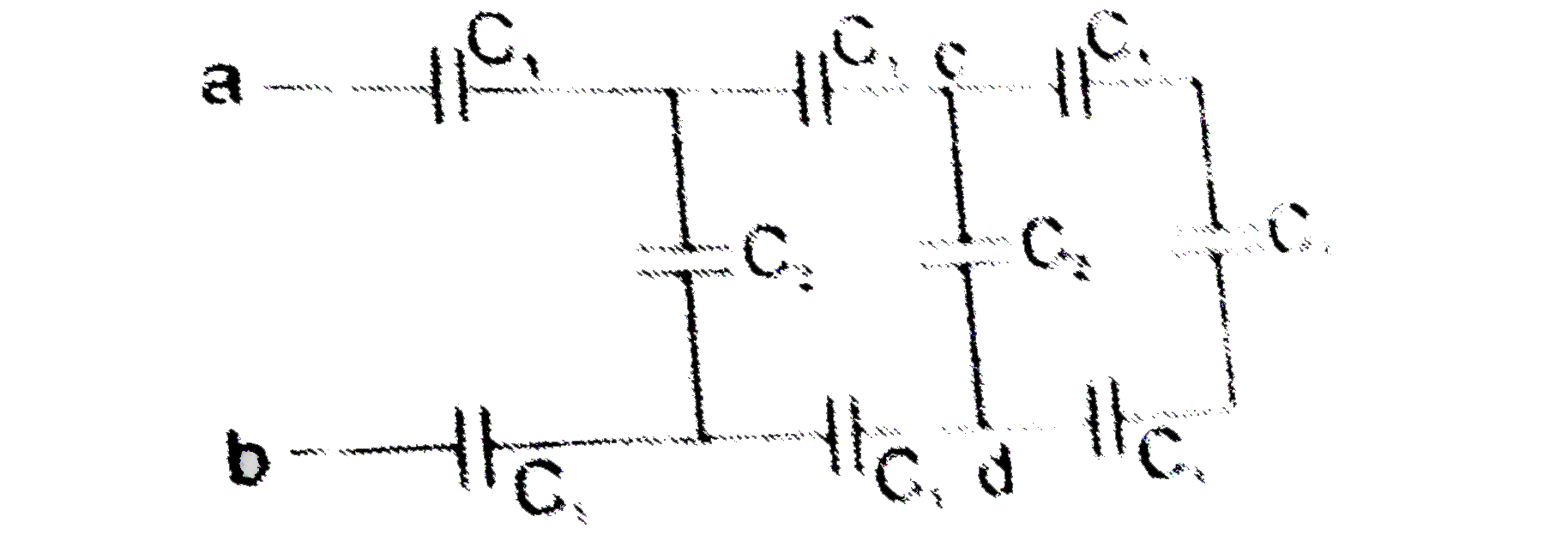
(i) Equivalent capacitance of the network between the points a and b is :
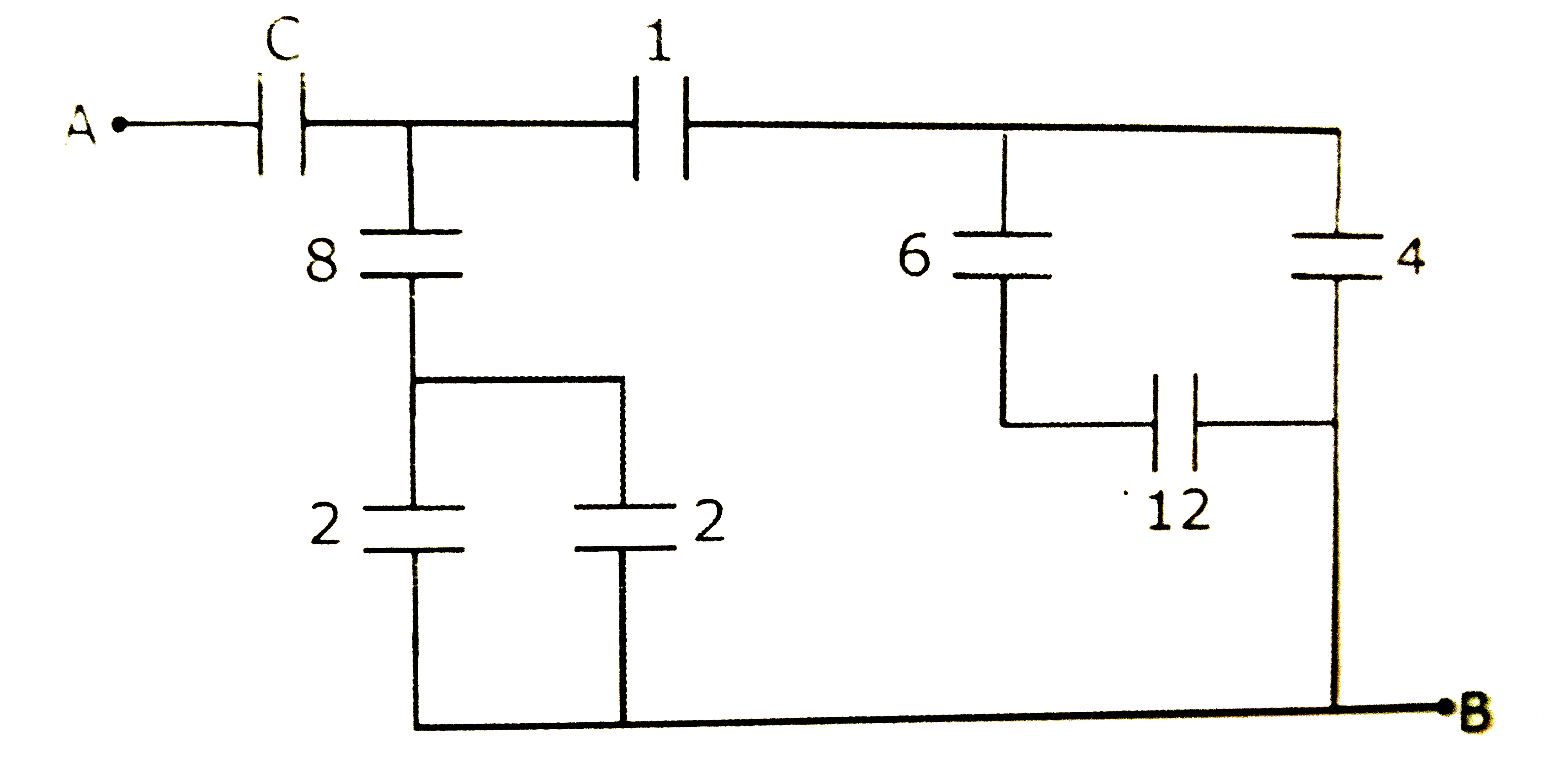
View Solution
Figure shows a network of capacitors where the numbers indicates capacitances in micro Farad. The value of capacitance C if the equivalent capacitance between point A and B is to $1 μ F$ is :

View Solution
An infinite ladder is constructed by connecting several sections of 2 $μ F, 4 μ F$ capacitor combination as shown in figure . It is terminated by a capacitor of capacitance C. What value should be chosen for C such that the equivalent capacitance of the ladder between points A and B becomes independent of the number of sections in between

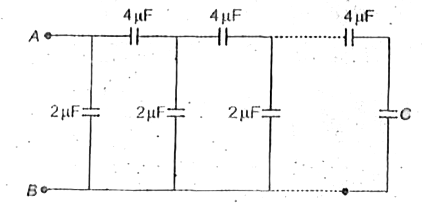
View Solution

Recommended Questions

Home
Ask Doubt
Profile