Two forces with equal magnitudes F act on a body and the magnitude of the resultant force is F /3. The angle between the two forces is
cos−1(−1718)
cos−1(−13)
cos−1(23)
cos−1(89)
The correct Answer is:A
To solve the problem, we need to find the angle between two equal forces F when the resultant force is given as F3.
1. Identify the Given Information:
- Two forces F1 and F2 with equal magnitudes F.
- The magnitude of the resultant force R=F3.
2. Use the Formula for Resultant of Two Forces:
The formula for the resultant R of two forces F1 and F2 acting at an angle θ is given by:
R=√F21+F22+2F1F2cosθ
Since F1=F2=F, we can substitute:
R=√F2+F2+2F⋅Fcosθ
This simplifies to:
R=√2F2+2F2cosθ
3. Substituting the Resultant Value:
We know that R=F3, so we can set up the equation:
F3=√2F2+2F2cosθ
4. Square Both Sides:
Squaring both sides to eliminate the square root gives:
(F3)2=2F2+2F2cosθ
This simplifies to:
F29=2F2+2F2cosθ
5. Rearranging the Equation:
Rearranging the equation, we get:
F29−2F2=2F2cosθ
To combine the terms, convert 2F2 to a fraction:
F29−18F29=2F2cosθ
This simplifies to:
−17F29=2F2cosθ
6. Dividing by F2:
Dividing both sides by F2 (assuming F≠0):
−179=2cosθ
7. Solving for cosθ:
Dividing both sides by 2 gives:
cosθ=−1718
8. Finding the Angle θ:
To find the angle θ, we take the inverse cosine:
θ=cos−1(−1718)
Final Answer:
The angle between the two forces is θ=cos−1(−1718).
- Two forces F1 and F2 with equal magnitudes F.
- The magnitude of the resultant force R=F3.
The formula for the resultant R of two forces F1 and F2 acting at an angle θ is given by:
R=√F21+F22+2F1F2cosθ
Since F1=F2=F, we can substitute:
R=√F2+F2+2F⋅Fcosθ
This simplifies to:
R=√2F2+2F2cosθ
We know that R=F3, so we can set up the equation:
F3=√2F2+2F2cosθ
Squaring both sides to eliminate the square root gives:
(F3)2=2F2+2F2cosθ
This simplifies to:
F29=2F2+2F2cosθ
Rearranging the equation, we get:
F29−2F2=2F2cosθ
To combine the terms, convert 2F2 to a fraction:
F29−18F29=2F2cosθ
This simplifies to:
−17F29=2F2cosθ
Dividing both sides by F2 (assuming F≠0):
−179=2cosθ
Dividing both sides by 2 gives:
cosθ=−1718
To find the angle θ, we take the inverse cosine:
θ=cos−1(−1718)
The angle between the two forces is θ=cos−1(−1718).
Topper's Solved these Questions
Similar Questions
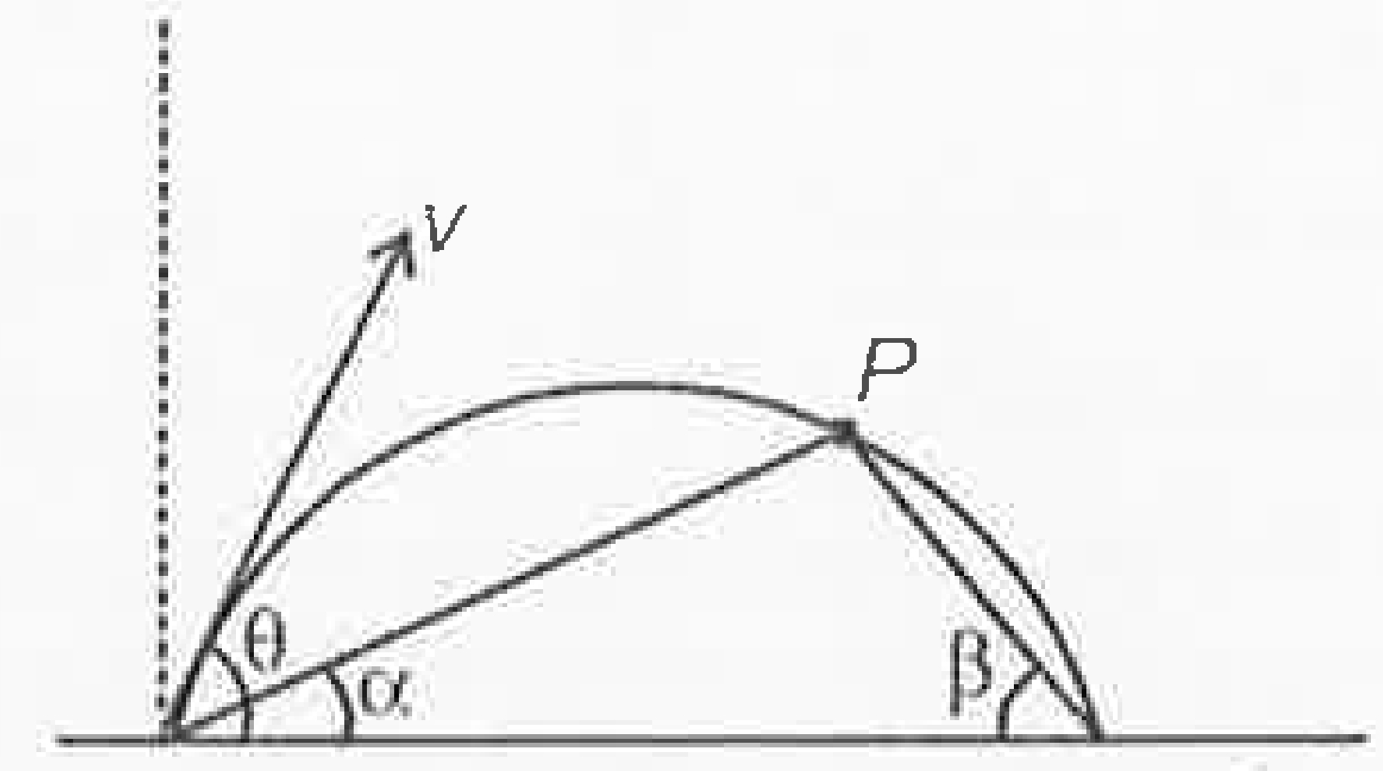
A point P on the trajectory of a projectile projected at angle with θ with horizontal subtrends angles α and β at the point of landing them
View SolutionThe r.m.s speed of the molecules of an ideal gas Is V0 If pressure of the gas is halved but temperature is kept constant then r.m.s. speed of the gas molecule will be
View SolutionTwo forces, each of magnitude F have a resultant of the same magnitude F. The angle between the two forces is
View SolutionTwo forces with equal magnitudes F act on a body and the magnitude of the resultant force is F /3. The angle between the two forces is
View SolutionTwo forces with equal magnitudes F act on body and the magnitude of the resultant forces is (F)/(3). The angle between the two forces is
View SolutionTwo forces of equal magnitude F are at a point. If theta is the angle between two forces then magnitude of the resultant forces will be
View SolutionTwo forces of equal magnitude F act at a point. If the angle between them is θ, then the magnitude of the resultant force is
View Solutionसमान परिणाम F वाले दो बल एक वस्तु पर क्रिया करते है और परिणामी बल का परिणाम F/3 है | इन दोनों बलों के बीच का कोण होगा -
View SolutionIf two forces are acting at a point such that the magnitude of each force is 2N and the magnitude of their resultant is also 2N, then the angle between the two forces is
View SolutionTwo forces of equal magnitude act simultaneously on a partical. If the resultant of the forces is equal to the magnitude of each of then the angle between the forces is
View SolutionTwo forces of the same magnitude F are at right angles to each other. The magnitude of the net force (total force) acting on the object is
View SolutionTwo forces of magnitude F having a resultant of the same magnitude of ,F the angle between the two forces is ______
View SolutionTwo forces with equal magnitudes F act on body and the magnitude of the resultant forces is F3. The angle between the two forces is
View SolutionTwo forces of equal magnitude F are at a point. If θ is the angle between two forces then magnitude of the resultant forces will be
View SolutionTwo forces, each of magnitude F have a resultant of the same magnitude F. The angle between the two forces is
View Solution
