Two equally charged identical metal spheres X and Y repel each other with a force 5⋅10−4⋅ N another identical uncharged sphere C is touched to A and then placed at the mid-point between X and Y. Net electric force on C is
5x10−4N
15x10−4N
3x10−4N
zero
The correct Answer is:A
To solve the problem, we will follow these steps:
Step 1: Understand the Problem
We have two equally charged identical metal spheres, X and Y, that repel each other with a force of F=5×10−4N. An uncharged identical sphere, C, is touched to sphere X, acquiring half of its charge, and then placed at the midpoint between X and Y.
Step 2: Determine the Charge on Spheres
Let the charge on each of the spheres X and Y be q. The force of repulsion between them can be expressed using Coulomb's law:
F=k⋅q2r2
where k=14πϵ0 is Coulomb's constant, q is the charge on each sphere, and r is the distance between the centers of the two spheres.
Step 3: Charge Distribution on Sphere C
When sphere C is touched to sphere X, it acquires half of the charge from sphere X. Therefore, the charge on sphere C becomes:
qC=q2
The charge on sphere X after touching sphere C becomes:
qX=q2
Step 4: Calculate the Forces on Sphere C
Now, sphere C is placed at the midpoint between spheres X and Y. The distance from sphere C to either sphere X or Y is r2.
Force on C due to X
The force FCX on sphere C due to sphere X is given by:
FCX=k⋅qC⋅qX(r2)2=k⋅(q2)⋅(q2)(r2)2
=k⋅q24r24=k⋅q2r2
Force on C due to Y
The force FCY on sphere C due to sphere Y is given by:
FCY=k⋅qC⋅qY(r2)2=k⋅(q2)⋅q(r2)2
=k⋅q22r24=2k⋅q2r2
Step 5: Net Force on Sphere C
The net force FC acting on sphere C is the vector sum of the forces due to spheres X and Y:
FC=FCY−FCX
Substituting the values we calculated:
FC=2k⋅q2r2−k⋅q2r2=k⋅q2r2
Step 6: Relate to Given Force
From the earlier step, we know that:
F=k⋅q2r2=5×10−4N
Conclusion
The net electric force on sphere C is:
FC=5×10−4N
We have two equally charged identical metal spheres, X and Y, that repel each other with a force of F=5×10−4N. An uncharged identical sphere, C, is touched to sphere X, acquiring half of its charge, and then placed at the midpoint between X and Y.
Let the charge on each of the spheres X and Y be q. The force of repulsion between them can be expressed using Coulomb's law:
F=k⋅q2r2
where k=14πϵ0 is Coulomb's constant, q is the charge on each sphere, and r is the distance between the centers of the two spheres.
When sphere C is touched to sphere X, it acquires half of the charge from sphere X. Therefore, the charge on sphere C becomes:
qC=q2
The charge on sphere X after touching sphere C becomes:
qX=q2
Now, sphere C is placed at the midpoint between spheres X and Y. The distance from sphere C to either sphere X or Y is r2.
The force FCX on sphere C due to sphere X is given by:
FCX=k⋅qC⋅qX(r2)2=k⋅(q2)⋅(q2)(r2)2
=k⋅q24r24=k⋅q2r2
The force FCY on sphere C due to sphere Y is given by:
FCY=k⋅qC⋅qY(r2)2=k⋅(q2)⋅q(r2)2
=k⋅q22r24=2k⋅q2r2
The net force FC acting on sphere C is the vector sum of the forces due to spheres X and Y:
FC=FCY−FCX
Substituting the values we calculated:
FC=2k⋅q2r2−k⋅q2r2=k⋅q2r2
From the earlier step, we know that:
F=k⋅q2r2=5×10−4N
The net electric force on sphere C is:
FC=5×10−4N
Topper's Solved these Questions
Similar Questions
Two similarly and equally charged identical metal spheres A and B repel each other with a force of 2×10−5N. A third identical unchared sphere C is touched with A and then placed at the midpoint between A and B. Find the net electric force on C.
View SolutionTwo equally charged, indentical metal spheres A and B repel each other with a force F. The spheres are kept fixed with a distance r between them. A third identical, but uncharged sphere C is brought in contact with A and removed. The magnitude of the net electric force on A is
View Solution
Knowledge Check
Two point charges of +3μCand+4μC repel each other with a force of 10 N. If each is given an additional charge of −6μC, the new force is
A2 NB4 NC5 ND7.5 N
Two uniform spheres A (Hollow) and B (solid) of same radius R (√125GmPr, where P is _____
View SolutionTwo point charge +2C and +6C repel each other with a force of 12N . If a charge of −4C is given to each other of these charges , the force will now be
View SolutionTwo equally charged identical small balls kept some fixed disttance apart exert a repulsive force F on each other. A similar uncharged ball, after touching one of them is placed at the mid-point of line joining the two balls. Force experienced by the third ball is
View SolutionTwo point charges +10 muC and +20 muC repel each other with a force of 100N. If a charge of-2 muC is added to each charge, then force between them will become
View SolutionAn uncharged sphere of metal is placed in between two charged plates as shown. The lines of force look like
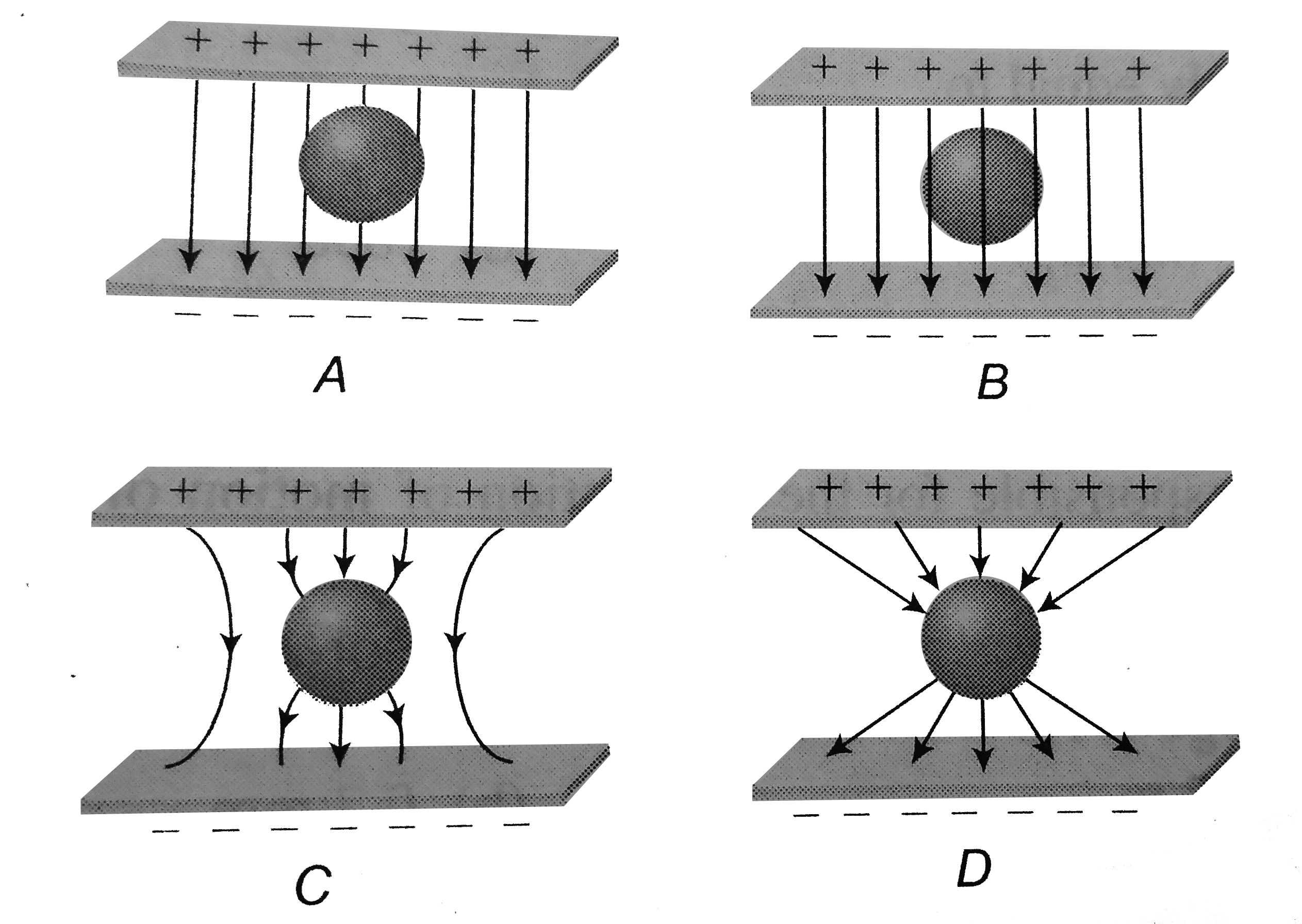
View SolutionTwo identical conducting spheres A and B, carry equal charge. They are separated by a distance much larger than their diameters, and the force between them is F. A third identical conducting spheres, C,is uncharged. Sphere C is first touched to A, then to B, and then removed. As a result, the force between A and B would be equal to :
View SolutionTwo identical conducting spheres are charged by induction and then separated by a large distance, sphere 1 has charge +Q and sphere 2 has charge -Q. A third sphere is initially uncharged. If sphere 3 is touched to sphere 1 and separated and then touched to sphere 2 and separated what is the final charge on each of the three spheres?
View Solution