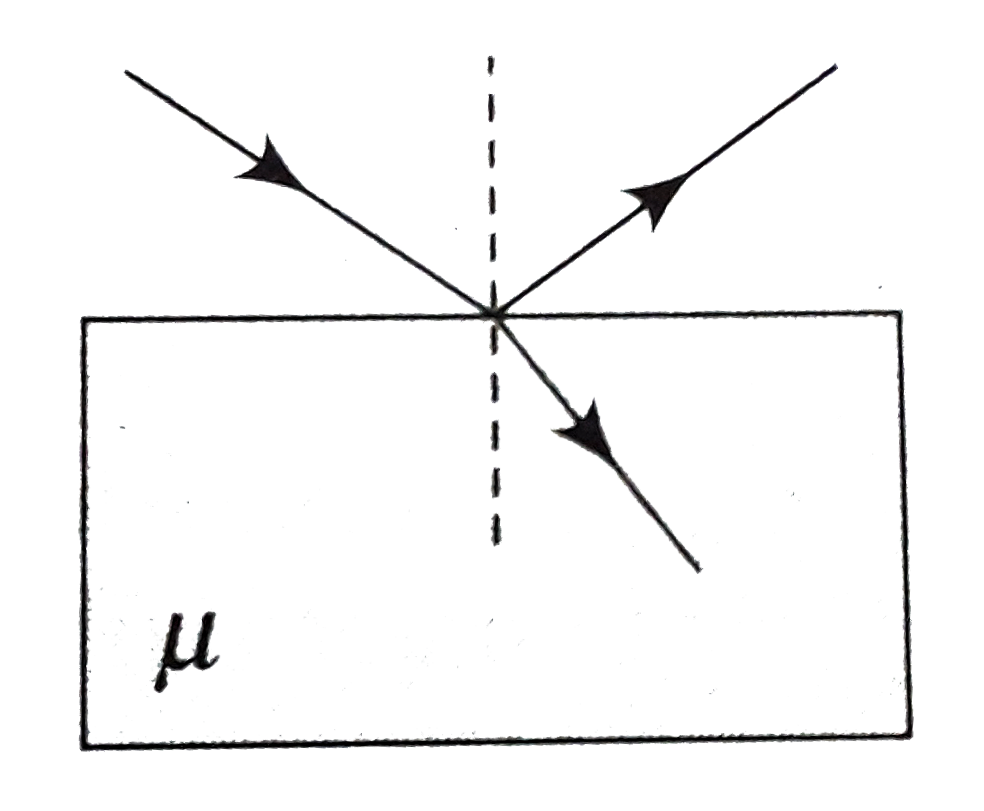
A ray of light falls on a transparent glass slab of refractive index √2. If the reflected and refracted rays are mutually perpendicular, then the angle of incidence is
tan−1(√32)
sin−1(√23)
tan−1(√2)
sin−1(√3)
The correct Answer is:c
To solve the problem, we need to find the angle of incidence when a ray of light falls on a transparent glass slab with a refractive index of √2, and the reflected and refracted rays are mutually perpendicular.
1. Understanding the Geometry:
- When the reflected and refracted rays are mutually perpendicular, it means that the angle between the reflected ray and the refracted ray is 90∘.
- Let i be the angle of incidence, r be the angle of refraction, and R be the angle of reflection. According to the law of reflection, R=i.
2. Setting Up the Relationship:
- Since the reflected and refracted rays are perpendicular, we can write:
R+r=90∘
- Substituting R=i into the equation gives us:
i+r=90∘
- Rearranging this, we find:
r=90∘−i
3. Applying Snell's Law:
- Snell's law states:
n1sin(i)=n2sin(r)
- Here, n1 (refractive index of air) is 1 and n2 (refractive index of glass) is √2. Thus, we can write:
1⋅sin(i)=√2⋅sin(r)
4. Substituting for r:
- We know from our earlier relationship that r=90∘−i. Therefore, we can substitute this into Snell's law:
sin(i)=√2⋅sin(90∘−i)
- Using the identity sin(90∘−x)=cos(x), we have:
sin(i)=√2⋅cos(i)
5. Rearranging the Equation:
- Dividing both sides by cos(i) (assuming cos(i)≠0):
tan(i)=√2
6. Finding the Angle of Incidence:
- To find i, we take the inverse tangent:
i=tan−1(√2)
Final Answer:
The angle of incidence i is tan−1(√2).
- When the reflected and refracted rays are mutually perpendicular, it means that the angle between the reflected ray and the refracted ray is 90∘.
- Let i be the angle of incidence, r be the angle of refraction, and R be the angle of reflection. According to the law of reflection, R=i.
- Since the reflected and refracted rays are perpendicular, we can write:
R+r=90∘
- Substituting R=i into the equation gives us:
i+r=90∘
- Rearranging this, we find:
r=90∘−i
- Snell's law states:
n1sin(i)=n2sin(r)
- Here, n1 (refractive index of air) is 1 and n2 (refractive index of glass) is √2. Thus, we can write:
1⋅sin(i)=√2⋅sin(r)
- We know from our earlier relationship that r=90∘−i. Therefore, we can substitute this into Snell's law:
sin(i)=√2⋅sin(90∘−i)
- Using the identity sin(90∘−x)=cos(x), we have:
sin(i)=√2⋅cos(i)
- Dividing both sides by cos(i) (assuming cos(i)≠0):
tan(i)=√2
- To find i, we take the inverse tangent:
i=tan−1(√2)
The angle of incidence i is tan−1(√2).
Topper's Solved these Questions
Similar Questions
A ray of light is incident on a transparent glass slab of refractive index √3. If the reflected and refracted rays are mutually perpendicular, what is the angle of incidence?

View SolutionA ray of light falls on a transparent glass slab of refractive index 1.62. If the reflected ray and the refracted rays are mutually perpendicular, what is the angle of refraction ?
View Solution
Knowledge Check
A ray of light in incident on a glass plate at an angle of 60∘. What is the refractive index of glass if the reflected and refracted rays are perpendicular to each other?
A12B√32C32D√3Unpolarized light is incident on a plane glass surface. The angle of incidence so that reflected and refracted rays and perpendicular to each other, then
Ataniβ=μ2Btaniβ=μCsiniβ=μDcosiβ=μ
A ray of light is incident at an angle i from denser to rare medium. The reflected and the refracted rays are mutually perpendicular. The angle of reflection and the angle of refraction are respectively r and r'. Then the critical angle will be:
View SolutionA ray of light from a denser medium strikes a rarer medium at an angle of incidence i=tan−1(1/2). If the reflected and the refracted rays are mutually perpendicular to each-other, what is the value of the critical angle in degree?
View SolutionA ray of light 10−9 second to cross a glass slab of refractive index 1.5. The thickness of the slab will be
View SolutionStatement I: A light ray is incident on a glass slab. Some portion of it is reflected and some is refracted. Refracted and reflected rays are always perpendicular to each other.
Statement II: Angle of incidence is equal to angle of reflection.
View SolutionUnpolarized light is incident on a plane glass surface having refractive index . The angle of incidence at which reflected and refracted rays would become perpendicular to each other is :
View SolutionA : When an unpolarised light is incident on a glass plate at Brewster angle, the reflected ray and refracted ray are matually perpendicular.
R : The refractive index of glass is equal to sine of the angle of polarisation.
View Solution
