A wire of length l and charge q is bent in form of a semicircle. The charge is uniformly distributed over the length. The electric field at the centre of semicircle is
qε0l2
q2(ε0l2)
q4π(ε0l2)
q2π(ε0l2)
To find the electric field at the center of a semicircular wire of length l and charge q that is uniformly distributed, we can follow these steps:
Step 1: Understand the Geometry
The wire is bent in the shape of a semicircle. The center of the semicircle is the point where we need to calculate the electric field.
Step 2: Define the Charge Distribution
Since the charge q is uniformly distributed over the length l of the semicircle, we can express the linear charge density λ as:
λ=ql
Step 3: Consider an Element of Charge
Let’s consider a small element of charge dq located at an angle θ on the semicircle. The length of this small element can be expressed as:
dq=λ⋅rdθ=ql⋅rdθ
where r is the radius of the semicircle.
Step 4: Calculate the Electric Field Contribution
The electric field dE due to the charge element dq at the center of the semicircle can be expressed as:
dE=14πϵ0⋅dqr2
Substituting dq:
dE=14πϵ0⋅ql⋅rdθr2=q4πϵ0lrdθ
Step 5: Resolve the Electric Field into Components
The electric field dE has both horizontal and vertical components. However, due to symmetry, the horizontal components from opposite sides of the semicircle will cancel out. Therefore, we only need to consider the vertical component:
dEy=dE⋅cos(θ)=q4πϵ0lrdθ⋅cos(θ)
Step 6: Integrate Over the Semicircle
Now we integrate dEy from −π2 to π2:
Ey=∫π2−π2dEy=∫π2−π2q4πϵ0lrcos(θ)dθ
The integral of cos(θ) over the limits is:
∫π2−π2cos(θ)dθ=(sin(θ))π2−π2=sin(π2)−sin(−π2)=1−(−1)=2
Thus:
Ey=q4πϵ0lr⋅2=q2πϵ0lr
Step 7: Substitute r in Terms of l
For a semicircle, the relationship between the length l and the radius r is:
l=πr⟹r=lπ
Substituting r into the expression for Ey:
Ey=q2πϵ0l⋅lπ=q2πϵ0l2π=q2ϵ0l2
Final Result
Thus, the electric field at the center of the semicircle is:
E=q2ϵ0l2
---
The wire is bent in the shape of a semicircle. The center of the semicircle is the point where we need to calculate the electric field.
Since the charge q is uniformly distributed over the length l of the semicircle, we can express the linear charge density λ as:
λ=ql
Let’s consider a small element of charge dq located at an angle θ on the semicircle. The length of this small element can be expressed as:
dq=λ⋅rdθ=ql⋅rdθ
where r is the radius of the semicircle.
The electric field dE due to the charge element dq at the center of the semicircle can be expressed as:
dE=14πϵ0⋅dqr2
Substituting dq:
dE=14πϵ0⋅ql⋅rdθr2=q4πϵ0lrdθ
The electric field dE has both horizontal and vertical components. However, due to symmetry, the horizontal components from opposite sides of the semicircle will cancel out. Therefore, we only need to consider the vertical component:
dEy=dE⋅cos(θ)=q4πϵ0lrdθ⋅cos(θ)
Now we integrate dEy from −π2 to π2:
Ey=∫π2−π2dEy=∫π2−π2q4πϵ0lrcos(θ)dθ
The integral of cos(θ) over the limits is:
∫π2−π2cos(θ)dθ=(sin(θ))π2−π2=sin(π2)−sin(−π2)=1−(−1)=2
Thus:
Ey=q4πϵ0lr⋅2=q2πϵ0lr
For a semicircle, the relationship between the length l and the radius r is:
l=πr⟹r=lπ
Substituting r into the expression for Ey:
Ey=q2πϵ0l⋅lπ=q2πϵ0l2π=q2ϵ0l2
Thus, the electric field at the center of the semicircle is:
E=q2ϵ0l2
Similar Questions
Charge q is uniformly distributed over a thin half ring of radius R. The electric field at the centre of the ring is
View SolutionA wire of length l and mass m is bent in the form of a semicircle. The gravitational field intensity at the centre of semicircle is

View SolutionA charged wire is bent in the form of a semicircular arc of radius a. If charge per unit length is λ coulomb/metre, the electric field at the centre O is
View SolutionA thin glass rod is bent into a semicircle of radius r. A charge +Q is uniformly distributed along the upper half, and a charge -Q is uniformly distributed along the lower half, as shown in fig. The electric field E at P, the center of the semicircle, is

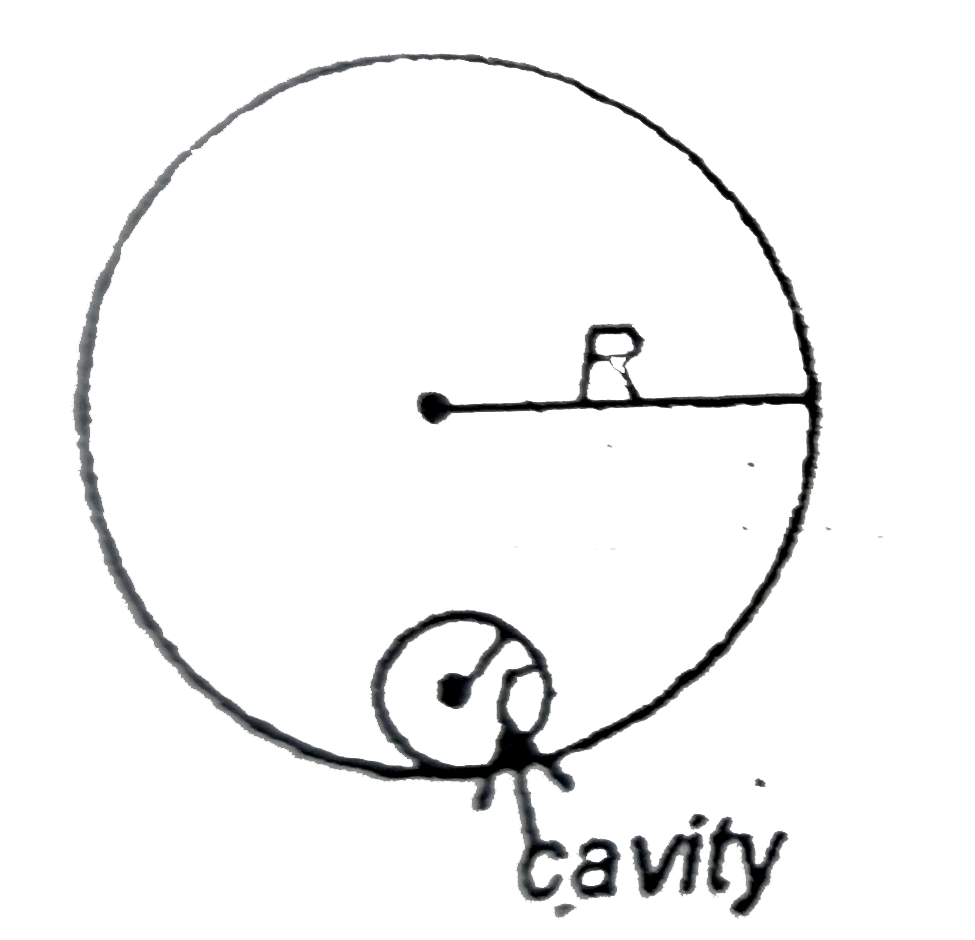
View SolutionA solid sphere of radius R has a spherical cavity of radius r as shown in the figure. If the volume charge density σ is uniformly distributed over the whole volume of the sphere, then electric field strength at the centre of the sphere will be
View SolutionA spherical shell of radius R has a uniformly distributed charge ,then electric field varies as
View SolutionAn iron rod of length L and magnetic moment M is bent in the form of a semicircle. Now its magnetic moment will be
View SolutionA rod of length L has a total charge Q distributed uniformly along its length. It is bent in the shape of a semicircle. Find the magnitude of the electric field at the centre of curvature of the semicircle.
View SolutionA wire is bent in the form of a regular hexagon and a total charge q is distributed uniformly on it. What is the electric field at the centre? You may answer this part without making any numerical calculations.
View SolutionA thin of length L is bent to form a semicircle. The mass of rod is M. What will be the gravitational potential at the centre of the circle ?
View Solution