A ball stikes a horizontal floor at an angle theta = 30° with normal to the floor . The coefficient of restitution between the ball and floor is. e=13. The percentage of its kinetic energy lost in the collision is nearly
25%
50%
33%
66%
To solve the problem, we need to find the percentage of kinetic energy lost during the collision of a ball with the floor. Here's a step-by-step solution:
Step 1: Understand the initial conditions
- The ball strikes the floor at an angle θ=30∘ with respect to the normal.
- The coefficient of restitution e=13.
Step 2: Break down the initial velocity components
Let the initial velocity of the ball be V.
- The component of velocity perpendicular to the floor (vertical component) is:
Vy=Vcos(30∘)=V⋅√32
- The component of velocity parallel to the floor (horizontal component) is:
Vx=Vsin(30∘)=V⋅12
Step 3: Determine the final velocity components after the collision
- The vertical component of the velocity after the collision is given by:
V′y=−e⋅Vy=−13⋅V⋅√32=−V√36
- The horizontal component remains unchanged:
V′x=Vx=V⋅12
Step 4: Calculate the final velocity magnitude
The final velocity V′ can be calculated using the Pythagorean theorem:
V′=√(V′x)2+(V′y)2
Substituting the values:
V′=
⎷(V⋅12)2+(−V√36)2
=√V24+V2⋅336
=√V24+V212
To combine the fractions, find a common denominator (which is 12):
=√3V212+V212=√4V212=√V23=V√3
Step 5: Calculate the initial and final kinetic energies
- Initial kinetic energy KEi:
KEi=12mV2
- Final kinetic energy KEf:
KEf=12m(V′)2=12m(V√3)2=12m⋅V23=mV26
Step 6: Calculate the percentage loss in kinetic energy
The loss in kinetic energy is:
ΔKE=KEi−KEf=12mV2−mV26
Finding a common denominator (which is 6):
=3mV26−mV26=2mV26=mV23
Now, the percentage loss in kinetic energy is:
Percentage loss=ΔKEKEi×100=mV2312mV2×100
The mV2 cancels out:
=1312×100=23×100=66.67%
Final Answer
The percentage of kinetic energy lost in the collision is approximately 67%.
- The ball strikes the floor at an angle θ=30∘ with respect to the normal.
- The coefficient of restitution e=13.
Let the initial velocity of the ball be V.
- The component of velocity perpendicular to the floor (vertical component) is:
Vy=Vcos(30∘)=V⋅√32
- The component of velocity parallel to the floor (horizontal component) is:
Vx=Vsin(30∘)=V⋅12
- The vertical component of the velocity after the collision is given by:
V′y=−e⋅Vy=−13⋅V⋅√32=−V√36
- The horizontal component remains unchanged:
V′x=Vx=V⋅12
The final velocity V′ can be calculated using the Pythagorean theorem:
V′=√(V′x)2+(V′y)2
Substituting the values:
V′=
⎷(V⋅12)2+(−V√36)2
=√V24+V2⋅336
=√V24+V212
To combine the fractions, find a common denominator (which is 12):
=√3V212+V212=√4V212=√V23=V√3
- Initial kinetic energy KEi:
KEi=12mV2
- Final kinetic energy KEf:
KEf=12m(V′)2=12m(V√3)2=12m⋅V23=mV26
The loss in kinetic energy is:
ΔKE=KEi−KEf=12mV2−mV26
Finding a common denominator (which is 6):
=3mV26−mV26=2mV26=mV23
Now, the percentage loss in kinetic energy is:
Percentage loss=ΔKEKEi×100=mV2312mV2×100
The mV2 cancels out:
=1312×100=23×100=66.67%
The percentage of kinetic energy lost in the collision is approximately 67%.
Similar Questions
A ball strickes a horizontal floor at an angle θ=45∘ with the normal to floor. The coefficient of restitution between the ball and the floor is e=1/2. The fraction of its kinetic energy lost in the collision is.
View SolutionA ball strickes a horizontal floor at an angle θ=45∘ with the normal to floor. The coefficient of restitution between the ball and the floor is e=1/2. The fraction of its kinetic energy lost in the collision is.
View Solution
Knowledge Check
A ball is dropped from a height h on to a floor . If the cofficient of restitution is e, calculate the height the ball first rebounds ?
Ae2hBeh2Ce4hDhe4
A ball is dropped from a certain height on a horizontal floor. The coefficient of restitution between the ball and the floor is 12. The displacement time graph of the ball will be.
View SolutionA ball is dropped on a smooth inclined plane and is observed to move horizontally after the impact. The coefficient of restitution between the plane and ball is e. The inclination of the plane is
View SolutionA ball is dropped from a height h on a floor. The coefficient of restitution for the collision between the ball and the floor is e. The total distance covered by the ball before it comes to the rest.
View SolutionA ball moving with speed v collides with a horizontal smooth surface at an angle θ with normal to surface as shown in figure. If coefficient of restitution of collision is e, then find v'
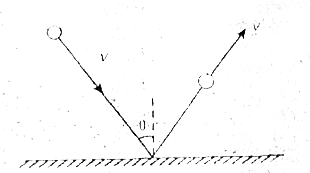
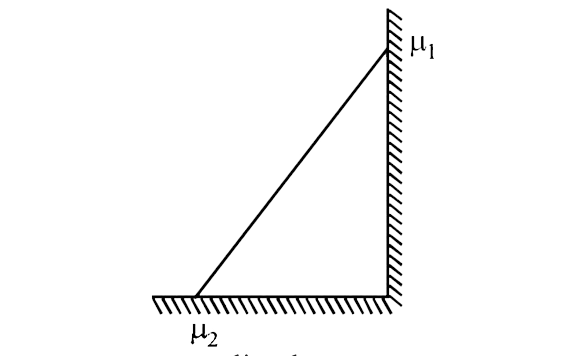
View SolutionIn the figure, a ladder of mass m is shown leaning against a wall. It is in static equilibrium making an angle θ with the horizontal floor. The coefficient of friction between the wall and the ladder is μ1 and that between the floor and the ladder is μ2. the normal reaction of the wall on the ladder is N1 and that of the floor is N2. if the ladder is about to slip. than
View SolutionA ball is dropped from height 5m. The time after which ball stops rebounding if coefficient of restitution between ball and ground e=1/2, is
View SolutionA particle of mass m strikes a horizontal smooth floor with velocity u making an angle θ with the floor and rebound with velocity v making an angle θ with the floor. The coefficient of restitution between the particle and the floor is e. Then
View Solution
