Two charges each having magnitude q and same polarity are fixed some distance apart.A point charge Q is kept on exactly middle of the line joining the two charges.Then equilibrium of Q is
Stable along the line along perpendicular bisector of the line joining the two charges if Q is of same polarity as that of q
Neutral along the line joining the two charges for any polarity of Q
Stable along the line joining two charges if charge Q is of opposite polarity to that of q
Unstable along the line joining two charges if charge Q is of opposite polarity to that of q
To solve the problem, we need to analyze the situation involving two charges of the same polarity and a point charge placed at the midpoint between them. Let's break down the solution step by step.
1. Understanding the Setup:
- We have two fixed charges, each with a magnitude of q and the same polarity (let's assume both are positive, +q).
- A point charge Q is placed at the midpoint between these two charges.
2. Analyzing Forces on Charge Q:
- Since both charges are of the same polarity (positive), they will repel the point charge Q.
- The forces acting on Q due to the two charges will be repulsive.
3. Calculating Forces:
- Let’s denote the distance between the two charges as d. Therefore, the distance from each charge to the point charge Q is d2.
- The force exerted on Q by each charge can be calculated using Coulomb's law:
\(
F = k \frac{|qQ|}{(d/2)^2} = k \frac{4|qQ|}{d^2}
\)
- Since both forces are equal in magnitude but act in opposite directions, we denote them as \( F_1 \) and \( F_2 \).
4. Direction of Forces:
- Both forces \( F_1 \) and \( F_2 \) will act away from the charges towards the point charge Q.
- If Q is displaced slightly towards one of the charges, the force from that charge will increase (since it is closer), while the force from the other charge will decrease (since it is farther away).
5. Equilibrium Analysis:
- If Q is displaced slightly towards one of the charges, the net force will push it further away from the midpoint. This indicates that the equilibrium at the midpoint is unstable.
- Hence, the equilibrium of Q is unstable along the line joining the two charges.
6. Conclusion:
- Therefore, the correct answer is that the equilibrium of Q is unstable along the line joining the two charges if the charge q is of the same polarity as Q.
Final Answer:
The equilibrium of Q is unstable along the line joining the two charges if charge q is of the same polarity.
- We have two fixed charges, each with a magnitude of q and the same polarity (let's assume both are positive, +q).
- A point charge Q is placed at the midpoint between these two charges.
- Since both charges are of the same polarity (positive), they will repel the point charge Q.
- The forces acting on Q due to the two charges will be repulsive.
- Let’s denote the distance between the two charges as d. Therefore, the distance from each charge to the point charge Q is d2.
- The force exerted on Q by each charge can be calculated using Coulomb's law:
\(
F = k \frac{|qQ|}{(d/2)^2} = k \frac{4|qQ|}{d^2}
\)
- Since both forces are equal in magnitude but act in opposite directions, we denote them as \( F_1 \) and \( F_2 \).
- Both forces \( F_1 \) and \( F_2 \) will act away from the charges towards the point charge Q.
- If Q is displaced slightly towards one of the charges, the force from that charge will increase (since it is closer), while the force from the other charge will decrease (since it is farther away).
- If Q is displaced slightly towards one of the charges, the net force will push it further away from the midpoint. This indicates that the equilibrium at the midpoint is unstable.
- Hence, the equilibrium of Q is unstable along the line joining the two charges.
- Therefore, the correct answer is that the equilibrium of Q is unstable along the line joining the two charges if the charge q is of the same polarity as Q.
The equilibrium of Q is unstable along the line joining the two charges if charge q is of the same polarity.
Similar Questions
Draw E−r and V−r graphs due to two point charges +q and −2q kept at some along the line joining these two charges.
View SolutionIf Two charges +qand+4q are separated by a distance d and a point charge Q is placed on the line joining the above two charges and in between them such that all charges are in equilibrium. Then the charge Q and it's position are
View SolutionTwo charge +aq and -q are kept apart. Then , at any point on the right bisector of line joining the two charge
View SolutionTwo point charges +q and −q are placed a distance x apart. A third charge is so placed that at the three charges are in equilibrium. Then
View SolutionTwo positive point charges of magnitude Q each are separated by a distance "2a". A test charge 'g0' is located in a plane which is normal to the line joining these charges and midway between them. The locus of the points in this plane for which the force on the test charge has a maximum value is
View SolutionTwo point charges +Q and -Q are separated by a certain distance. The resultant electric field is parallel to the line joining the charges at the points
View SolutionTwo point charges qA=3μCandqB=−3μC are located 20cm apart in vacuum. What is the electric field at the mid point of the line joining the two charges.
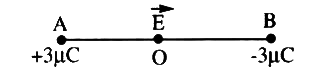
View SolutionAnswer the following:
(a) Derive and expression for the electric field at any point on the equatorial line of an electric dipole.
(b) Two identical point charges, q each kept 2m apart in air. A third point charge Q of unknown magnitude and sign is placed on the line joining the charges such that the system remains in equilibrium. Find the position and nature of Q.
View SolutionTwo charges of equal magnitude q are placed in air at a distance 2a apart and third charge −2q is placed at mid-point . The potential energy of the system is (ε0 = permittivity of free space)
View SolutionTwo idential point charges Q are kept at a distance r from each other. A third point charge is placed on the line joing the above two charges such that all the three charges are in equilibrium. The third charge
(a) should be of magnitude q=………..
(b) should be of sign .......
(c ) should be placed ........ .
View Solution
