Magnetic moment of a square loop is M.What will be the magnetic moment of the loop if its shape is changed to circular
4Mπ
πM4
πM2
πM
To solve the problem of finding the magnetic moment of a circular loop when the magnetic moment of a square loop is given as M, we can follow these steps:
Step 1: Define the side of the square loop
Let the side of the square loop be a.
Step 2: Calculate the area of the square loop
The area A of the square loop can be calculated using the formula:
A=a2
Step 3: Write the formula for magnetic moment of the square loop
The magnetic moment M of the square loop is given by:
M=I⋅A=I⋅a2
where I is the current flowing through the loop.
Step 4: Relate the square loop to the circular loop
When the shape of the loop changes from square to circular, the perimeter of the square loop will equal the circumference of the circular loop. The perimeter of the square is:
P=4a
The circumference of the circle is given by:
C=2πr
Setting these equal gives:
4a=2πr
Step 5: Solve for the radius r of the circular loop
From the equation 4a=2πr, we can solve for r:
r=4a2π=2aπ
Step 6: Calculate the area of the circular loop
The area A′ of the circular loop is given by:
A′=πr2
Substituting the value of r:
A′=π(2aπ)2=π⋅4a2π2=4a2π
Step 7: Write the formula for magnetic moment of the circular loop
The magnetic moment M′ of the circular loop is given by:
M′=I⋅A′=I⋅4a2π
Step 8: Substitute a2 from the magnetic moment of the square loop
From Step 3, we know M=I⋅a2, so we can express a2 as:
a2=MI
Substituting this into the equation for M′:
M′=I⋅4π⋅MI=4Mπ
Conclusion
Thus, the magnetic moment of the circular loop is:
M′=4Mπ
Let the side of the square loop be a.
The area A of the square loop can be calculated using the formula:
A=a2
The magnetic moment M of the square loop is given by:
M=I⋅A=I⋅a2
where I is the current flowing through the loop.
When the shape of the loop changes from square to circular, the perimeter of the square loop will equal the circumference of the circular loop. The perimeter of the square is:
P=4a
The circumference of the circle is given by:
C=2πr
Setting these equal gives:
4a=2πr
From the equation 4a=2πr, we can solve for r:
r=4a2π=2aπ
The area A′ of the circular loop is given by:
A′=πr2
Substituting the value of r:
A′=π(2aπ)2=π⋅4a2π2=4a2π
The magnetic moment M′ of the circular loop is given by:
M′=I⋅A′=I⋅4a2π
From Step 3, we know M=I⋅a2, so we can express a2 as:
a2=MI
Substituting this into the equation for M′:
M′=I⋅4π⋅MI=4Mπ
Thus, the magnetic moment of the circular loop is:
M′=4Mπ
Similar Questions
Magnetic dipole moment of rectangular loop is
View SolutionThe magnetic dipole moment of current loop is independent of
View SolutionCompute the magnetic dipole moment of the loop shown in Fig. 1.69
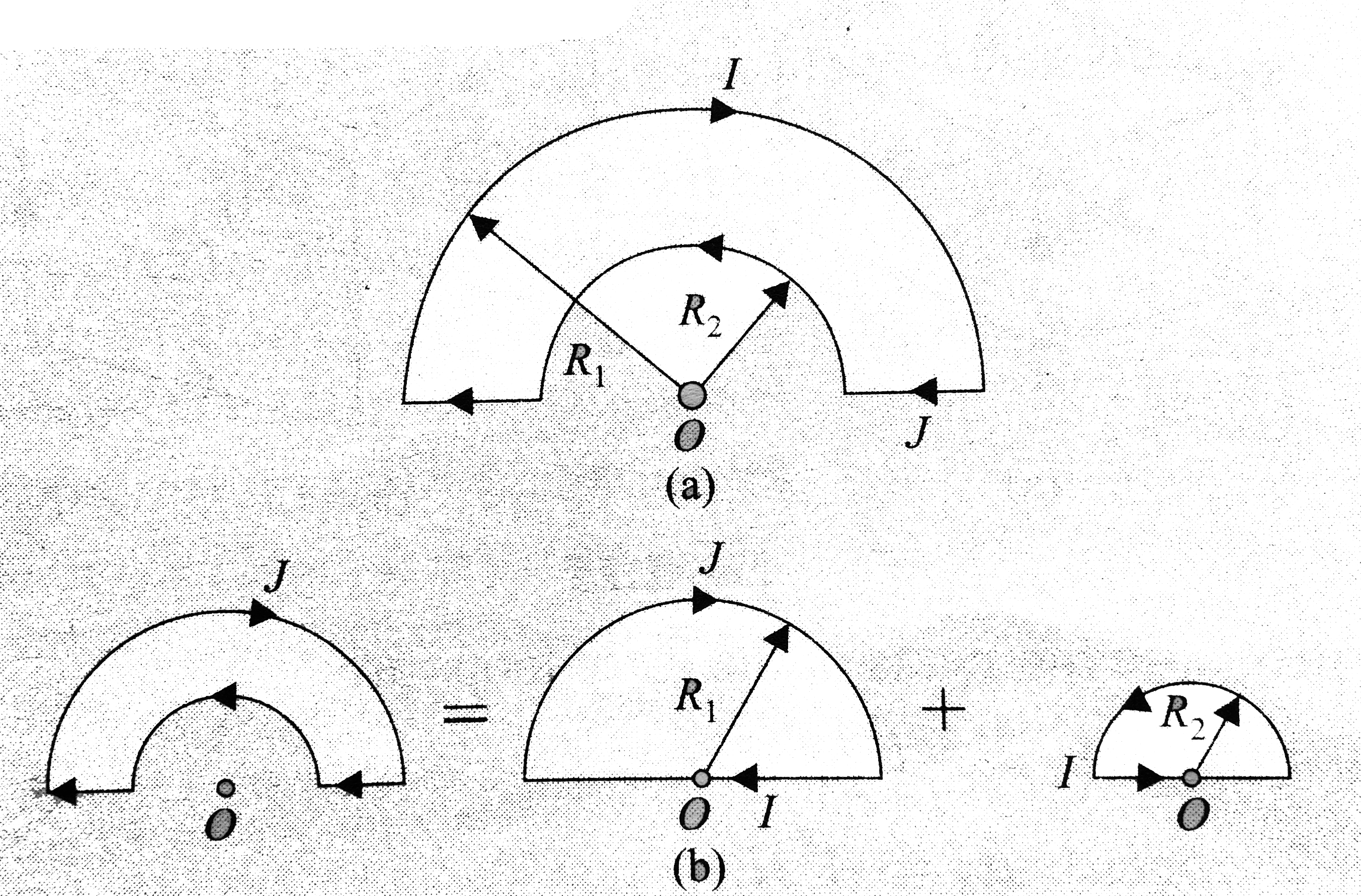 View Solution
View SolutionA steel wire has a magmetic moment M. What will be its magnetic moment if it is bent into a semi-circular arc ?
View SolutionA square loop is carrying a steady current I and the magnitude of its magnetic dipole moment is m. if this square loop is changed to a circular loop and it carries the current, the magnitude of the magnetic dipole moment of circular loop will be :
View SolutionA square loop is carrying a steady current I and the magnitude of its magnetic dipole moment is m. if this square loop is changed to a circular loop and it carries the current, the magnitude of the magnetic dipole moment of circular loop will be :
View SolutionThe dipole moment of a circular loop carrying a current I, is m and the magnetic field at the centre of the loop is B1 . When the dipole moment is doubled by keeping the current constant, the magnetic field at the centre of the loop is B2 . The ratio B1B2 is:
View SolutionA current loop, carrying a current of 5.0 A, is in the shape of a right triangle with sides 30,40, and 50 cm. The loop is in a uniform magnetic field of magnitude 80 mT whose direction is parallel to the current in the 50 cm side of the loop. Find the magnitude of (a) the magnetic dipole moment of the loop and (b) the torque on the loop.
View SolutionAn infinitesimal bar magnet of dipole moment M is pointing and moving with speed v in the x-direction. A closed circular conducting loop of radius a and negligible self-inductance lies in the y−z plane with its centre at x=0 and its axis coinciding with x-axis. find the force opposing the motion of the magnet, if the resistance of the loop is R . Assume that the distance x of the magnet from the centre of the loop is much greater than a .
View SolutionOn meter length of wires carriers a constant current. The wire is bent to from a circular loop. The magnetic field at the centre of this loop is B. The same is now bent to form a circular loop of smaller radius to have four turns in the loop. The magnetic field at the centre of this loop B. The same is now bent to form a circular loop of smaller radius of have four turns in the loop. The magnetic field at the centre of this new loop is
View Solution

