A thin rod of length L is hanging from one end and free to oscillate like s compound pendulum about a horizontal axis, Its time period for small oscillations is
2π√Lg
2π√2Lg
2π√2g3L
2π√2L3g
To find the time period of a thin rod of length L that is hanging from one end and oscillating like a compound pendulum, we can follow these steps:
Step 1: Understand the formula for the time period of a physical pendulum
The time period T of a physical pendulum is given by the formula:
T=2π√Img⋅Lcm
where:
- I is the moment of inertia of the rod about the pivot point,
- m is the mass of the rod,
- g is the acceleration due to gravity,
- Lcm is the distance from the pivot point to the center of mass of the rod.
Step 2: Calculate the moment of inertia I
For a thin rod of length L rotating about one end, the moment of inertia I is given by:
I=13mL2
Step 3: Determine the center of mass Lcm
The center of mass of a uniform thin rod is located at its midpoint, which is at a distance of L2 from the pivot point (the end from which it is hanging). Thus:
Lcm=L2
Step 4: Substitute I and Lcm into the time period formula
Now, substituting I and Lcm into the time period formula:
T=2π
⎷13mL2mg⋅L2
Step 5: Simplify the expression
Now, simplify the expression:
T=2π
⎷13L2g⋅L2
=2π√2L3g
Final Result
Thus, the time period for small oscillations of the thin rod is:
T=2π√2L3g
The time period T of a physical pendulum is given by the formula:
T=2π√Img⋅Lcm
where:
- I is the moment of inertia of the rod about the pivot point,
- m is the mass of the rod,
- g is the acceleration due to gravity,
- Lcm is the distance from the pivot point to the center of mass of the rod.
For a thin rod of length L rotating about one end, the moment of inertia I is given by:
I=13mL2
The center of mass of a uniform thin rod is located at its midpoint, which is at a distance of L2 from the pivot point (the end from which it is hanging). Thus:
Lcm=L2
Now, substituting I and Lcm into the time period formula:
T=2π
⎷13mL2mg⋅L2
Now, simplify the expression:
T=2π
⎷13L2g⋅L2
=2π√2L3g
Thus, the time period for small oscillations of the thin rod is:
T=2π√2L3g
Similar Questions
A thin rod of mass m and length l is suspended from one of its ends. It is set into oscillation about a horizontal axis. Its angular speed is ω while passing through its mean position. How high will its centre of mass rise from its lowest position ?
View SolutionA uniform rod of mass m and length l is suspended about its end. Time period of small angular oscillations is

View SolutionA uniform rod of length 1.00 m is suspended through an end and is set into oscillation with small amplitude under gravity. Find the time period of oscillation.
View SolutionA uniform rod of length 2.0 m is suspended through an end and is set into oscillation with small amplitude under gravity. The time period of oscillation is approximately
View SolutionA uniform square plate at side a is hinged at one at its comes it is suspended such than it can rotate about horizontal axis. The time period of small oscillation about its equilibrium position.
View SolutionA thin uniform rod of length l is pivoted at its upper end. It is free to swing in a vertical plane. Its time period for oscillation of small amplitude is
View SolutionA uniform rod ofmass M and length L is hanging from its one end free to rotate in a veritcal plane.A small ball of equal mass is attached of the lowe end as shown. Time period of small oscillations of the rod is

View SolutionA long uniform rod of length L and mass M is free to rotate in a horizontal plane about a vertical axis through its one end 'O'. A spring of force constant k is connected between one end of the rod and PQ. When the rod is in equilibrium it is parallel to PQ.
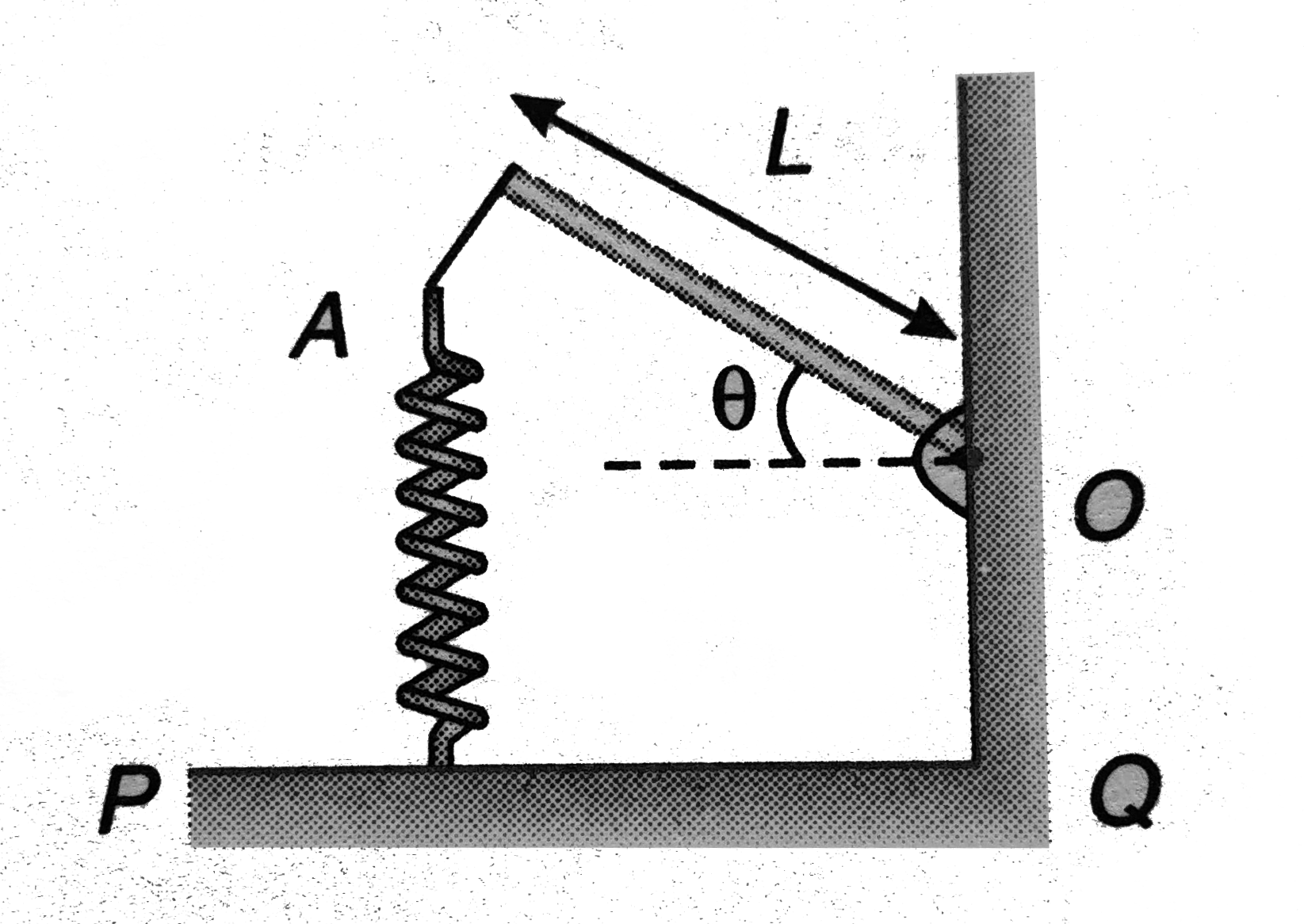
(a)What is the period of small oscillation that result when the rod is rotated slightly and released ?
(b) What will be the maximum speed of the displacement end of the rod, if the amplitude of motion is θ0?
View SolutionA solid cylinder of mass m length L and radius R is suspended by means of two ropes of length l each as shown. Find the time period of small angular oscillations of the cylinder about its axis AA'
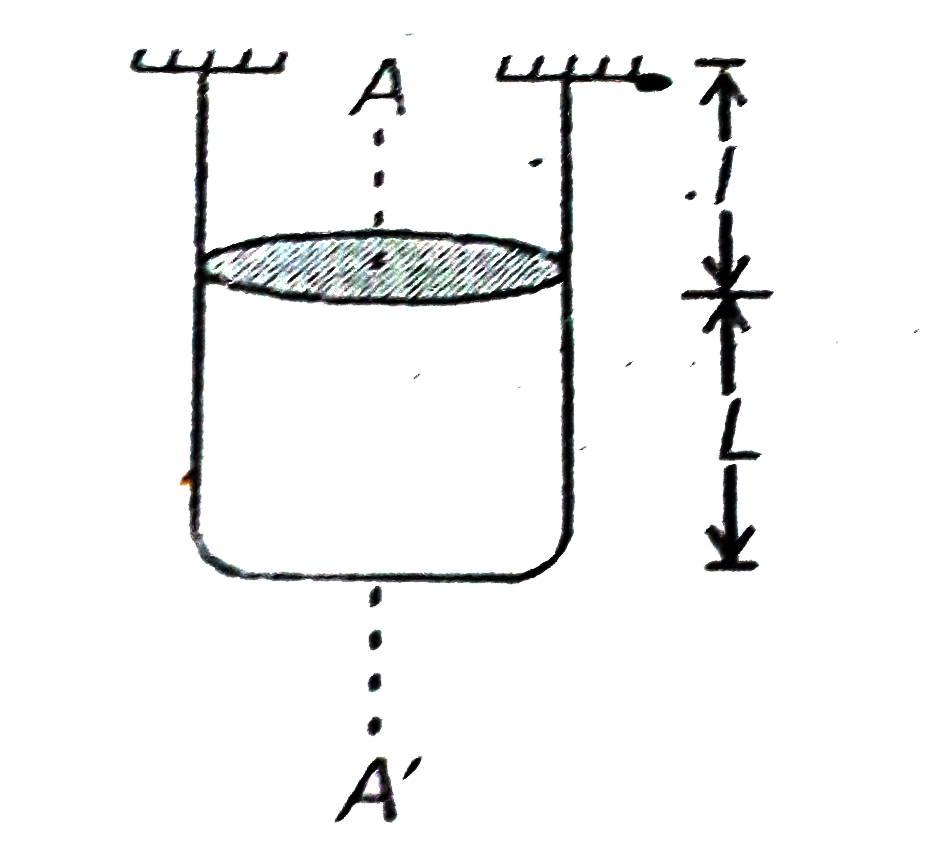
View SolutionA uniform rod of mass m and length L is hinged at one of its end with the ceiling and another end of the rod is attached with a thread which is attached with the horizontal ceiling at point P. If one end of the rod is slightly displaced horizontally and perpendicular to the rod and released. If the time period of small oscillation is 2π√2lsinθxg . Find x.
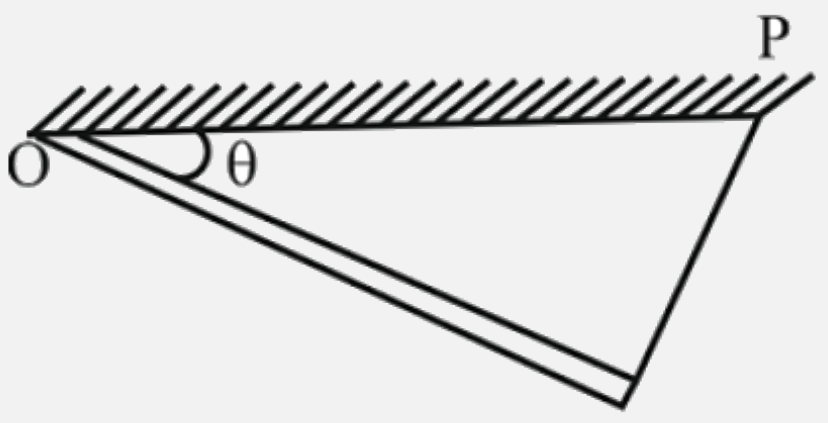
View Solution