A uniform rod of length 2.0 m is suspended through an end and is set into oscillation with small amplitude under gravity. The time period of oscillation is approximately
1.60 s
1.80 s
2.00 s
2.40 s
The correct Answer is:D
To find the time period of oscillation of a uniform rod of length 2.0 m suspended from one end, we can use the formula for the time period of a physical pendulum:
Step 1: Identify the formula
The time period T of a physical pendulum is given by the formula:
T=2π√Imgd
where:
- I is the moment of inertia of the rod about the pivot point,
- m is the mass of the rod,
- g is the acceleration due to gravity,
- d is the distance from the pivot to the center of mass of the rod.
Step 2: Calculate the moment of inertia
For a uniform rod of length L (2.0 m) suspended from one end, the moment of inertia I is given by:
I=13mL2
Substituting L=2.0m:
I=13m(2.0)2=43m
Step 3: Determine the distance to the center of mass
The center of mass of the rod is located at a distance d from the pivot, which is half the length of the rod:
d=L2=2.02=1.0m
Step 4: Substitute values into the formula
Now, substituting I and d into the time period formula:
T=2π
⎷43mmg⋅1.0=2π√43g
Here, g is approximately 9.8m/s2.
Step 5: Simplify the expression
Now substituting g:
T=2π√43⋅9.8=2π√429.4=2π√214.7
Step 6: Calculate the numerical value
Calculating the square root:
√214.7≈√0.1361≈0.369
Now substituting back:
T≈2π⋅0.369≈2.32seconds
Step 7: Final approximation
Rounding this to two decimal places, we find:
T≈2.40seconds
Thus, the time period of oscillation is approximately 2.40 seconds.
The time period T of a physical pendulum is given by the formula:
T=2π√Imgd
where:
- I is the moment of inertia of the rod about the pivot point,
- m is the mass of the rod,
- g is the acceleration due to gravity,
- d is the distance from the pivot to the center of mass of the rod.
For a uniform rod of length L (2.0 m) suspended from one end, the moment of inertia I is given by:
I=13mL2
Substituting L=2.0m:
I=13m(2.0)2=43m
The center of mass of the rod is located at a distance d from the pivot, which is half the length of the rod:
d=L2=2.02=1.0m
Now, substituting I and d into the time period formula:
T=2π
⎷43mmg⋅1.0=2π√43g
Here, g is approximately 9.8m/s2.
Now substituting g:
T=2π√43⋅9.8=2π√429.4=2π√214.7
Calculating the square root:
√214.7≈√0.1361≈0.369
Now substituting back:
T≈2π⋅0.369≈2.32seconds
Rounding this to two decimal places, we find:
T≈2.40seconds
Similar Questions
A uniform rod of length 1.00 m is suspended through an end and is set into oscillation with small amplitude under gravity. Find the time period of oscillation.
View SolutionThe time period of small oscillations of mass m :-
_S01_040_Q01.png) View Solution
View SolutionA uniform rod of length 2.0 m is suspended through its endpoint about which it performs small angular oscillations in the vertical plane, its time period is nearly
View SolutionA uniform rod of length l is suspended from a point P. and the rod is made to undergo small oxcillations. Match the following
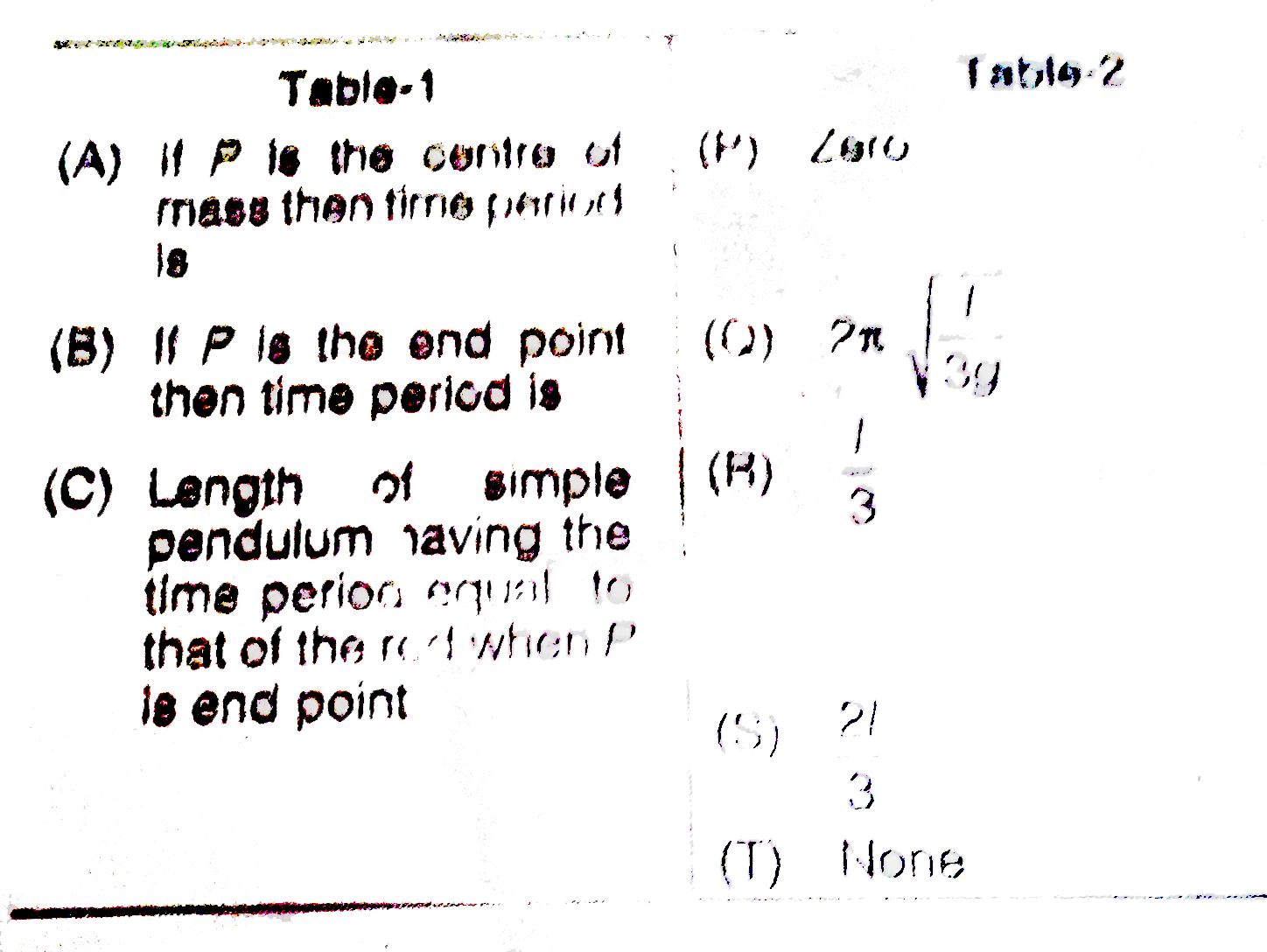 View Solution
View SolutionA uniform rod of mass m and length l is suspended about its end. Time period of small angular oscillations is
 View Solution
View SolutionA thin rod of length L is hanging from one end and free to oscillate like s compound pendulum about a horizontal axis, Its time period for small oscillations is
View SolutionAn infinite nonconducting sheet has surface charge density s. There is a small hole in the sheet as shown in the figure. A uniform rod of length l having linear charge density λ is hinged in the hole as shown. If the mass of the rod is m, then the time period of oscillation for small angular displacement is
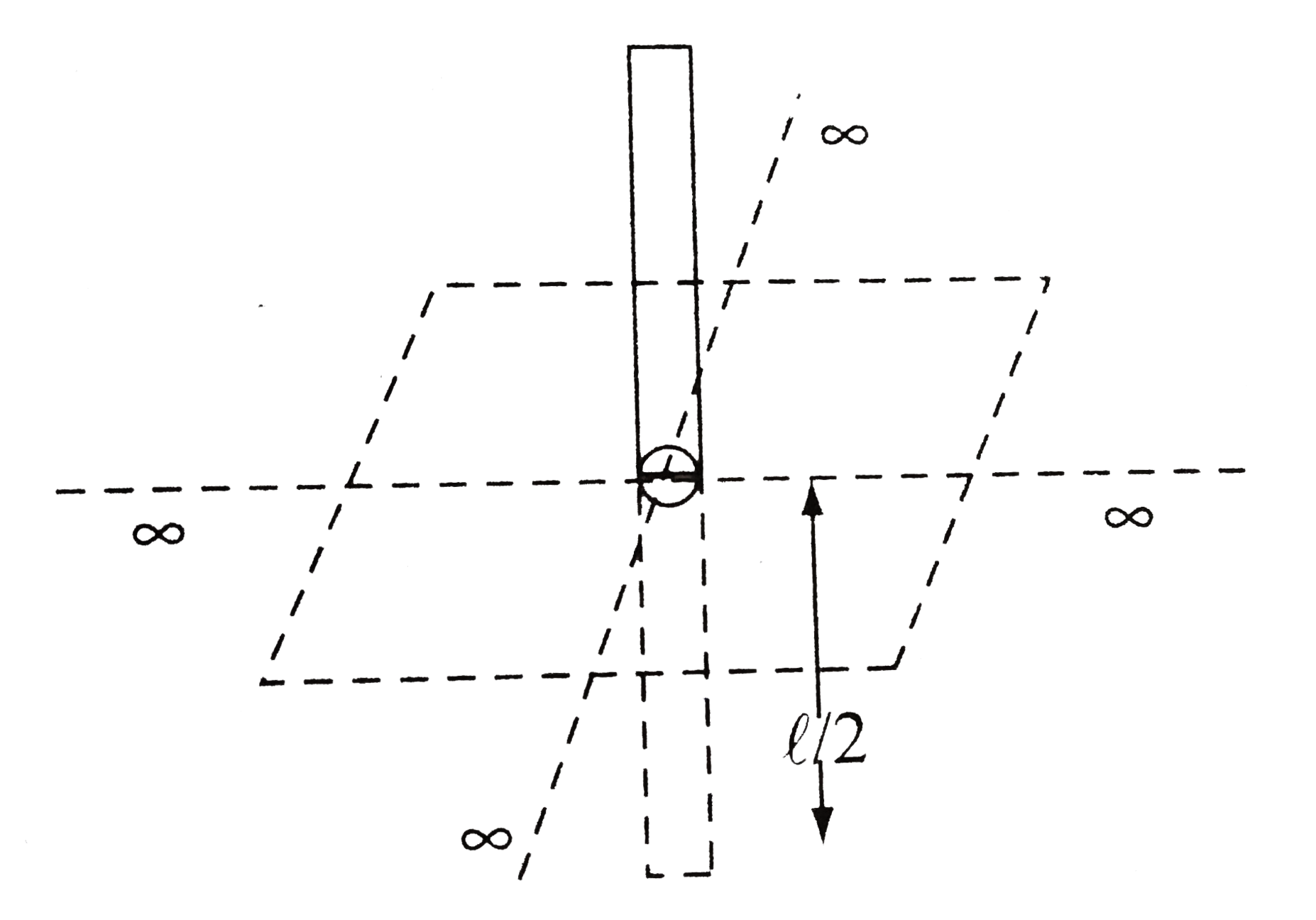 View Solution
View SolutionIf a simple pendulum oscillates with an amplitude of 50 mm and time period of 2 sec, then its maximum velocity is
View SolutionA thin uniform rod of length l is pivoted at its upper end. It is free to swing in a vertical plane. Its time period for oscillation of small amplitude is
View SolutionA uniform rod of mass m and length l is suspended through a light wire of length l and torsional constant k as shown in figure. Find the time perid iof the system makes a. small oscillations in the vertical plane about the suspension point and b. angular oscillations in the horizontal plane about the centre of the rod.
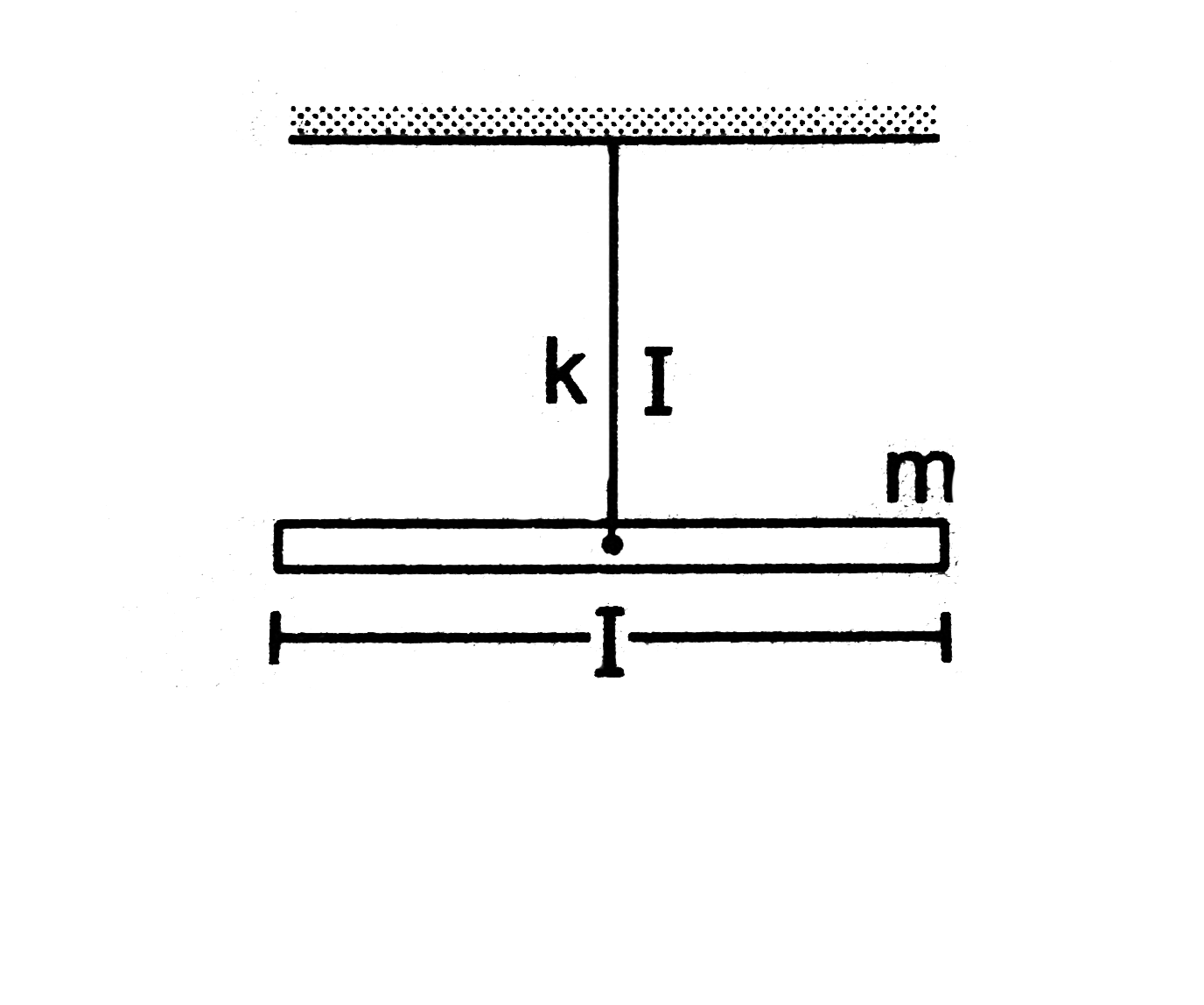 View Solution
View Solution

