In the given pressure - temperature (P-T) diagram, the density of an ideal gas at point A and B are ρ0 and 3ρ02respectively, then the value of X is
3P02
4P0
2P0
3P0
To solve the problem step by step, we will use the ideal gas law and the relationship between pressure, temperature, and density.
1. Understanding the Ideal Gas Law:
The ideal gas law is given by the equation:
PV=nRT
where P is pressure, V is volume, n is the number of moles, R is the universal gas constant, and T is temperature.
2. Expressing Pressure in Terms of Density:
The density ρ of the gas can be expressed as:
ρ=mV
where m is the mass of the gas. Rearranging the ideal gas law, we can express pressure P as:
P=mV⋅RTm=ρ⋅RTM
where M is the molar mass of the gas.
3. Setting Up the Equations for Points A and B:
For point A, we have:
PA=ρ0⋅R⋅TA
For point B, the density is given as 3ρ02, so:
PB=(3ρ02)⋅R⋅TB
4. Relating Pressure at Points A and B:
We can set up a ratio of the pressures at points A and B:
PBPA=(3ρ02)⋅R⋅TBρ0⋅R⋅TA
Simplifying this gives:
PBPA=32⋅TBTA
5. Finding the Value of X:
If we assume that PA=P0 and PB=X, we can write:
XP0=32⋅TBTA
Rearranging gives:
X=P0⋅32⋅TBTA
6. Finalizing the Value of X:
If we assume that TB=TA (which is often the case in these types of problems unless stated otherwise), then:
X=P0⋅32
Therefore, if we consider the standard conditions, we can conclude:
X=3P0
Conclusion:
The value of X is 3P0.
The ideal gas law is given by the equation:
PV=nRT
where P is pressure, V is volume, n is the number of moles, R is the universal gas constant, and T is temperature.
The density ρ of the gas can be expressed as:
ρ=mV
where m is the mass of the gas. Rearranging the ideal gas law, we can express pressure P as:
P=mV⋅RTm=ρ⋅RTM
where M is the molar mass of the gas.
For point A, we have:
PA=ρ0⋅R⋅TA
For point B, the density is given as 3ρ02, so:
PB=(3ρ02)⋅R⋅TB
We can set up a ratio of the pressures at points A and B:
PBPA=(3ρ02)⋅R⋅TBρ0⋅R⋅TA
Simplifying this gives:
PBPA=32⋅TBTA
If we assume that PA=P0 and PB=X, we can write:
XP0=32⋅TBTA
Rearranging gives:
X=P0⋅32⋅TBTA
If we assume that TB=TA (which is often the case in these types of problems unless stated otherwise), then:
X=P0⋅32
Therefore, if we consider the standard conditions, we can conclude:
X=3P0
The value of X is 3P0.
Similar Questions
In the process PV = constant, pressure (P) versus density (ρ) graph of an ideal gas is
View SolutionIf P is the pressure and ρ is the density of a gas, then P and ρ are related as :
View SolutionThe given figure shows the pressure-temperature (P-T) diagram for a fixed mass of an ideal gas undergoing cyclic process ABCA. The corresponding pressure-volume (P-V) graph for the given cyclic process will be
_PHY_E06_003.png)
View SolutionIn figure shown,Pressure versus temperature graph of an ideal gas is as . Density of the gas at point A is ρ0 . Density at point B will be ____
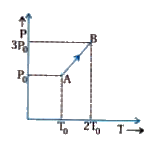
View SolutionPressure versus temperature graph of an ideal gas is shown in figure. Density of the gas at point A is ρ0. Density at B will be
View SolutionThe velocities of sound at same temperature in two monoatomic gases densities ρ1 and ρ2 are v1 and v2 repectively , if ρ1ρ2=4, then the value of v1v2 will be
View SolutionThe density of the given gas at constant pressure and temperature is ρ and its rate of diffusion isr. If density of the gas becone ρ/3 then rate of diffusion becomes
View SolutionThe ednsity (ρ) of an ideal gas varies with temperature T as shown in figure. Then
View Solutionv1andv2 are the velocities of sound at the same temperature in two monoatomic gases of densities ρ1andρ2 respectively. If ρ1ρ2=14 then the ratio of velocities v1andv2 is
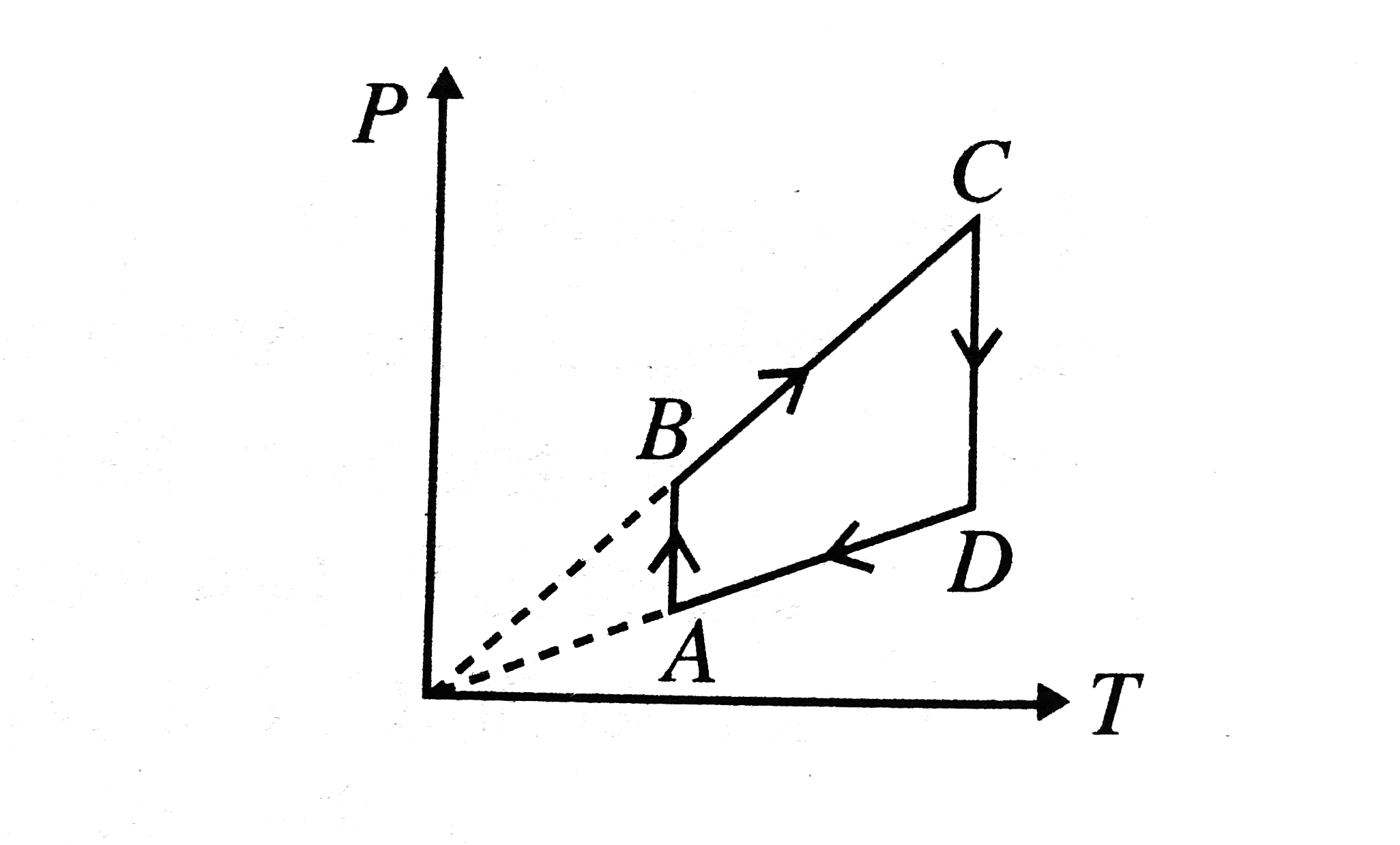
View SolutionPressure versus temperature graph of an ideal gas as shown in Fig.
Corresponding density (ρ) versus volume (V) graph will be
View Solution