A fixed mass of an ideal gas is compressed in such a manner that its pressure and volume can be related as P3V3 = constant. During this process, temperature of the gas is.
increased
Decreased
First increases then decreases
Remains constant
To solve the problem, we need to analyze the relationship given between pressure (P) and volume (V) of an ideal gas, which is expressed as P3V3=constant. We want to determine how the temperature of the gas changes during this process.
1. Understanding the Given Relationship:
We start with the equation:
P3V3=C
where C is a constant.
2. Taking the Cube Root:
To simplify the relationship, we can take the cube root of both sides:
PV=C1/3
Let's denote C1/3 as a new constant C1:
PV=C1
3. Identifying the Type of Process:
The equation PV=C1 resembles the equation for an isothermal process, where the product of pressure and volume remains constant. In an isothermal process for an ideal gas, the temperature (T) remains constant.
4. Using the Ideal Gas Law:
According to the ideal gas law:
PV=nRT
where n is the number of moles and R is the universal gas constant. Since n and R are constants, if PV is constant (as we derived), then T must also be constant.
5. Conclusion:
Since the temperature is constant during the process where PV=C1, we conclude that the temperature of the gas does not change.
Final Answer:
The temperature of the gas remains constant during this process.
We start with the equation:
P3V3=C
where C is a constant.
To simplify the relationship, we can take the cube root of both sides:
PV=C1/3
Let's denote C1/3 as a new constant C1:
PV=C1
The equation PV=C1 resembles the equation for an isothermal process, where the product of pressure and volume remains constant. In an isothermal process for an ideal gas, the temperature (T) remains constant.
According to the ideal gas law:
PV=nRT
where n is the number of moles and R is the universal gas constant. Since n and R are constants, if PV is constant (as we derived), then T must also be constant.
Since the temperature is constant during the process where PV=C1, we conclude that the temperature of the gas does not change.
The temperature of the gas remains constant during this process.
Topper's Solved these Questions
Similar Questions
An ideal gas expands in such a manner that its pressure and volume can be related by equation PV2= constant. During this process, the gas is
View SolutionA gas undergoes a process in which its pressure P and volume V are related as VP = constant. The bulk modulus of gas for this process
View SolutionFor an ideal gas, the heat of reaction at constant pressure and constant volume are related as
View SolutionA gas undergoes a process in which its pressure P and volume V are related as VPn= constant. The bulk modulus of the gas in the process is:
View SolutionThe energy density uV of an ideal gas is related to its pressure P as
View SolutionCan the temperature of a gas increased keeping its pressure and volume constant ?
View SolutionA monoatomic gas undergoes a process in which the pressure (P) and the volume (V) of the gas are related as PV−3=constant. What will be the molar heat capacity of gas for this process?
View SolutionOne mole of an ideal monotomic gas undergoes a linear process from A to B in which its pressure p and its volume V change as shown in figure.
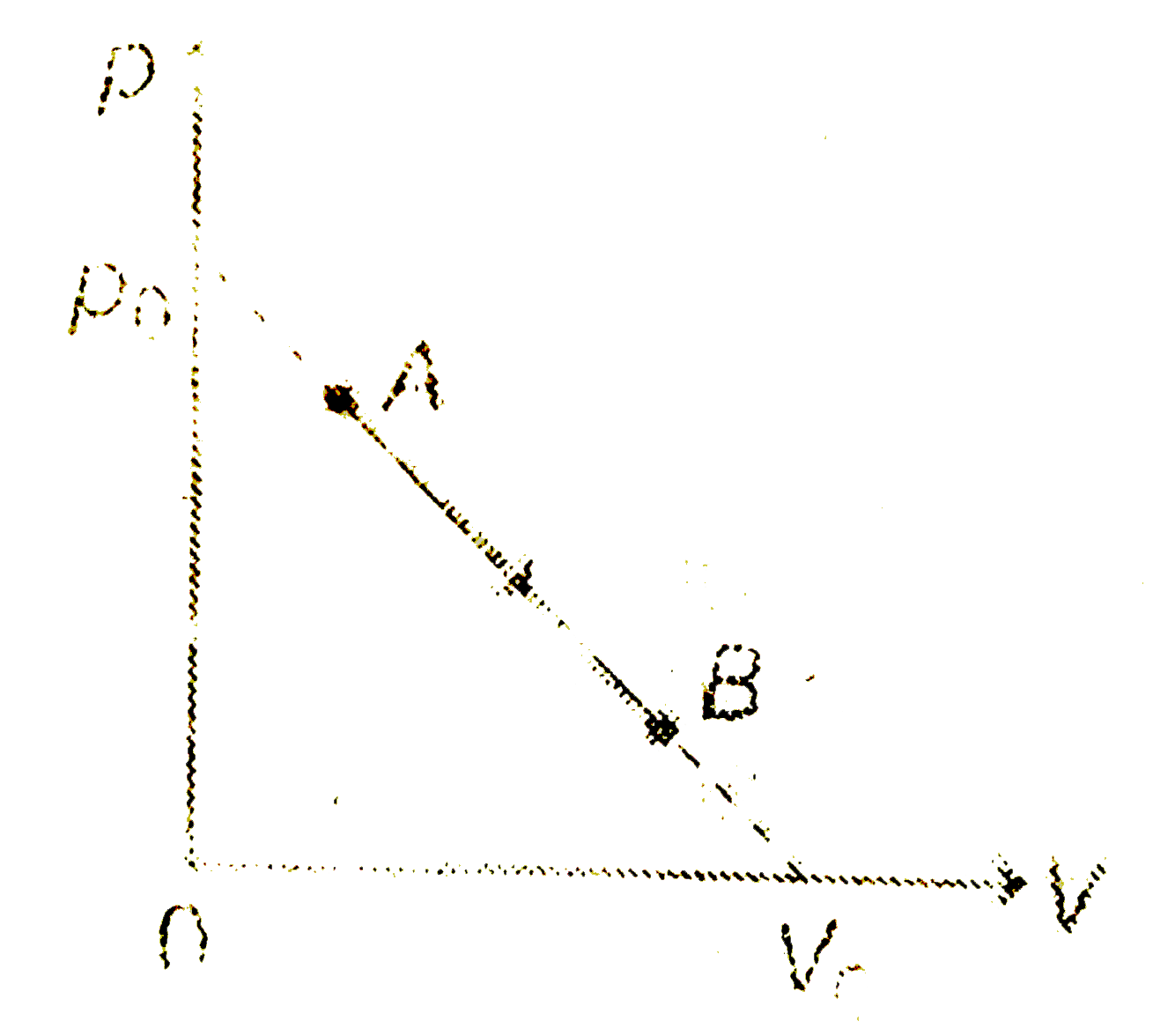
The maximum temperature of the gas during this process is
View SolutionIn a process, temperature and volume of one mole of an ideal monoatomic gas are varied according to the relation VT= K, where K is a constant. In this process the temperataure of the gas is increased by ΔT. The amount of heat absorbed by gas is (R is gas constant).
View SolutionIn a process, temperature and volume of one mole of an ideal monoatomic gas are varied according to the relation VT= K, where K is a constant. In this process the temperataure of the gas is increased by ΔT. The amount of heat absorbed by gas is (R is gas constant).
View Solution
